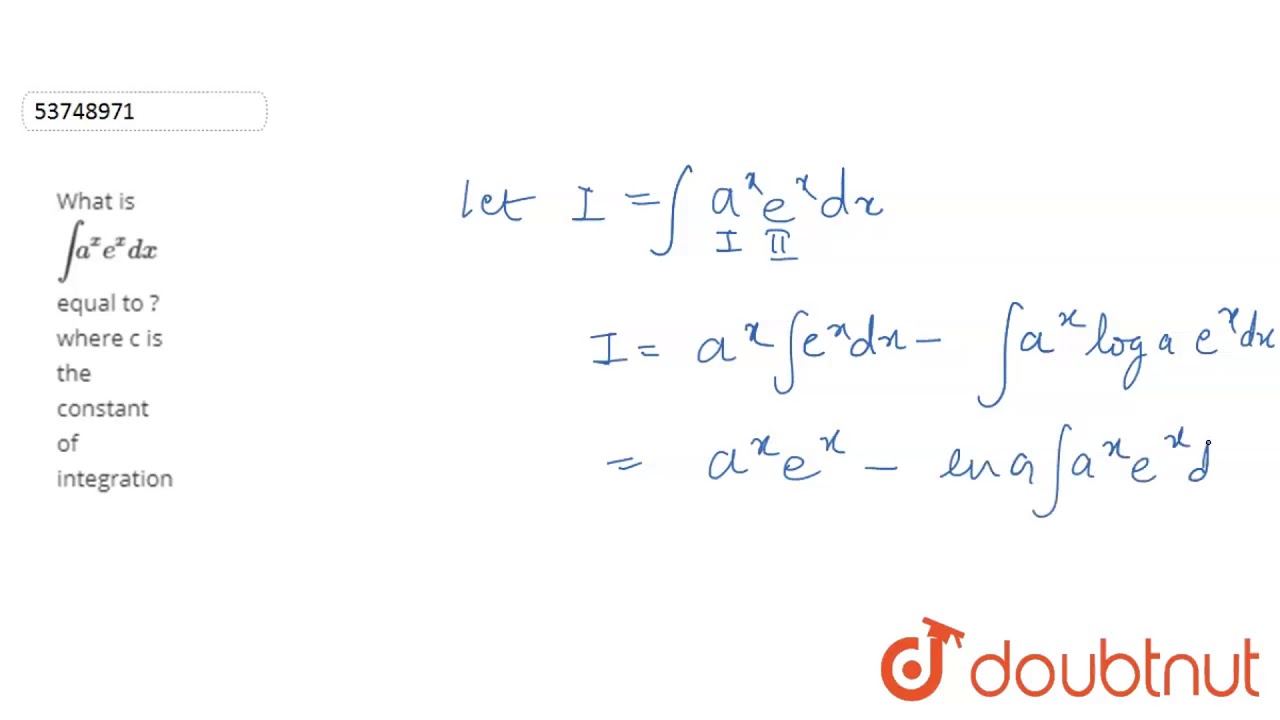
Has a freakin infinity subbed in it. Using repeated applications of integration by parts: This tutorial shows you how to do integration by parts when the integral has limits, (definite integration).
Solved Evaluate The Definite Integral By The Limit Defini
We’ll start with the product rule.
∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application.
By reversing the process in obtaining the derivative of the exponential function, we obtain the remarkable result: The integration by parts equation comes from the product rule for derivatives. ∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1 3 x d x solution. Now, integrate both sides of this.
Then we apply the formula:
However, since one of the limit is infinity, the term. Clear example of integrating xcos2x between 0 and π/4 So integration by parts, i'll do it right over here, if i have the integral and i'll just write this as an indefinite integral but here we wanna take the indefinite integral and then evaluate it at pi and evaluate it at zero, so if i have f of x times g prime of x, dx, this is going to be equal to, and in other videos we prove this, it really just comes straight out of the product rule that you learned in. You will see plenty of examples soon, but first let us see the rule:
Now if i let and , i will have:
This is the currently selected item. V = e x 2. Integration by parts with a definite integral. I'm having a tough time trying to do integration by parts with one of my limits being infinity.
∫ v.dx).dx]b a ∫ a b u v.
Integration by parts with limits. Tabular integration can be used where one function is differentiated until it gets zero, and another function can be integrated simultaneously multiple times. Here, a = lower limit. If we rewrite the integrand as.
We need to use the factor trick on this one.
D x − ∫ ( u ′ ∫ v. There are two related but different operations you have to do for integration by parts when it's between limits: So we have integration by parts uv formula Thus, the formula for the same would be:
Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways.
The limits for integrations by parts can be applied similar to the definite integrals. ∫ (f g)′dx =∫ f ′g +f g′dx ∫ ( f g) ′ d x = ∫ f ′ g + f g ′ d x. Integrating using linear partial fractions. Applying the lower limit 'a', and the upper limit 'b' to integration by parts, we have ∫ b a uv.dx = [u∫ v.dx −∫(u.
It is also possible to get the formula of integration by parts with limits.
It's easier to see how to take the parts. ∫ a b du(dv/ dx)dx=[uv] a b −∫ a b v(du/ dx)dx. ∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx. Tabular integration can also be used where none of the.
This calculus explains how to find the indefinite integral of a 3 product term expression using integration by parts.my website:
For example, we could calculate $∫_0^{\pi} x\cos(x)$ using the solution above as: ( x) d x without the limits of itegration (as we computed previously), and then use ftc ii to evalute the definite integral. First solve it by integration by parts as indefinite integral then use the limits. It is important to read the next section to understand where this comes from.
The usual way to calculate $∫_a^b f(x)\,dx$ is to calculate the indefinite integral first and then apply the limits to the result, and integration by parts is no exception.
Finding an antiderivative for one of the functions (and the derivative of the other, but that's not where your problem lies), and finding the value of the integral between the limits, which we normally (but not necessarily) do by finding a continuous antiderivative. Sometimes integration by parts must be repeated to obtain an answer. `int e^udu=e^u+k` it is remarkable because the integral is the same as the expression we started with. In calculus, definite integrals are being defined as the integral with the upper and lower limits.
(f g)′ =f ′g+f g′ ( f g) ′ = f ′ g + f g ′.
Let u and v be functions of t. X − 1 4 x 2 + c. Where we neglect writing the constant of integration. V' = 2 xe x 2.
U is the function u(x) v is the function v(x) u' is the derivative of the function u(x)
This yields the formula for integration by parts : To do this integral we will need to use integration by parts so let’s derive the integration by parts formula. D x = [ u ∫ v.