Unit 1 extending the number system lesson plan number title. Click here to see a detailed solution to problem 2. X n+1 n+1 + c:
Integral of Exponential Functions Basic Integration
Integrating functions of the form result in the absolute value of the natural log function, as shown in the following rule.
If you have a real number,a, and raise it to a power b we say a^b is a times itself b times.
Indefinite integrals indefinite integrals are antiderivative functions. ∫ f dx + ∫ g dx: `int_0^1sec^2 x\ e^ (tan x)dx`. To apply the rule, simply take the exponent and add 1.
For a complete list of integral functions, please see the list of integrals.
The rules of exponents, also known as the “exponent rules”, are some of the rules on the subject of algebra that we need to be familiar with. Integral exponent means the exponent is a whole number [that is, an integer] note 2: List of integrals of exponential functions the following is a list of integrals of exponential functions. Exponent rules & practice 1.
What is an integral exponent?
Z ax dx= ax ln(a) + c with base e, this becomes: 8 2 could be called 8 to the second power, 8 to the power 2 or simply 8 squared Integral formulas for other logarithmic functions, such as and are also included in the rule. Open image in a new page.
Mastering these basic exponent rules along with basic rules of logarithms (also known as “log rules”) will make your study of algebra very productive and enjoyable.
`int_0^1sec^2x\ e^ (tan x)dx= [e^ (tan x)]_0^1`. Order of operations with parenthesis and exponents algebra worksheets order of operations exponent worksheets. The important laws of exponents are given below: The exponent of a number says how many times to use the number in a multiplication.
A constant (the constant of integration) may be added to the right
As per the power rule of integration, if we integrate x raised to the power n, then; (you can't multiply something by itself negative 3 times! Xn+1 n+ 1 + c; The shaded region represents the integral we need to find.
To multiply when two bases are the same, write the base and add the exponents.
,then we have an integral exponent. Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>: ∫xn dx = (xn+1/n+1) + c. If you can write it with an exponents, you probably can apply the power rule.
We have the integral in the form of ∫ e x (f (x) + f ′ (x)) d x, \displaystyle \int e^x\big(f(x) + f'(x)\big)\, dx, ∫ e x (f (x) + f ′ (x)) d x, where f (x) = sin (x) f(x) = \sin(x) f (x) = sin (x).
You have seen positive exponents. With super, get unlimited access to this resource and over 100,000 other super resources. We can use this rule, for other exponents also. By this rule the above integration of squared term is justified, i.e.∫x2 dx.
The power rule for integrals allows us to find the indefinite (and later the definite) integrals of a variety of functions like polynomials, functions involving roots, and even some rational functions.
Write your answers with positive exponents only. To divide when two bases are the same, write the base and subtract the exponents. A homework assignment which covers rule 1 and 2 only. Click here to see a detailed solution to problem 1.
Let `u=tan x`, then `du=sec^2 x\ dx`.
The power rules for integral exponents. Thank you for being super. Get unlimited access to this and over 100,000 super resources So if b is an integer,.
Integral exponents worksheets with answers.
∫ (f + g) dx: Warm up evaluate each expression for the given values of the variables. Click here to see a detailed solution to problem 3. Assume that all variables represent nonzero real numbers.
Exponents addition subtraction multiplication and division circle the correct answer.
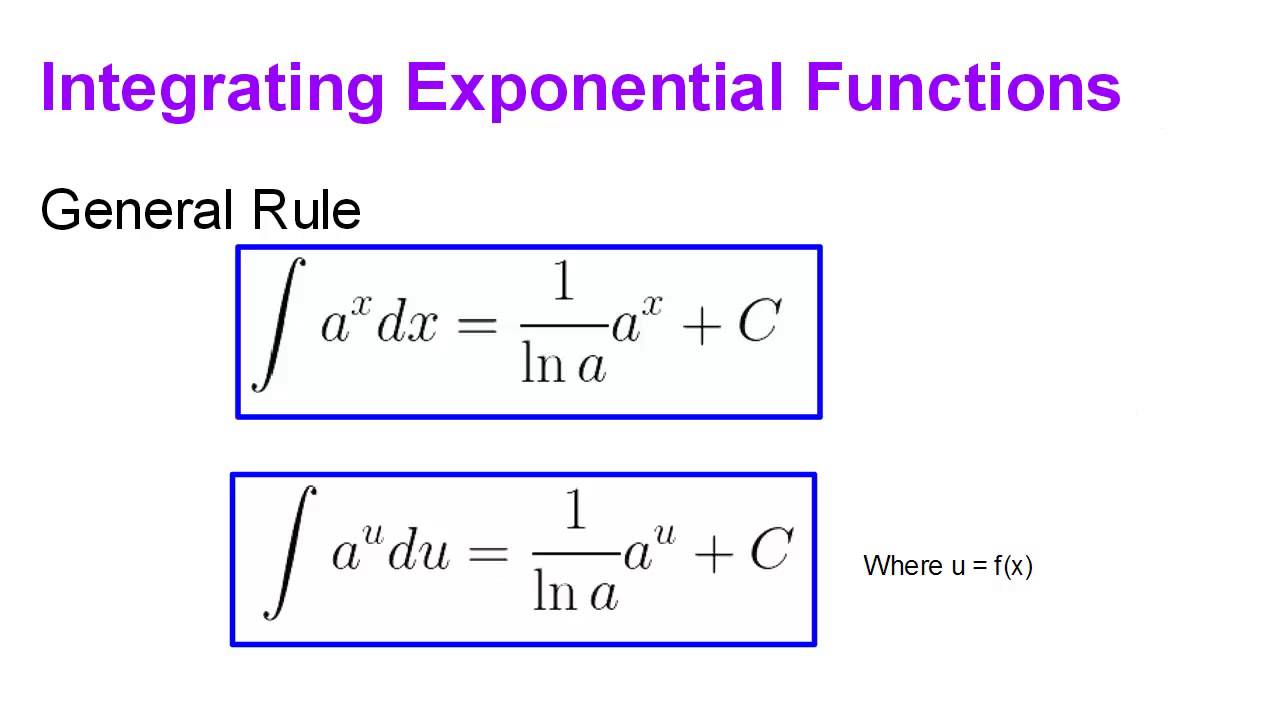
The integral of the exponential function is given by the following formula $\displaystyle \int a^xdx=\frac{a^x}{\ln(a)}$, where $a > 0$ and $a \neq 1$ $e^u$ intermediate steps The integration of exponential functions. E x sin (x) + c. If n6= 1 lnjxj+ c;
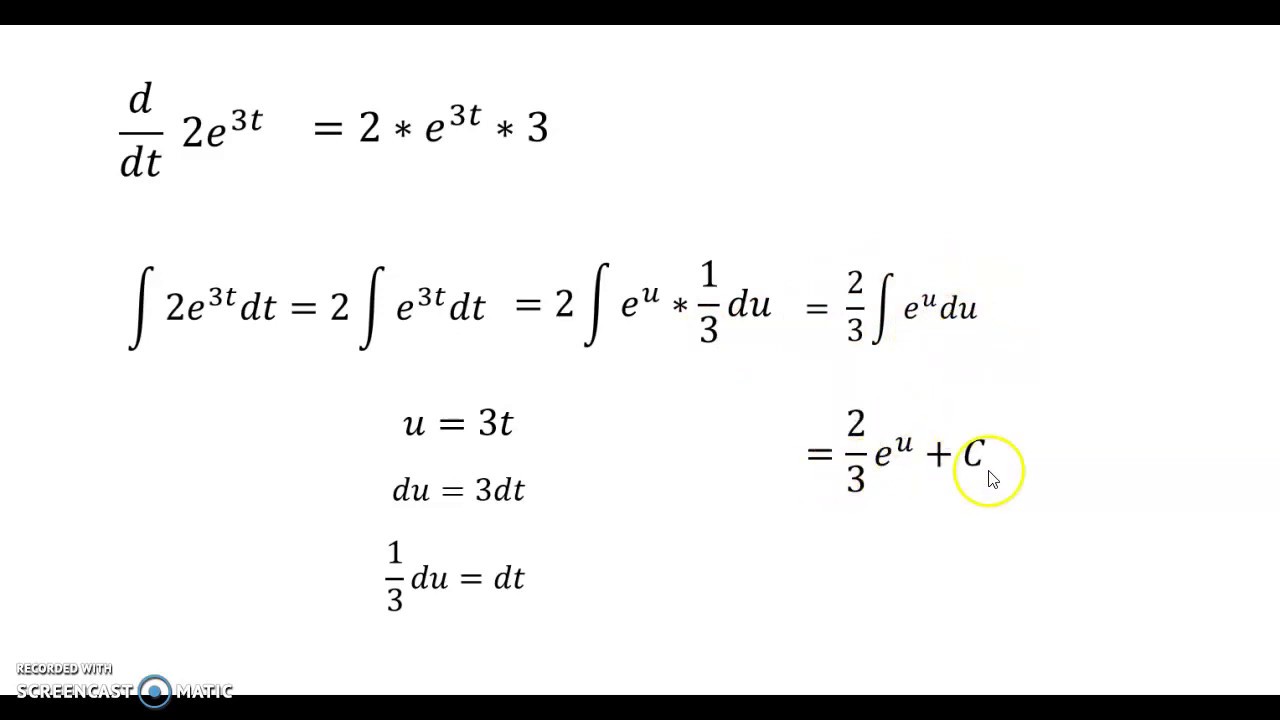
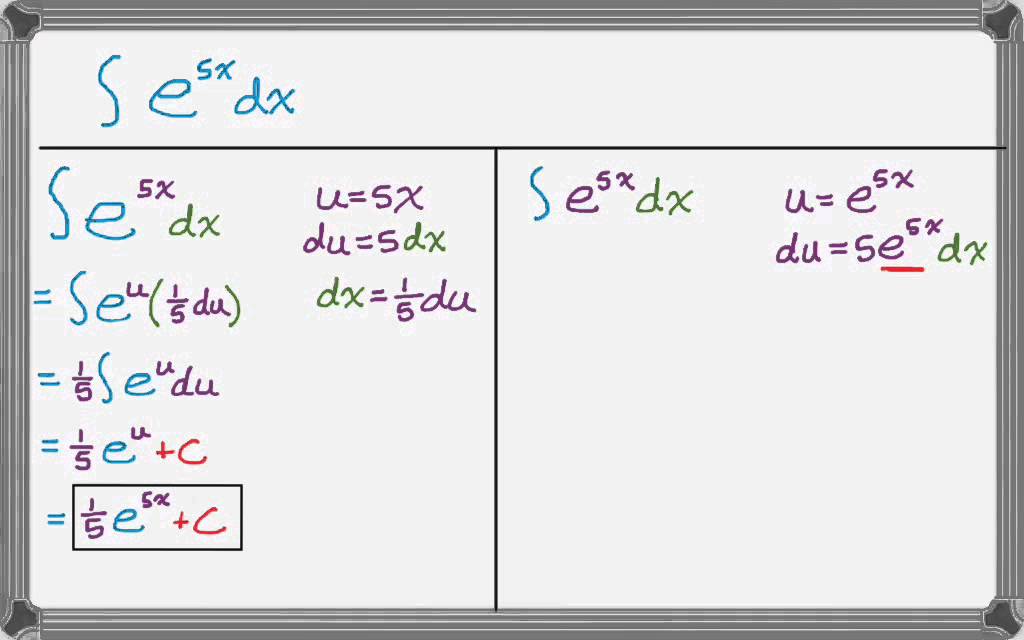
Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z sin(x)dx= cos(x) + c z
∫x3 dx = x(3+1)/ (3+1) = x4/4. In the next example we use the power rules with negative exponents. The above definition only really holds if m is a positive integer, since it doesn't make a lot of sense if m is negative. That is to say aaaaaaaa.aaa b times.
A is the base and b is the exponent.
E^x\sin(x) + c.\ _\square e x sin (x) + c. Power rule (n≠−1) ∫ x n dx: