Then du dx = 2 3 and v = z e3xdx = 1 3 e3x. Then we must nd (iii) du (by di erentiation); ³ ³ ³x e dx fg g fdx x e n x e dxn x n x n x ' 1.
Integration Formulas Trig, Definite Integrals Class 12
1.use integration by parts to nd the following inde nite integrals.
Now, since dv/dx = cos x
Choose u and du use the integration by parts formula for definite integrals u = dv= e did 2. By letting u=f(x)⇒du=f!(x)dx dv=g(x)dx⇒v=g(x) we get the more common formula for integration by parts: We can use the formula again. (uv) = u v + uv.
Apply integration by parts formula 4.
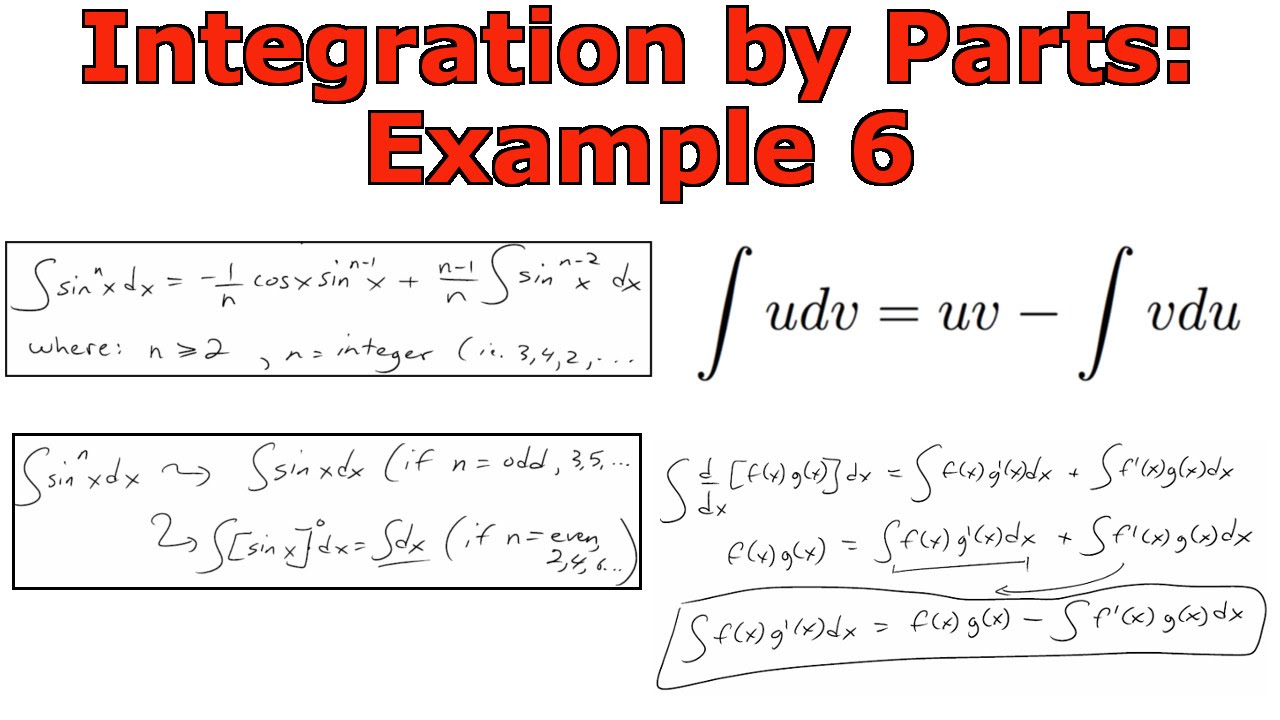
One can integrate all positive integer powers of cos x. A)use trigonometric identities to show that. Most people write the formula for integration by parts as z u dv = uv z v du; The resulting integral is still a product.
The aim in using integration by parts is to obtain a simpler integral (∫ 𝑢𝑢𝑢𝑢𝑢𝑢) than the one started with (∫ 𝑣𝑣𝑣𝑣𝑣𝑣).
As per the formula, we have to consider, dv/dx as one function and u as another function. To use integration by parts, we need to identify (i) u; Knowing which function to call u and which to call dv takes some practice. Integration by parts, calculus, mathematical.
The integral above is defined for positive integer values n.
Again, u = x and dv/dx = cos x. The original integral, but reduces it to a formula that involves the same integral, but with a lower power of x. This time we choose u = 2 3 x and dv dx = e3x. Integration by parts jan 12 learning outcome example find fxé ' dx • recognize when to use integration by parts * recall:
Uv dx = uv − u v dx.
They key is to remember that the second time around, you must use the “same type of substitution” as the first time. ³³x e dx x e n x e dxn x n x n x 1. We can therefore conclude that for any value of n: Integrate and differentiate correct functions 3.
By using the identity sin2 =1 cos2 x,onecanexpresssinm x cosn x as a sum of constant multiples of powers of cosx if m is even.
Because we have an indefinite ∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts: I in n=−2, n≥ 2. & ' ( or & ' ( then, at some point, you have to use integration by parts again.
Formula to convert into an integral involving trig functions.
Integration by parts shortcut formula: Fudv=uv fvdu use the integration by parts formula for indefinite integrals solution 1. See class notes for the details. Sin sin 2 2cos 1 sin.
The standard integration by parts formula is:
∫ 𝑢𝑢𝑢𝑢𝑢𝑢 = 𝑢𝑢𝑢𝑢 − ∫ 𝑣𝑣𝑣𝑣𝑣𝑣. A b− u v dx. This uses a special integration by parts method. Here is a general guide:
Up to 24% cash back this formula for integration by parts often makes it possible to reduce a complicated integral involving a product to.
(2) as an application of the quotient rule integration by parts formula, consider the integral sin(x−1/2) x2 dx. Using repeated applications of integration by parts: Uv = (uv) − u v uv dx = (uv) dx − u v dx uv dx = uv − u v dx the integration by parts formula is: Then sin(x−1/2) x2 dx = 2cos(x−1/2) x1/2 + 2cos(x−1/2) x · 1 2 x−1/2 dx = 2cos(x−1/2) x1/2 −2 cos(x−1/2)· −
Then, using the formula for integration by parts, z x2e3x dx = 1 3 e3x ·x2 − z 1 3 e3x ·2xdx = 1 3 x2e3x − z 2 3 xe3x dx.
C)evaluate inin both cases, where nis either odd or even positive integer. We obtain a quotient rule integration by parts formula: One can use integration by parts to derive a reduction formula for integrals of powers of cosine: Θ θ θ θ − − = −.
Therefore, we have to apply the formula of integration by parts.
At the outset, you can use any one of the following choices: It is dif¿cult enough to train students to use the change of variable method So z x2e3x dx = 1 3 x2e3x − z 2 3 xe3x dx = 1 3 x2e3x − ˆ 2 3 x·. The formula for integration by parts becomes:
Remember, all of the techniques that we talk about are supposed to make integrating easier!
Assign variables the problems that most students encounter with this formula are the substitutions for u, dv, v, and du. Repeat if necessary step 1: 2 22 a sin b a bx x− ⇒= θ cos 1 sin22θθ= − 22 2 a sec b bx a x− ⇒= θ tan sec 122θθ= − 2 22 a tan b a bx x+ ⇒= θ sec 1 tan2 2θθ= + ex. For definite integrals, it becomes:
To derive the formula for integration by parts we just rearrange and integrate the product formula:
It is a product of the functions 2 3 x and e3x. (a)using integration by parts, r f0g= fg r fg0, with f0= sinx(so f= cosx) and g= x2+ 2x, we get z (x2+ 2x)sinxdx= (x2+ 2x)cosx+ z (2x+. Where u = g(x) and dv = f0(x)dx. Integrate by parts twice, and solve for i.
Integration by parts, partial fractions, trigonometric integrals.
We already found the value, du/dx = 1. U inverse trig function (sin ,arccos , 1 xxetc) logarithmic functions (log3 ,ln( 1),xx etc) algebraic functions (xx x3,5,1/, etc) trig functions (sin(5 ),tan( ),xxetc) The formula is given by: Dv u = v u + v u2 du.
Sometimes integration by parts must be repeated to obtain an answer.
We prove this using integration by parts: Integrate both sides and rearrange, to get the integration by parts formula Here, let x is equal to u, so that after differentiation, du/dx = 1, the value we get is a constant value. Let u = x1/2, dv = sin(x−1/2) x3/2 dx, du = 1 2 x−1/2 dx,v= 2cos(x−1/2).
16 x2 49 x2 dx ∫ − 22 x = ⇒ =33sinθ dx dcosθθ 49− x2=−= =4 4sin 4cos 2cos22θ θθ recall xx2=.
Use derivative product rule (uv)0= d dx (uv) = du dx v + dv dx u = u0v + uv0; F e g x f e g nx' , , , 'x n x n. Ncert solutions for class 12 maths chapter 7 exercise 7.11, 7.10, 7.9, 7.8, 7.7, 7.6, 7.5, 7.4, 7.3, 7.2, 7.1 and miscellaneous exercises of integrals in english and free to download in pdf or use online. Integration by parts and partial fractions integration by parts formula :
Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= +
(ii) dv (it must be something we can integrate).