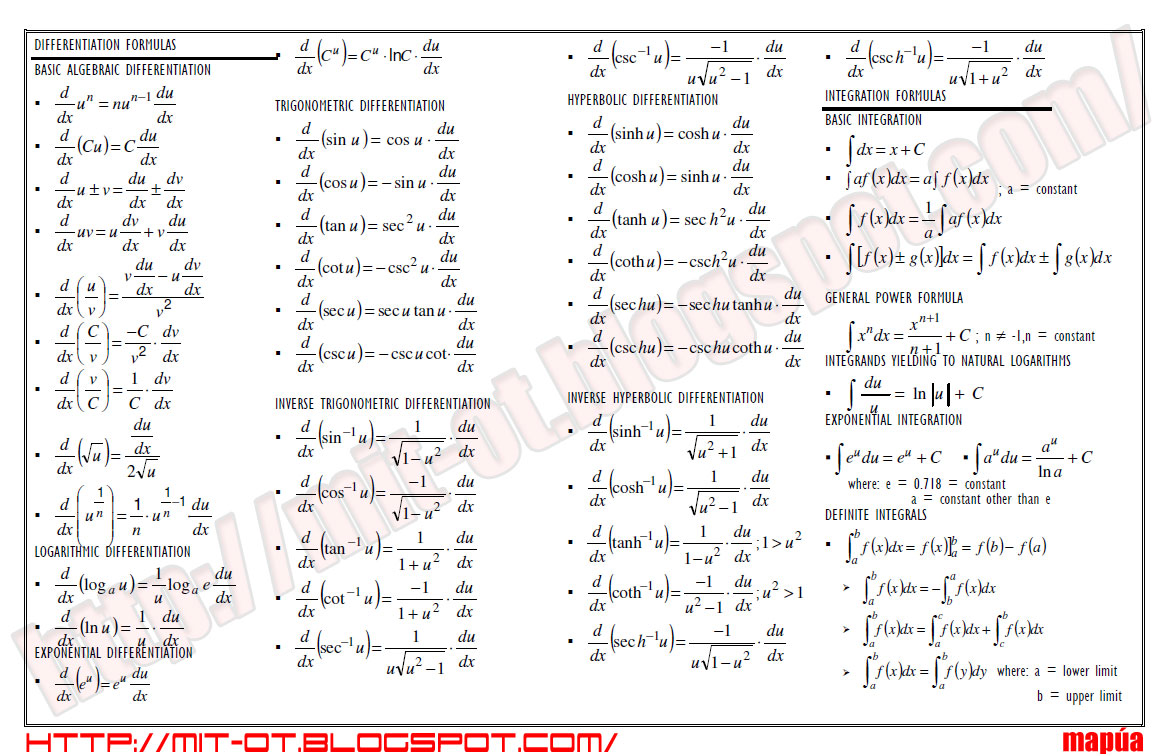
Dx = a x /loga+ c; ∫ ex dx = ex+ c; ∫kf (x)dx = k∫f (x)dx + c.
Differentiation formulas, Calculus, Ap calculus
Some generalized results obtained using the fundamental theorems of integrals are remembered as integration formulas in indefinite integration.
∫ sec x (tan x) dx = sec x + c;
∫ 1 dx = x + c; Integration can be used to find areas, volumes, central points and many useful things. ∫ cos x dx = sin x + c. ∫ (1/x) dx = ln |x| + c;
∫f (x) dx = φ (x) + c ⇔ d dx d d x [φ (x)] = f (x) 2.
Get strong fundamentals of definite integration by using the available definite integration formula cheat sheet. Dx = x (n + 1) / (n + 1) + c; ∫ sec x dx = log|sec x + tan x| + c. With this definite integration formulas list, you can learn definition, properties of definite integrals, summation of series by intergration, and some other important formulas to solve complicated problems.
∫ e x [f(x) + f'(x)].
If we substitute f (x) = t, then f’ (x) dx = dt. You will very well know the concepts by referring to the antiderivative formulas provided. ∫ xn dx = ((xn+1)/(n+1))+c ; 2 1 sin ( ) 1 cos(2 )x 2 sin tan cos x x x 1 sec cos x x cos( ) cos( ) x x 22sin ( ) cos ( ) 1xx 2 1 cos ( ) 1 cos(2 )x 2 cos cot sin x x x 1 csc sin x x sin( ) sin( ) x x 22tan ( ) 1 sec ( )x x geometry fomulas:
∫ cot x dx = log|sin x| + c.
∫ 1.dx = x + c; Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + ∫(c) = x+c ∫ ( c) = x + c ( where c is a constant) ∫(cx) = cx2 2 +c ∫ ( c x) = c x 2 2 + c ( where c is a constant) ∫(xn) = xn+1 n+1 ∫ ( x n) = x n + 1 n + 1. ∫ sec x (tan x) dx = sec x + c;
Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z
∫(ax) = ax loga +c ∫ ( a x) = a x l o g a + c. ∫ sec2 dx = tan x + c; List of indefinite integral formulas the main motto behind providing the indefinite integral formulae here is to simplify your work while doing complex problems involving integrals. For a complete list of antiderivative functions, see lists of integrals.
∫ tan x dx = log|sec x| + c.
∫ a dx = ax+ c; Contents 1 integrals involving only sine 2 integrands involving only. Generally, if the function is any trigonometric function, and is its derivative, in all formulas the constant a is assumed to be nonzero, and c denotes the constant of integration. ∫ 1 dx = x + c;
A s2 1 area of a triangle:
The basic integral formulas are given below: Basic forms z xndx = 1 n+ 1 xn+1(1) z 1 x dx= lnjxj (2) z udv= uv z vdu (3) z 1 ax+ b dx= 1 a lnjax+ bj (4) integrals of rational functions z 1 (x+ a)2. ∫ sec2x dx = tan x + c. Integration formulas can be applied for the integration of various different functions such as algebraic expressions, trigonometric functions, inverse trigonometric functions, logarithmic and exponential functions.
The list of integral formulas are given below:
∫(1 x) = ln|x|+c ∫ ( 1 x) = l n | x | + c. Dx = e x + c; ∫ cos x dx = sin x + c; Table of integrals basic forms (1)!xndx= 1 n+1 xn+1 (2) 1 x!dx=lnx (3)!udv=uv!vdu (4) u(x)v!(x)dx=u(x)v(x)#v(x)u!(x)dx rational functions (5) 1 ax+b!dx= 1 a ln(ax+b) (6) 1 (x+a)2!dx= 1 x+a (7)!(x+a)ndx=(x+a)n a 1+n + x 1+n #$ % &', n!1 (8)!x(x+a)ndx= (x+a)1+n(nx+xa) (n+2)(n+1) (9) dx!1+x2 =tan1x (10) dx!a2+x2 = 1 a tan1(x/a) (11) xdx!a2+x2.
But it is easiest to start with finding the area under the curve of a function like this:
∫ x n dx = ((x n+1)/(n+1))+c ; ∫ ax dx = (ax/ln a) + c ; (i) when you find integral ∫g (x) dx then it will not contain an arbitrary constant. Dx= ln( 1 x+ a (5) z (x+ a)ndx= (x+ a)n+1.
∫(ex) = ex +c ∫ ( e x) = e x + c.
Dx = log|x| + c; ∫ xe x dx is of the form ∫ f(x).g(x). If f (x), g (x) are two functions of a variable x and k is a constant, then. 6 rows some generalised results obtained using the fundamental theorems of integrals are remembered as.
Know more about these integrals class 12 formulas in pdf list.
∫ sec 2 x dx = tan x + c; ∫ (1/x) dx = ln |x| + c ∫(logax) = 1 xlna +c ∫ ( l o g a x) = 1 x l n a + c. ∫ cos x dx = sin x + c;
∫ ( d d x ( f ( x)) ∫ ( g ( x)) d x) d x.
Integral formulas of trigonometric functions: Integrals of some special function s. Integration is a fundamental operation of calculus. You can use the simple formulas for indefinite integral and apply them in.
The integration formula while using partial integration is given as: