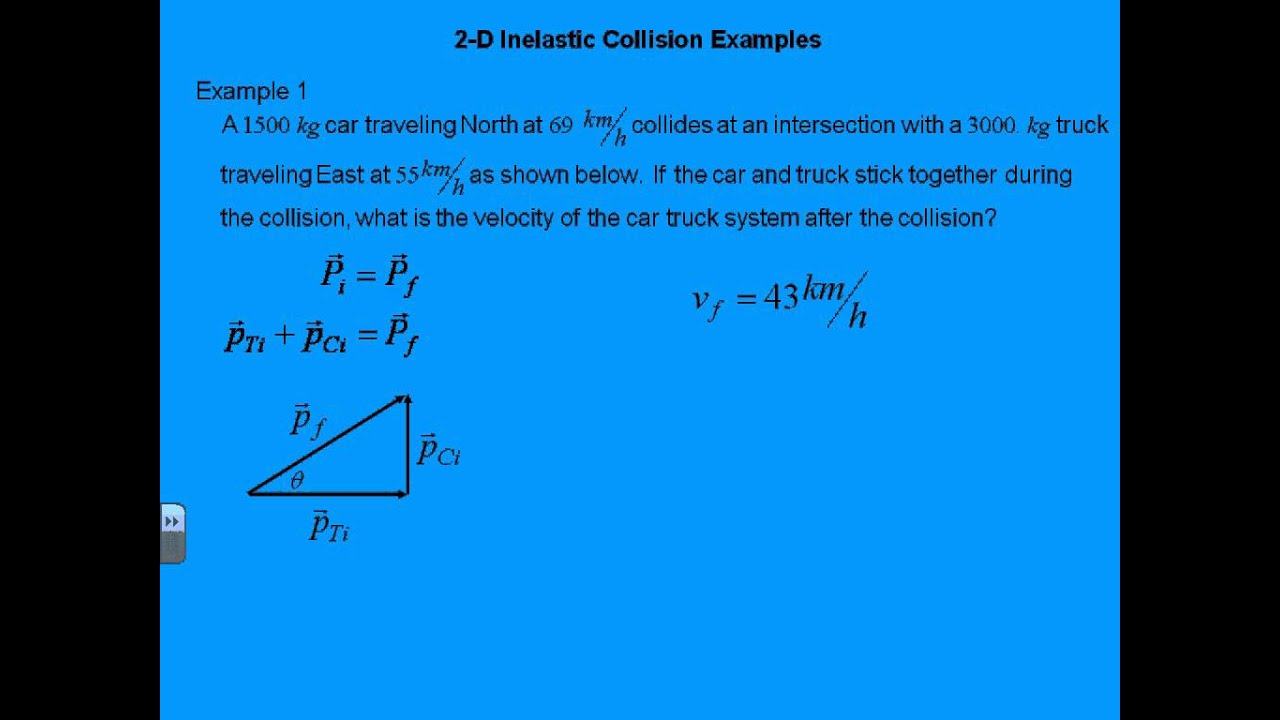
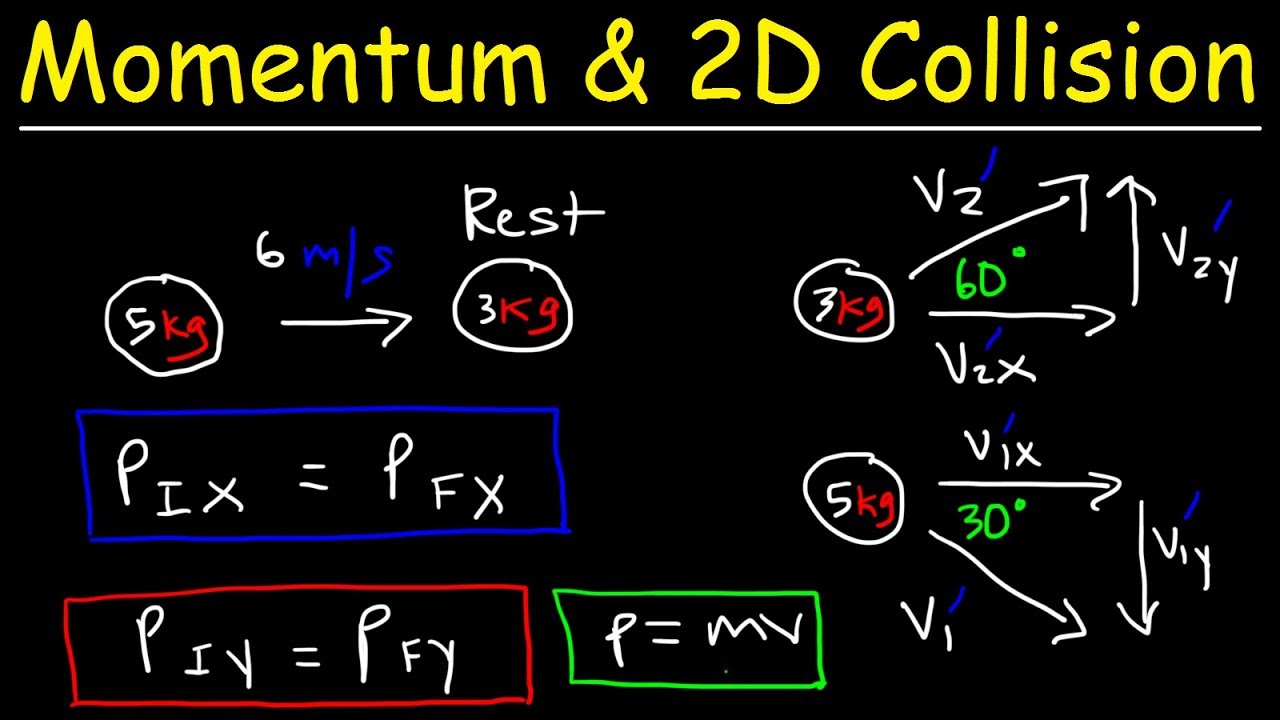
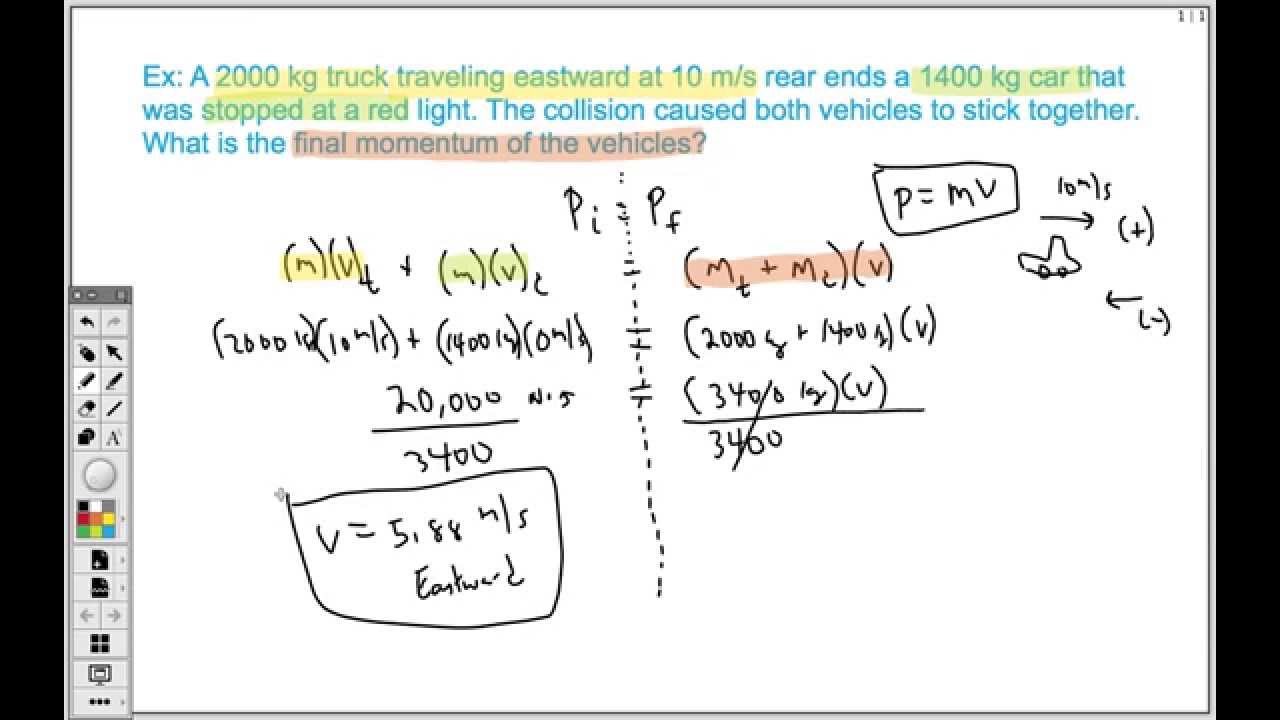
An inelastic collision is commonly defined as a collision in which linear momentum is conserved, but kinetic energy is not conserved. P~ t ˘m1 ~v1 ¯m2v~2 ¯¢¢¢¯mnv~n (2.2) when the sum of external forces acting on a system is zero, momentum is conserved. Therefore, the momentum p f after inelastic collision becomes m 1 v + m 2 v or v(m 1 +m 2) so, the equation of conservation of momentum for inelastic collision is, m 1 u 1 + m 2 +u 2 = v(m 1 +m 2) the formula for final velocity after inelastic.
Angular Momentum in an Inelastic Collision Physics Forums
Mass and velocity of a.
This common nal velocity can be found from the momentum conservation equation (1):
After the boys collide, their combined velocity is 3.20 m/s. M 1 v 1 = (m 1 +m 2)v 2 where: Understanding elastic and inelastic collisions. Momentum is conserved, m1→v i1 + m2→v i2 = m1→v f1 + m2→v f2 = (m1 + m2)→v f m 1 v → i 1 + m 2 v → i 2 = m 1 v → f 1 + m 2 v → f 2 = ( m 1 + m 2) v → f.
~p net = m 1~v 1 + m 2~v 2 = m 1~v 0 + m 2~v0 = (m 1 + m 2)~v0;
Any objects that collide in this way will reduce the total kinetic energy (and total velocity) by this ratio. Because momentum is a vector equation, there is only one momentum conservation equation. Totally inelastic collisions in a totally inelastic collision, the two colliding bodies stick together and move at the same velocity ~v0 1 = ~v02= ~v0after the collision. (a) two objects of equal mass initially head directly toward one another at the same speed.
Kf / ki = m1 / ( m1 + m2 ) some basic mathematical analysis will allow you to look at the expression m1 / ( m1 + m2) and see that for any objects with mass, the denominator will be larger than the numerator.
The total system momentum is. Where mass of body 1 = m 1. The inelastic collision equation is: (16) hence ~v0 1 = ~v 0 2 = ~v 0 = m 1 m 1 + m 2 ~v 1 + m 2.
Fill in the start conditions:
All quantities are in si units. That is, the total initial momentum of the system p~i is equal to the total final momentum p~f: The final velocity can be found for the two boys by rearranging the formula: Mass of the stationary object, in kg v 2:
The formula for inelastic collision:
Velocity of the moving object, in m/s m 2: Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in. The momentum and energy of the particle at rest are rr p =0 em=. Mass of the moving object, in kg v 1:
The momentum of the moving particle is m 22 45 4 1 4 3 1 5 mv pm v == = − ⎛⎞ −⎜⎟ ⎝⎠ m, and its energy is () m m 43 5 45 3 p m em v == =, for the composite particle, the conservation of energy implies that cm r 8 3 e =+=ee m, while the conservation of momentum implies that cm 4 3 pp==m.
Solution to example 1 let p1 be the momentum of the two balls before collision. After the collision, what is the speed and direction of each ball? The calc will provide the unknown mass or velociy of b. We know that the formula for momentum is given as formula:
Momentum of collided body = (m 1 + m 1) × v.
Up to 10% cash back example question #1 : The inelastic collision formula is articulated as. Thus, it is possible to equate momentum in the start and final states of a system and thus calculate an unknown. (b) the objects stick together (a.
Momentum is conserved, but internal kinetic energy is not conserved.
Similarly, there will only be one energy conservation equation. Mass of body 2 = m 2 the initial velocity of body 1 = u 1 the initial velocity of body 2 = u 2 Then fill in either the mass of b or the final velocity of a+b. This formula is used to calculate the final velocity of the objects after the collision in one dimension if the collision is a perfectly inelastic collision.
The first ball has mass 0.54kg and is traveling 7.1m/s to the right.
The total momentum p~t of a system of n discrete parts can be expressed as the sum of each of the parts: V = (m 1 v 1 +m 2 v 2)/(m 1 +m 2) where, v= final velocity Since the collision is inelastic, we know that. The crash in which kinetic energy of the system is not conserved but the momentum is conserved, then that collision is termed as inelastic collision.
The general equation for conservation of linear momentum for a system of particles is:
Velocity of the stationary object after collision, in m/s Pb = mass × velocity = 0.7 × 0 = 0 kg.m/s p1 = pa + pb = 1 kg.m/s after collision the two balls make one If the collision is considered perfectly inelastic, the equation due to the conservation of momentum can be written as {eq}m_1v_1 + m_2v_2 = (m_1 + m_2)v' {/eq}. Putting the value gives, m 1 u 1 + m 2 u 2 = (m 1 + m2) v.
M1, m2 ,., mn is.
In any closed system, momentum is conserved. From, law of conservation of momentum we get to know that, p initial = p final But if the collision is inelastic, both objects move with one final velocity v in the same direction.