Convert the equation into general form. Here a=4, b=2 and r=6. X 2 + y 2 = r 2.
Equation of a circle 1 YouTube
The required equation is x 2 + y 2 = 4 2.
Y ≈ 17,813.006 km y ≈ 17,813.006 km.
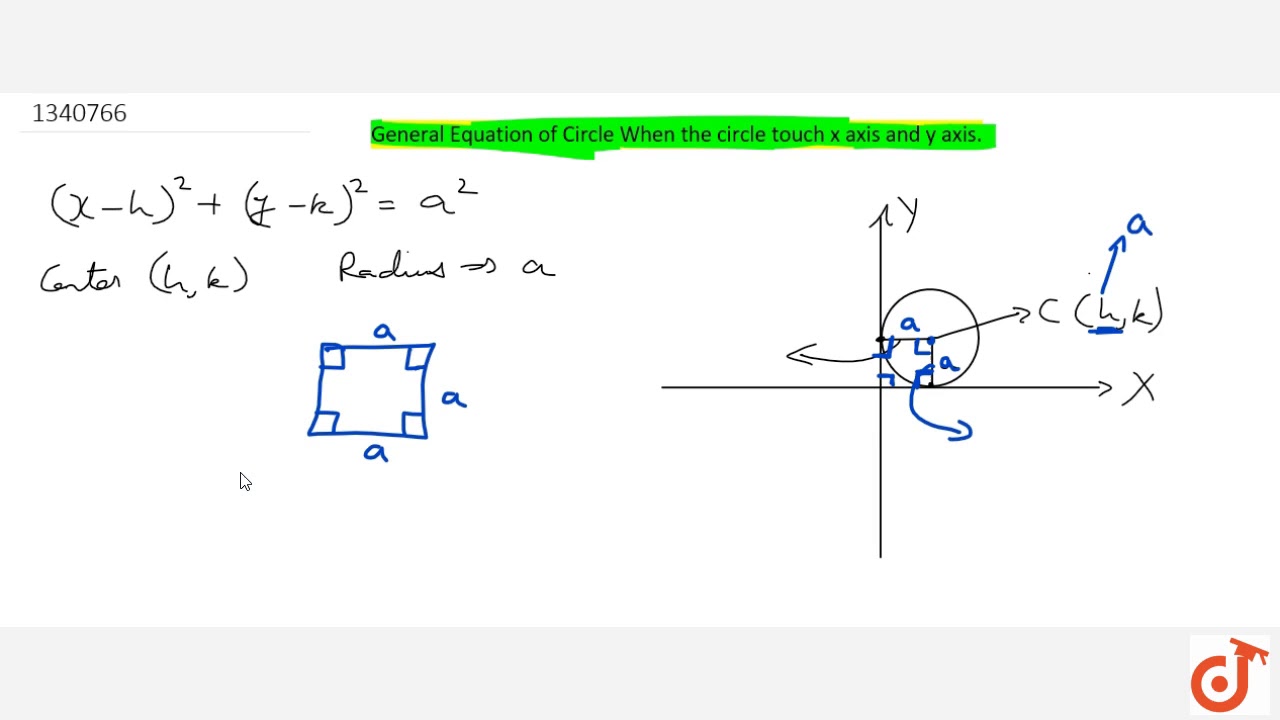
This is called the general equation of a circle. Given the general equation of circle find the coordinates for center of the circle. General form of the equation of a circle : X 2 + y 2 + 2gx + 2fy + c = 0.
Circles outcome 4 equations of a circle the.
And we end up with this: First group the similar variables together and move constant to the right side of the equation. (x + 4) 2 + y 2 = 49. Therefore the equation of circle is= (x − 4)2 + (y − 2)2 = 36.
Sample problem 2 find the center and radius of a circle given by the equation (x +6)2 +(y +3)2 = 4 solution:
The radius (r) hasn’t been given. We know that the standard equation is. X 2 + 2(x)(4) + 4 2 + y 2 = 49. Find the equation of the circle, whose centre is at the origin and radius is equal to 9 units.
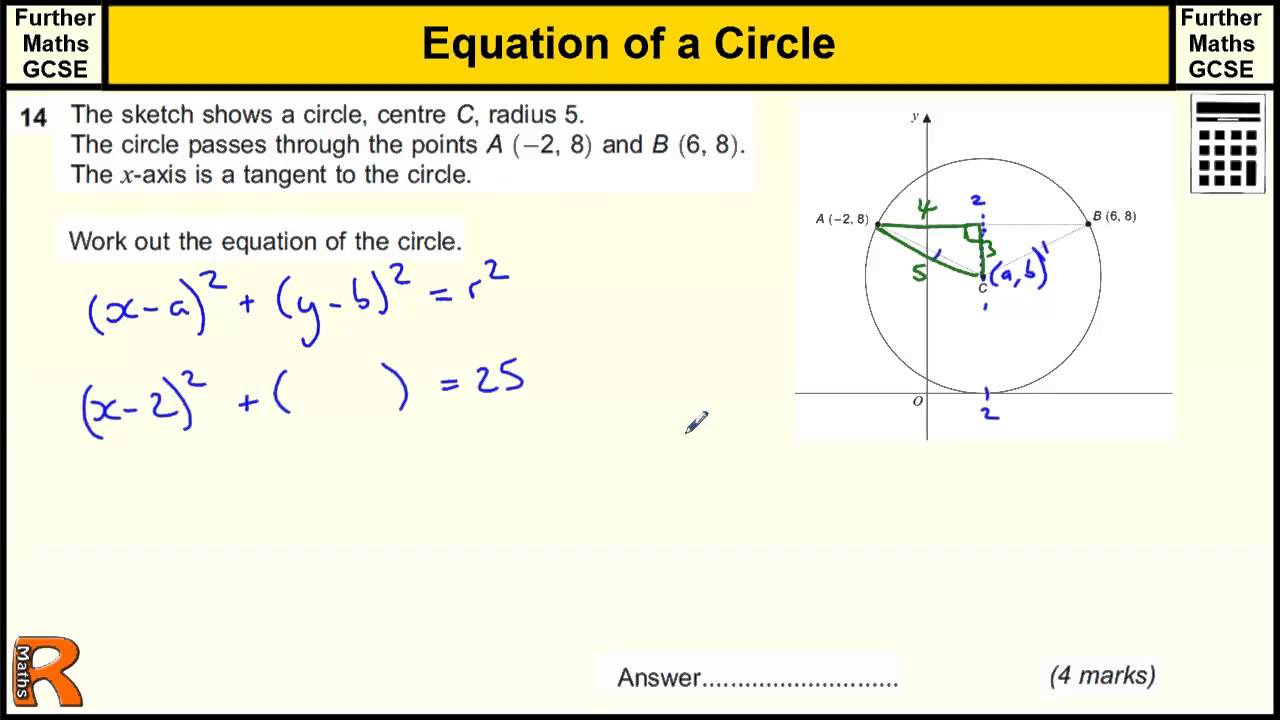
Find the centre and radius of the circle with the given equation of a circle.
Solution a slight twist in the previous example. Find the diameter form of the circle, drawn on the intercept made by the line 2x + 3y = 6 between the coordinates axes as diameter. So when you see something like that think hmm. We rewrite the equation as, dividing whole equation by 3.
The line 2x + 3y = 6 meets x and y axes at a (3, 0) and b (0, 2) respectively.
Circle equations harder example khan academy. Now solve for and by completing the square. Note that you have to add same value on both side of the equation. Ad over 27,000 video lessons and other resources, you're guaranteed to find what you need.
X2 − 2x + 1 + y2 − 4y + 4 = 9.
X 2 + y 2 + 2gx + 2fy + c = 0. X2 + y2 − 2x − 4y − 4 = 0. Write the following equation of a circle in general form. Given general equation of circle.
Converting an equation of a circle from general to standard form key stage 3.
X2 + y2 − 2x − 4y + 1 + 4 − 9 = 0. As given in the question, radius = 4. We know that the equation of the circle described on the line segment joining ( x 1, y 1) and ( x 2, y 2) as a. But it is known that the circle passes through the point (3, 2), which means its coordinates.
This is a ks3 lesson on converting an equation of a circle from general form to standard form.
Subtract 49 from each side. Radius = 2 university of minnesota general equation of a circle 9 rows for example, the center of the circle is (1, 1) and the radius is 2 units then the general. (x−1)2 + (y−2)2 = 32.
Equation of a circle in standard form formula practice problems and pictures how to express with given radius.
Find the centre and radius? Equation of the circle in general form : Given parameters are center (a, b) = (4, 5); X 2 + y 2 + 8x + 16 = 49.
You know your c c value, your hypotenuse, is 20,428 km 20,428 k m.
Given centre is at origin that is (0,0) and radius is 9 units. It is for students from year 8 who are preparing for gcse. General form of the equation of a circle examples. Radius = 2 university of minnesota general equation of a circle
Example 2 find the equation of the circle whose centre is at the origin and which passes through the point (3, 2).
X 2 + 8x + 16 + y 2 = 49. Equation of a circle mathematics level revision Represent this as a circle equation ? To find the centre and radius of the circle, we first need to transform the equation from general form to standard form.
The circle equation general form is expressed as:
Equation of a circle with centre (h, k) and radius r : It is a circle equation, but in disguise!