Equations of motion for pure rotation (section 17.4) ∑fn = m (ag)n = m rg ω2 ∑ft = m (ag)t = m rg α ∑mg = ig α since the body experiences an angular acceleration, its inertia creates a moment of magnitude igαequal to the moment of the external forces about point g. I ω ˙ + ω × = m. In physics, equations of motion are equations that describe the behavior of a physical system in terms of its motion as a function of time.
PPT Chapter 9 Rotational Motion PowerPoint Presentation
The rotational equations of motion for body 2 may be written as euler's equations of motion in.
T = δ l/δ t, or torque is the rate of change of angular momentum.
It only describes motion—it does not include any forces or masses that may affect rotation (these are part of dynamics). This has to do with linear and rotational versions of deriv. For a body with uniform mass distribution. Ω=2π/t=2πf where t is the period of the motion and f is the frequency angular acceleration (centripetal acceleration);
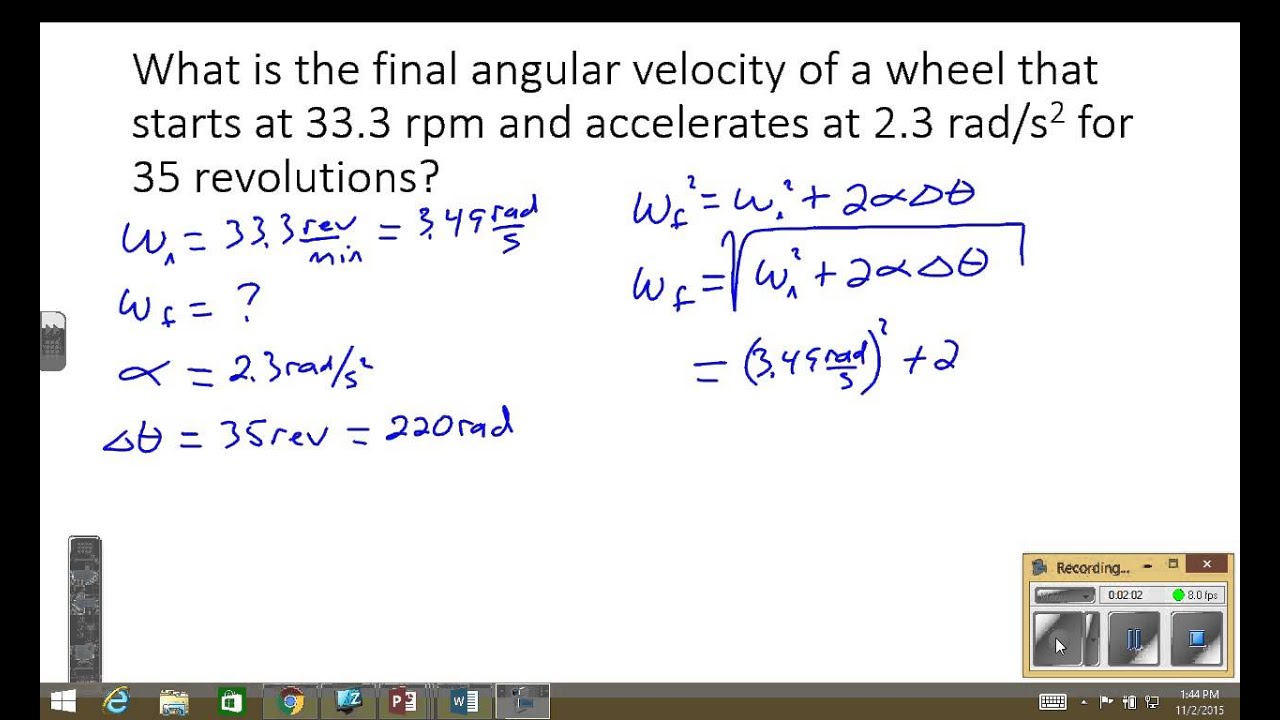
V² = v 0 2 + 2ax
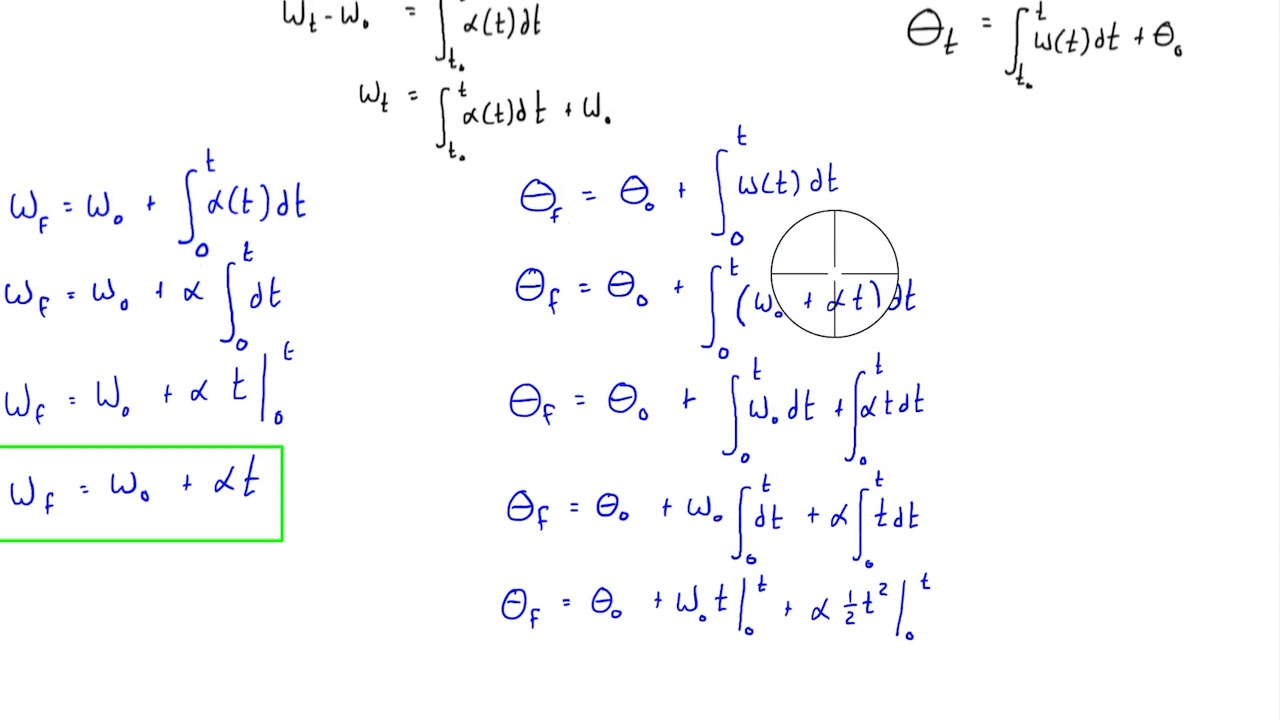
Solving this equation for x yields. Consider an object that starts rotating about a fixed axis at time \(t = 0\) with an initial angular velocity \({\omega _0}\). Arrow_back browse course material library_books « previous | next » session overview. Torque, t, is the tendency of a force to rotate an object about some axis • torque is a vector.
Finding equations of motion for rigid body rotation.
More specifically, the equations of motion describe the behavior of a physical system as a set of mathematical functions in terms of dynamic variables. We have already learned in the kinematics equations of linear or translational motion with uniform acceleration. Equations of motion for rotational motion. S = ut + 1/2 at^2 3.
Recall the kinematics equation for linear motion:
V = v 0 + a t v = v 0 + a t (constant a). Change in linear velocity with respect to time 4 rows θ= x r θ = x r. Displacement “s” is analogous to angle of rotation ( θ ), acceleration (a) is analogous to angular acceleration ( α ).
V=2πr/time where r is the radius of the motion path and t is the period of the motion angularvelocity;
After some time, \(t = t\) let its angular velocity be \(\omega\). I = m 1 r 12 + m 2 r 22 + m 3 r 32 + = ∑ i = 1 n m i r i 2. The three equation of motion was, v = v 0 + at. Let it move with a constant angular acceleration \(\alpha \) throughout the rotation and starting from an angular displacement.
Week 7 emphasizes finding the equations of motion of rotating and translating rigid bodies.
Change in linear displacement with respect to time: Translation rotation x dx v dt d = dt dv a dt d = dt f (?) m (?) f = ma (?) = (?) ke = (1/2) m v2 ke = (1/2) (?) 2 the rotational analogue of force is torque. Ω 22 = ω 12 + 2αθ. For a point of mass, angular momentum can be expressed as the product of linear momentum and the radius ( r):
These variables are usually spatial coordinates and time, but may include momentum.
V = u + at 2. Ω ¯ = ω 0 + ω 2 and v ¯ = v 0 + v 2. Change in angular displacement with respect to time: Analogous to newton's law (f = δ( mv)/δ t) there is a rotational counterpart for rotational motion:
Now let us see the derivation of the first equation.
Kinematic equations of rotational motion. Before using this equation, we must. V = u + at. Copyright © 2012 pearson education inc.
V^2 = u^2 + 2as (where) v = final velocity , u = initial velocity, s = displacement, t = time and a = acceleration.
Its magnitude is t = r fsin f = f d. These are equations that you will need to be able to derive and/or have memorized for your test. We introduce more complex problems and consider systems with more than one degree of freedom. The kinematics of rotational motion describes the relationships between the angle of rotation, angular velocity, angular acceleration, and time.
Ω ¯ = ω 0 + ω 2 and v ¯ = v 0 + v 2.
For every quantity in linear (1d translational) motion, there is corresponding quantity in rotational motion: Ω 2 = ω 1 + αt. Now, in the case of rotational motion, velocity (v) corresponds to the angular velocity ( ω ). {\displaystyle \mathbf {i} {\dot {\boldsymbol {\omega }}}+{\boldsymbol {\omega.
Θ = ω 1 t + 1 2 αt 2.
Size 12 {α} {} are constant. Regarding this, what is the formula of rotational motion? Or where ω is the angular velovity, r is the radius and v is the tangential velocity centripetal force; X = x 0 + v 0 t + (1/2) at².