A quotient rule is stated as the ratio of the quantity of the denominator times the derivative of the numerator function minus the numerator times the derivative of the denominator function to the square of the denominator function. Quotient and product rule formula: Use similar reasoning to obtain the following integratio quotient rule.
quotient rule integration DriverLayer Search Engine
Letting u = g (x) and v = f (x) and observing that du = g (x) d x and dv = f (x) d x,we obtain a quotient rule integration by parts formula:
Then f / g is differentiable at x and.
Tabular integration is a short method for integration to solve the integral problem quickly, instead of using the lengthy and tedious process of integration by parts traditional method. Let f ( x ) be a real valued function defined on an open interval ( a, b ) and let c ( a, b ). Then, f ( x ) is said to be differentiable or derivative at x = c if and only if. X2let u = x 1/2, dv = sin (x −1/2) dx, du = 1 x−1/2 d x, x 3/2 2 v = 2 cos (x−1/2).then sin (x −1/2) 2 cos.
R kf(x)dx = k r f(x)dx.
It follows from the limit definition of derivative and can be given by. The integration by parts formula is obtained from which differentiation rule? F ′ ( x) = lim δ x → 0 f ( x + δ x) − f ( x) δ x. (2) as an application of the quotient rule integration by parts formula, consider the integral sin(x−1/2) x2 dx.
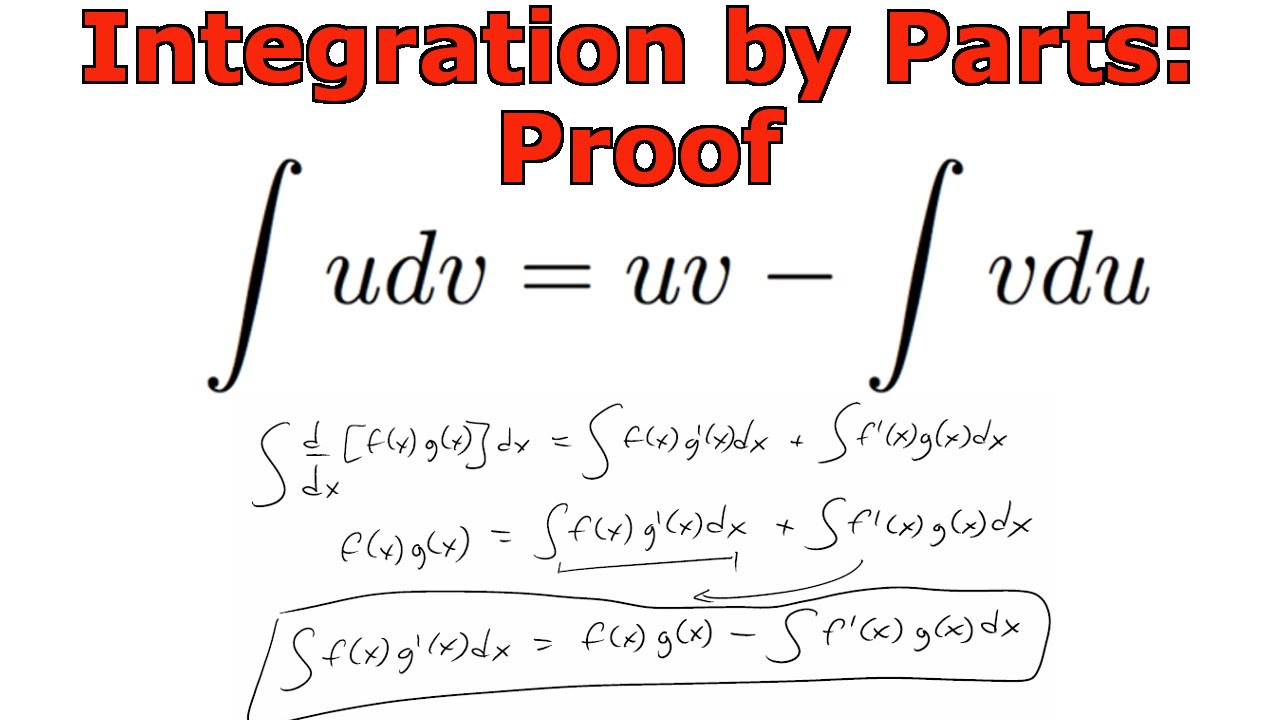
Given a single function to integrate, the typical strategy is to carefully separate this single function into a product of two functions u(x)v(x) such that the residual integral from the integration by parts formula is easier to evaluate than the.
A quotient rule integration by parts formula, apply the resulting integration formula to an example, and discuss reasons why this formula does not appear in calculus texts. R f(x)±g(x)dx = r f(x)dx± r g(x)dx in plain language, the integral of a constant times a function equals the constant times the derivative of the function and the derivative of a sum or difference. The advantage of the tabular integration method is that it can save huge time in solving the problem. Quotient rule in integration is known as integration by parts.
Always start with the “bottom” function and finish with the “underside” function squared.
This is also known as the quotient rule differentiation in. (2) uu u2 as an application of the quotient rule integration by parts formula, consider theintegral sin (x −1/2) dx. ∫ b a udv = uv|b a −∫ b a vdu ∫ a b u d v = u v | a b − ∫ a b v d u. U + du assume f(x) and g(x) are.
The quotient principle is an official rule for identifying problems where one function is divided by another.
Note that the uv|b a u v | a b in the first term is just the standard integral evaluation notation that you should be familiar with at this point. For integrating a quotient of two functions, usually the rule for integration by parts is recommended: Differentiate the function f (x) ⇒. Integration by parts is a heuristic rather than a purely mechanical process for solving integrals;
We will apply the limit definition of the derivative:
1) on which derivative rule is the method of integration by parts based? In short, the quotien t rule is a way of differentiating the division of functions or the quotients. F ( x) − f ( c) x − c exists finitely. Dv u = v u + v u2 du.
To calculate the integration by parts, take f as the first function and g as the second function, then this formula may be pronounced as:
F ( u) f ( v) \frac { f ( u ) } { f ( v ) } f (v)f (u). The formula for the integral division rule is deduced from the integration by parts u/v formula. Then sin(x−1/2) x2 dx = 2cos(x−1/2) x1/2 + 2cos(x−1/2) x · 1 2 x−1/2 dx = 2cos(x−1/2) x1/2 −2 cos(x−1/2)· − By the quotient rule, if f (x) and g(x) are differentiable functions, then d integrating both sides of this equation, we get f that is, f(x) which may be rearranged to obtain f___
For integrating a quotient of two functions, usually the rule for integration by parts is recommended:
Remember the rule in the following manner. Integration by parts, definite integrals. Dv v v =+ du. (a) recall that the formula for integration by parts is obtained from the product rule.
We obtain a quotient rule integration by parts formula:
It gives the solution fairly accurate than the integration by parts method. Let u = x1/2, dv = sin(x−1/2) x3/2 dx, du = 1 2 x−1/2 dx,v= 2cos(x−1/2). Select rating give a quotient rule integration by parts formula 1/5 give a quotient rule integration by parts formula 2/5 give a quotient rule integration by parts formula 3/5 give a quotient rule integration by parts formula 4/5 give a quotient rule integration by parts formula 5/5. Letting u = g (x) and v = f (x) and observing that du = g (x) dx and dv = f (x) dx, we obtain a quotient rule integration by parts formula:
[ f ( x) g ( x)] ′ = g ( x) f ′ ( x) − f ( x) g ′ ( x) [ g ( x)] 2.
H ′ ( x) = [ f ( x) g. Recall the quotient rule for differentiation.