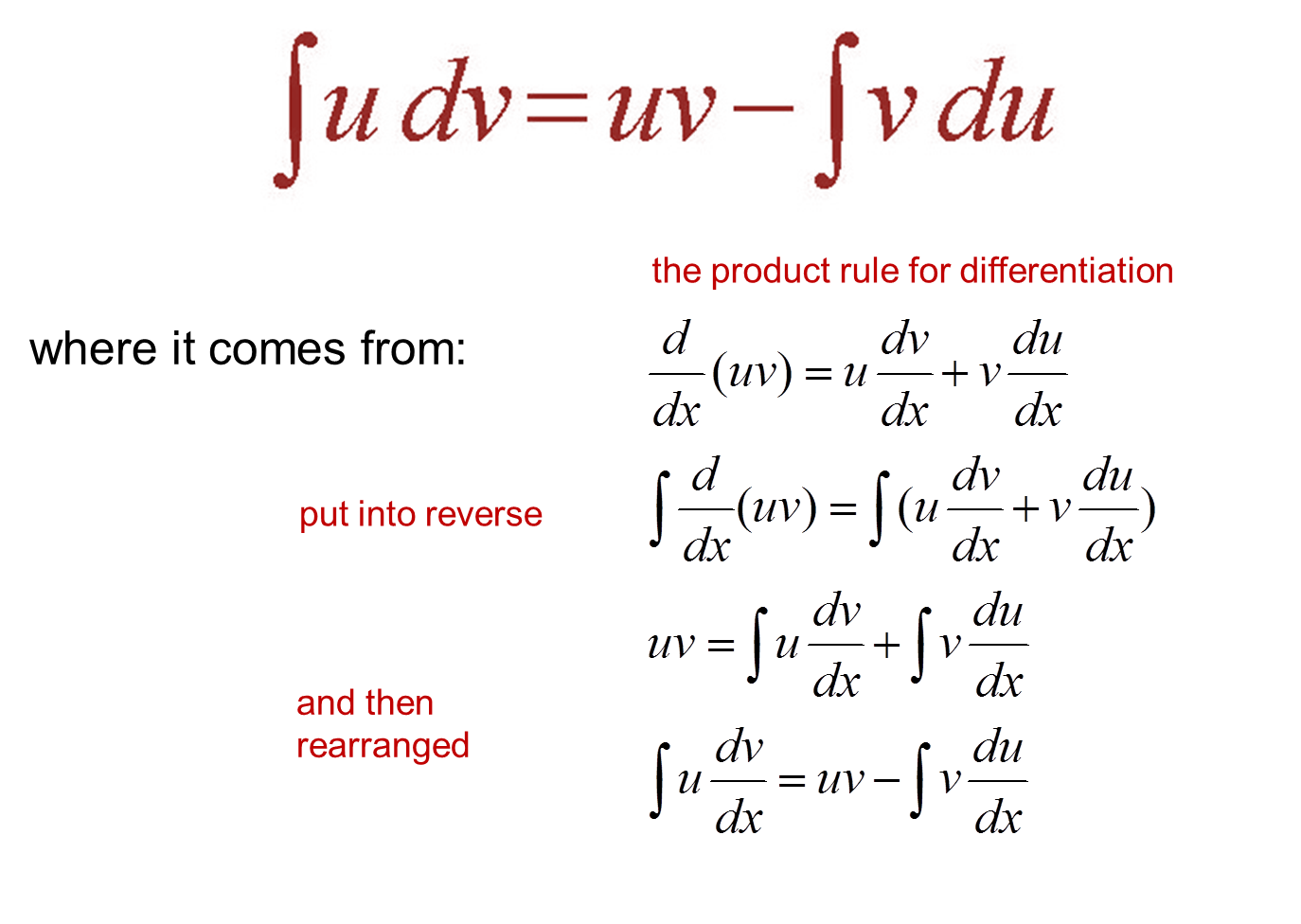Use the sum rule to split the integral on the right in two: You will see plenty of examples soon, but first let us see the rule: Integrating the product rule with respect to x;
core pure 3 notes integration by parts examples
There are many ways to integrate by parts in vector calculus.
(integration by parts) recall the product rule:
Note that u and v are both functions. Integrate the product rule f g0 = (fg)0 − f 0 g, and use the Product rule in differential form. Simplify the left side and split the integral on the right into two integrals:
Now, integrate both sides of this.
The first of the two. Next, rearrange the terms of the equation: R → r holds z f (x) g0(x) dx = f (x) g(x) − z f 0(x) g(x) dx. I will therefore demonstrate how to think about integrating by parts in vector calculus, exploiting the gradient product rule, the.
Theorem for all differentiable functions g,f :
D dx (f (x) g (x)) = integrate both sides with respect to x: Isolate one of the integrals: Derivation of the formula for integration by parts we already know how to differentiate a product: So many that i can't show you all of them.
Take u = f ( x) and v = g ( x).
For example, if we have to find the integration of x sin x, then we need to use this formula. This method is used to find the integrals by reducing them into standard forms. Integration by parts (or simply 'parts' for short) is often used to find the integrals of products of functions. There are, after all, lots of ways to put a vector differential form into an equation, and (at least) three dimensionalities of integral you might be trying to do!
∫ (f g)′dx =∫ f ′g +f g′dx ∫ ( f g) ′ d x = ∫ f ′ g + f g ′ d x.
Apply integration by parts formula, we have. Let their product be y. ∫ u v ′ d x = u v − ∫ v u ′ d x. Knowing how to derive the formula for integration by parts is less important than knowing when and how to use it.
Solve for the term r
Now integrate both sides of this equation: ∫ x 5 ln in x dx = ∫ ln in x x 5 dx. A rule exists for integrating products of functions and in the following section we will derive it. Applying the product rule of differentiation, we get.
And from that, we're going to derive the formula for integration by parts, which could really be viewed as the inverse product rule, integration by parts.
D ( u v) d x = u d v d x + v d u d x. With the product rule, you labeled one function “f”, the other “g”, and then. The first step is simple: Now, express the derivative product rule in differential form.
Let us derive the integration by parts formula using the product rule of differentiation.
If y = uv then dy dx = d(uv) dx = u dv dx +v du dx. To do this integral we will need to use integration by parts so let’s derive the integration by parts formula. So let's say that i start with some function that can be expressed as the product f of x, can be expressed as a product of two other functions, f of x times g of x. Here, the integrand is the product of the functions x and cosx.
The last line is the integration by parts formula:
We’ll start with the product rule. Vdu is really the product rule in disguise. Integration by parts is used to integrate when you have a product (multiplication) of two functions. Let f (x)=lnx and g' (x)= x 5 , then f' (x)= 1 x and g (x)= x 6 6.
U dv dx = d(uv) dx − v du dx.
(fg)0 = f 0 g + f g0. Consider two functions u and v. (f g)′ =f ′g+f g′ ( f g) ′ = f ′ g + f g ′. U is the function u(x) v is the function v(x) u' is the derivative of the function u(x)
In a way, it’s very similar to the product rule, which allowed you to find the derivative for two multiplied functions.
From the integration by parts formula discussed above, u is the function u(x) v is the function v(x) The trick we use in such circumstances is to multiply by 1 and take du/dx = 1. We have that z d dx (uv)dx = z du dx vdx+ z dv dx udx ) uv = z duv + z dvu = z vdu+ z udv: D d x ( u v) = u d d x v + v d d x u.
∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx.
D/dx (uv) = u (dv/dx) + v (du/dx) we will rearrange the terms here. For example, you would use integration by parts for ∫x · ln(x) or ∫ xe 5x. Integral form of the product rule remark: Multiply both sides of the equation by the differential element d x.
To see why the integration by parts formula is true, start with the product rule (uv)0= u 0v + v u that can be also written as d dx (uv) = du dx v + dv dx u:
Derive the integration by parts formula using the product rulevideo by: The integration by parts formula is an integral form of the product rule for derivatives: Integration by parts formula is used for integrating the product of two functions. Just rearrange the two products on the right side of the equation:
What is integration by parts?
Observation more information integration by parts essentially reverses the product rule for differentiation applied to (or ).: