In a perfectly inelastic collision, the colliding particles stick together. Velocity of the moving object, in m/s. The inelastic collision formula is articulated as.
Inelastic Collisions
The equation for the coefficient of restitution is:
In this type of collision, the kinetic energy does not remain conserved before and after the collision.
M1, m2 ,., mn is the mass of the individual particles in the system. Mass of body 2 = m 2. Homework equations i used these three equations: In a perfectly inelastic collision, the two collided bodies stick together and move with the same velocity v.
If it is 1 we have an elastic collision;
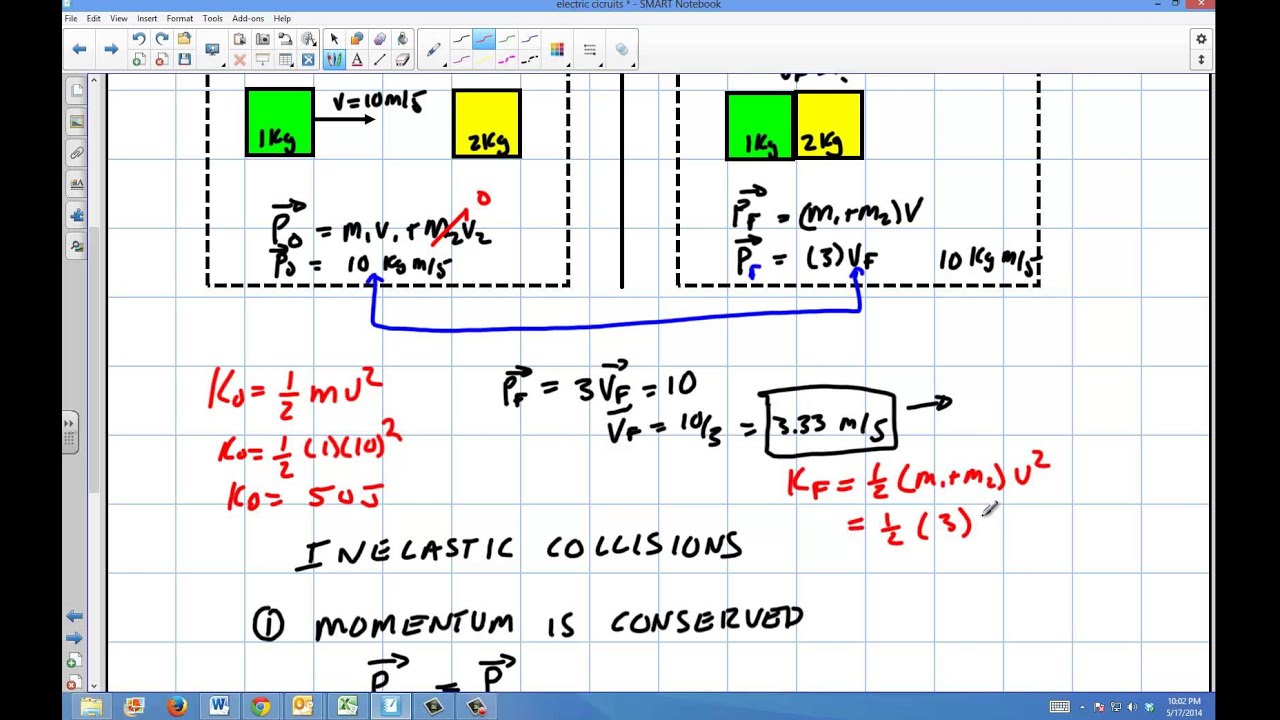
Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in. Inelastic collisions perfectly elastic collisions are those in which no kinetic energy is lost in the collision. We will start with the equation for an inelastic collision: If one body (a) with a velocity (a) strikes a second body (b) and sticks to it, then the resulting larger body will have a slower velocity.
In a perfectly inelastic collision, the difference in the velocities of two objects after a collision is zero because those objects stick together.
1.2 kg × m/s = 0.20 kg × v2. Any objects that collide in this way will reduce the total kinetic energy (and total velocity) by this ratio. The final velocity of the combined objects depends on the masses and velocities of the two objects that collided. Though kinetic energy is not conserved in these collisions, momentum is conserved and the equations of momentum can be used to understand the behavior of the components in this system.
Macroscopic collisions are generally inelastic and do not conserve kinetic energy, though of course the total energy is conserved as required by the general principle of conservation of energy.the extreme inelastic collision is one in which the colliding objects.
M1 v1 = (m1 + m 2) v2 equation 1 where m1 is the projectile mass and m2 is the target mass. Where the coefficient of restitution for a perfectly elastic collision is e = 1 and for a perfectly inelastic collision is e = 0. If each car is driven by a person with mass of 50.0 kg, how much kinetic energy is dissipated in a head on, perfectly inelastic collision? Collisions involve forces (there is a change in velocity).
The overall kinetic energy of the system internally is.
Imagine two of these cars performing in a stunt show. One car travels east with a speed of 106.0 km/h and the other travels west with a speed of 75.0 km/h. An inelastic collision is commonly defined as a collision in which linear momentum is conserved, but kinetic energy is not conserved. \frac {1} {2}m {v^2} + \frac {1} {2}m {v^2} = m {v^2} 21.
C r is the coefficient of restitution;
Mass of the stationary object, in kg. The impulse of the collision changes the velocity of car 1, and after the collision car 1 moves with uniform velocity v 2. Mass of the moving object, in kg. A perfectly inelastic collision is one in which the maximum amount of kinetic energy has been lost during a collision, making it the most extreme case of an inelastic collision.
The general equation for conservation of linear momentum for a system of particles is:
Before the collision car 1 and mass m move with uniform velocity v 1. The formula for inelastic collision: Most collisions in nature are inelastic collisions. The coefficient of restitution exists as a number between 0 and 1.
A collision of two particles in which the two particles remain together after the collision is called a perfectly inelastic collision.
Coefficient of restitution generally lies between 0 and 1. In perfectly inelastic collisions the momentum of the system is conserved but there is a change the kinetic energy of the system. This is when the objects that collide are equal in their masses. Velocity of the stationary object after collision, in m/s.
1 2 m v 2 + 1 2 m v 2 = m v 2.
The initial velocity of body 2 = u 2. The initial velocity of body 1 = u 1. Inelastic collisions, newton's laws, conservation of momentum, circular motion, frame transformations; V2 =1.2 / 0.20 = 6 m/s.
The momentum of the objects before the collision is conserved, but the total energy is not conserved.
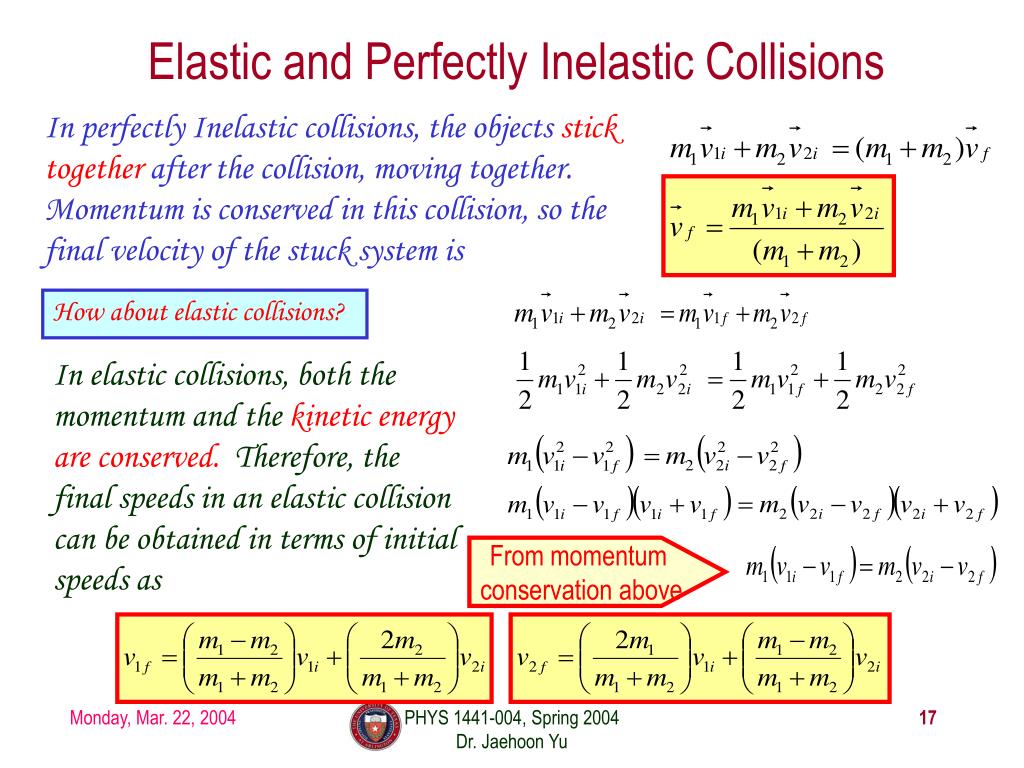
The final velocity of both the bodies = v. However, the momentum remains conserved. $$m_1v_{1i} + m_2v_{2i} = (m_1+m_2)v_f $$ These two equations give the same results as obtained for the elastic collision if e=1 and for the inelastic collision if e=0.
The inelastic collision equation is:
Physical sciences index classical mechanics index: Both the projectile speed before the collision, v1, and the combined speed after the collision, v2, are measured. If it is 0 we have a perfectly inelastic collision, see below. Coefficient of restitution is 0 for the perfectly inelastic collision.
In a center of momentum frame the formulas reduce to:
In a perfectly inelastic collision, two objects collide and stick together. V = m 1 u 1 +m 2 u 2 m 1 +m 2. In a perfectly elastic collision, we said that. Finds mass or velocity after collision.
Mass of body 1 = m 1.
This means that the coefficient of restitution for a perfectly inelastic collision is e = 0. The equation for the velocities after the collision becomes; To determine whether the collision is elastic or inelastic, calculate the total kinetic energy of the system both before and after the collision.