Integrals involving inverse trigonometric functions the derivatives of the six inverse trigonometric functions fall into three pairs. The inverse trigonometric functions are also known as the arc functions. 22 1 arctan du u c a u a a ³ 3.
12X1 T05 05 integration with inverse trig (2010)
∫ du u√u2−a2 = 1 a sec−1 u a +c ∫ d u u u 2 − a 2 = 1 a sec − 1 u a + c.
Y y) did we plug into the sine function to get x x.
Pdf file notebook file 5.1.1 (a. C is used for the arbitrary constant of integration that can only be determined if something about the value of the integral at some. The key is to know the derivatives. A.) b.) e.) it is assumed that you are familiar with the following rules of differentiation.
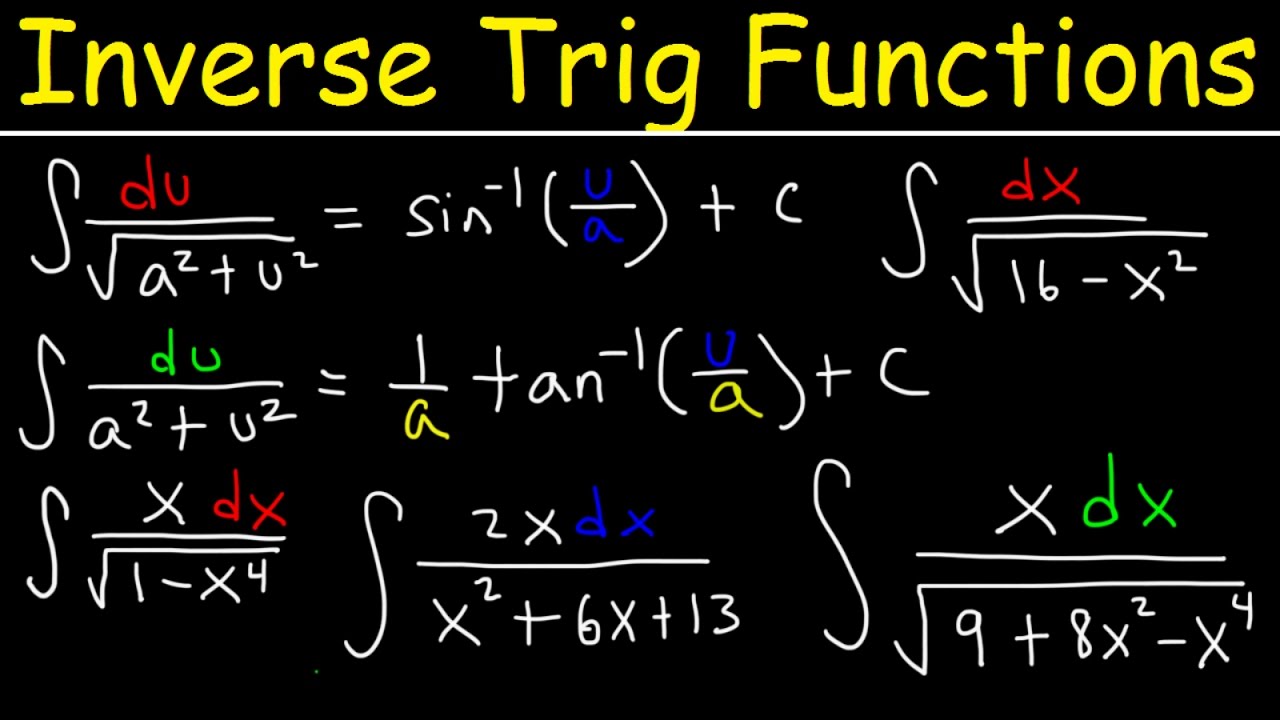
\[ \begin{align} ∫\dfrac{du}{\sqrt{a^2−u^2}} =\sin^{−1}\left(\dfrac{u}{a}\right)+c \\ ∫\dfrac{du}{a^2+u^2} =\dfrac{1}{a}\tan^{−1}\left(\dfrac{u}{a}\right)+c \\ ∫\dfrac{du}{u\sqrt{u^2−a^2}}.
So, to make sure we get a single value out of the inverse trig cosine function we use the following restrictions on inverse cosine. •use the method of completing the square to integrate a function. Up to 24% cash back inverse trigonometric functions. In the past, we’ve learned how inverse trigonometric functions can help us find unknown angles.
For example, and when listing the antiderivative that corresponds to each of the inverse trigonometric functions, you need to use only one member from each pair.
142 dx x ³ 2. ∫ du √a2−u2 = sin−1 u a +c ∫ d u a 2 − u 2 = sin − 1 u a + c. From derivatives, you can get these integral forms. In each pair, the derivative of one function is the negative of the other.
When you can recognize terms in the integrand that are direct derivatives of inverse trig functions, you can immediately write down the integral.
We can use and rearrange double angle identities, such as when given a squared trig function. The file links below are organized into categories based on the form of the integrand. Integration 383 review of basic integration rules you have now completed the introduction of the basic integration rules.to be efficient at applying these rules, you should have practiced enough so that each rule is committed to memory. For a complete list of integral formulas, see lists of integrals.
Although there are actually six inverse trig functions, remember that the.
For example, and when listing the antiderivativethat corresponds to each of the inverse trigonometric functions, you need to use only one member from each pair. This calculus video tutorial focuses on integration of inverse trigonometric functions using formulas and equations. The only difference is whether the integrand is positive or negative. However, only three integration formulas are noted in the rule on integration formulas resulting in inverse trigonometric functions because the remaining three are negative versions of the ones we use.
Y = sin−1x ⇔ siny =x for − π 2 ≤ y ≤ π 2 y = sin − 1 x ⇔ sin.
Here is the definition of the inverse sine. ∫ du a2+u2 = 1 a tan−1 u a +c ∫ d u a 2 + u 2 = 1 a tan − 1 u a + c. In each pair, the derivative of one function is the negative of the other. We can use the chain rule when the variable in brackets is more complex than x, for example , as we have divided by the derivative of the brackets.
So, evaluating an inverse trig function is the same as asking what angle ( i.e.
We don’t want to have to guess at which one of the infinite possible answers we want. ∫ 0 1 2 d x 1 − x 2 = sin −1 x | 0 1 2 = sin −1 1 2 − sin −1 0 = π 6 − 0 = π 6. 22 1 sec du u arc c u u a aa ³ why are there only three integrals and not six? Integrals resulting in inverse trig functions are normally challenging to integrate without the formulas derived from the derivative of inverse functions.
Return to top of page
When calculating integrals of inverse trig functions, we use integration by parts,. Sin(sin 1 x) = x for 1 x 1 and sin 1(sinx) = x for ˇ 2 x ˇ 2 similarly, one obtains cos 1 xon [ 1;1] is the inverse of cosxfor 0 x ˇand the analogous cancellation rule holds. Examples include techniques such as int. Some of the following trigonometry identities may be needed.
Integrals involving inverse trigonometric functions the derivatives of the six inverse trigonometric functions fall into three pairs.
Objectives integrals involving inverse trigonometric functions Up to 24% cash back section 5.7 inverse trigonometric functions: The only difference is whether the integrand is positive or negative. Before attempting to use an inverse trigonometric substitution, you should examine to see if a direct substitution, which is simpler, would work.
The following is a list of indefinite integrals of expressions involving the inverse trigonometric functions.
22 arcsin du u c au a ³ 2. There are six inverse trigonometric functions. 5 inverse trig function rules 5.1 inverse sine and cosine. The function tan 1 xon (1 ;1) has the inverse tanxfor ˇ 2 <x<ˇ 2:the inverses of other.
We can go directly to the formula for the antiderivative in the rule on integration formulas resulting in inverse trigonometric functions, and then evaluate the definite integral.
∫ 0 1 2 d x 1 − x 2 = sin −1 x | 0 1 2 = sin −1 1 2 − sin −1 0 = π 6 − 0 = π 6. Θ = cos − 1 ( x) − 1 ≤ x ≤ 1 a n d 0 ≤ θ ≤ π θ = cos − 1 ( x) − 1 ≤ x ≤ 1 a n d 0 ≤ θ ≤ π. There are six inverse trigonometric functions. The following integration formulas yield inverse trigonometric functions:
Integration formulas resulting in inverse trigonometric functions the following integration formulas yield inverse trigonometric functions:
•review the basic integration rules involving elementary functions. However, only three integration formulas are noted in the rule on integration formulas resulting in inverse trigonometric functions because the remaining three are negative versions of the ones we use.