This page lists some of the most common antiderivatives. Integration in a sense is the opposite of that process. So the integral of 2 is 2x + c, where c is a constant.
2.1.11.12.4 Chapter 4 Indefinite Integrals
Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z
Fundamental rules ( ) 𝑥 =0 ∫ 𝑥=𝑥+𝐶
For this reason, when we integrate, we have to add a constant. [note that you may need to use more than one of the above rules for one integral]. Xn+1 n+ 1 + c; ∫ sin 10(x) cos (x) dx.
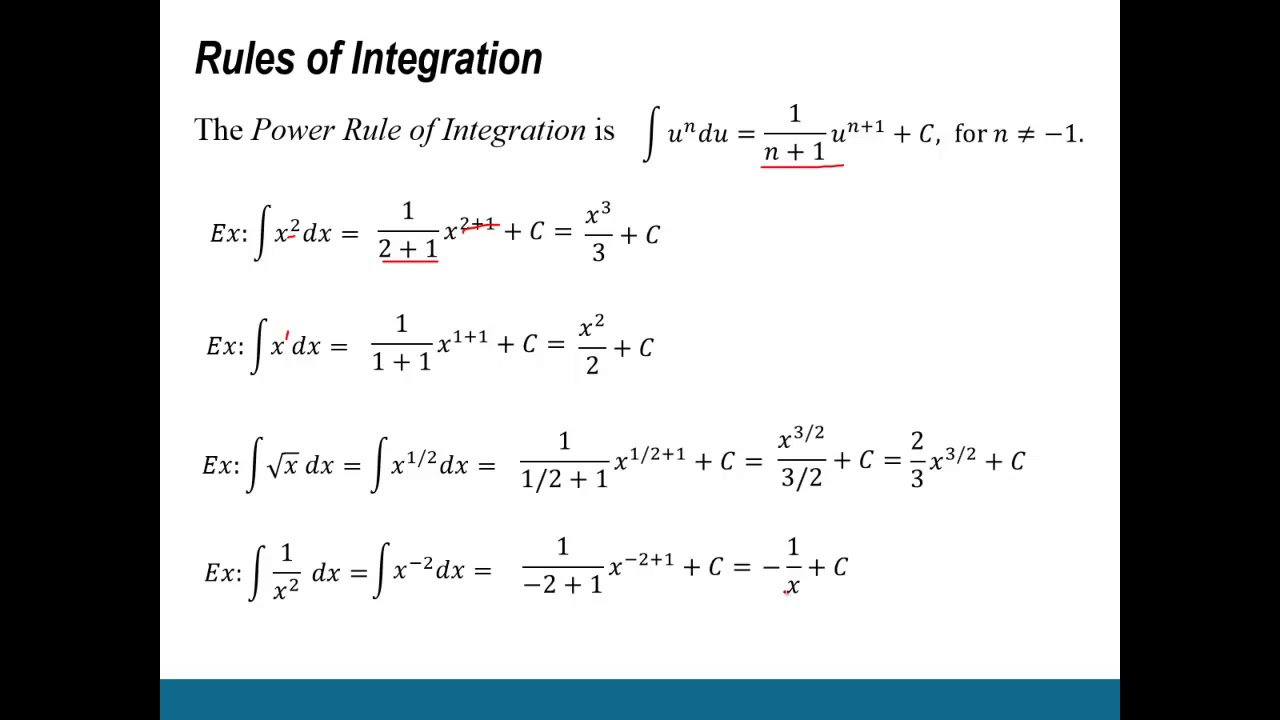
This can be obtained by the power rule of integration that says ∫x n dx = x n+1 / (n+1) + c, where 'c' is the integration constant (which we add after the integral of.
To represent the antiderivative of “f”, the integral symbol “∫” symbol is introduced. Basic integration formulas and the substitution rule 1the second fundamental theorem of integral calculus recall fromthe last lecture the second fundamental theorem ofintegral calculus. Z ax dx= ax ln(a) + c with base e, this becomes: If y = 2x, dy/dx = 2.
∫ [sin (x) + x 5] dx.
Apr 11 6:07 pm (7 of 15). Indefinite integrals are also called “antiderivatives”. If y = 2x + 3, dy/dx = 2. ∫ (y 2 + 2).dy = ∫ y 2.dy + ∫ 2.dy = y 3 /3 + 0 (applying power and constant rule) = y 3 /3 + c.
Dt dt = 1 or dx dx = 1.
These are some of the most frequently encountered rules for differentiation and integration. It is a summing process used to add up infinitesimally small quantities. While differentiation has straightforward rules by which the derivative of a complicated function can be found by differentiating its simpler component functions, integration does not, so tables of known integrals are often useful. Where stands for nth differential coefficient of u and stands for nth integral of.
In both of these rules, integration is applied separately on the functions and then they are subtracted or added accordingly.
Generally, we can write the function as follow: Let f (x) be a function. If n= 1 exponential functions with base a: The integration rules are rules used to integrate different types of functions.
Integration rules and summary vocabulary:
U is the function u(x) v is the function v(x) u' is the derivative of the function u(x) Integration rules and formulas integral of a function. Apr 11 6:05 pm (4 of 15) title: You will see plenty of examples soon, but first let us see the rule:
Apr 11 6:03 pm (2 of 15) title:
Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. Apr 11 6:06 pm (6 of 15) title: Assume k (this just means “k” is a constant) ³ k kdx x c ³ dx x c multiples, sums, and differences: We saw in the first part of this tutorial how differentiation is a way of working out the rate of change of functions.
³³k ( ) =k ( ) f x dx f x dx
Data integration rules for lightning data and company info give currency amounts in us dollars. ∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx. Integration is the reverse of differentiation. Where “c” is the arbitrary constant or constant of integration.
Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>:
For the following, let u and v be functions of x, let n be an integer, and let a, c, and c be constants. Integration is the basic operation in integral calculus. Apr 11 6:05 pm (5 of 15) title: We have seen that ∫ 2x dx = x 2 + c as d/dx (x 2) = 2x.
(d/dx) [f (x)+c] = f (x), where x belongs to the interval i.
The copyright holder makes no representation about the accuracy, correctness, or If n6= 1 lnjxj+ c; When the multiple currencies feature is enabled, lightning data rules convert numerical fields mapped to a currency type field from us dollars to the record currency. Apr 11 6:04 pm (3 of 15) title:
Then the collection of all its primitives is called the indefinite.
There are many rules of integration that help us find the integrals. 1 1 nn x dx x c n ³ , nz 1 specific rules based on the power rule: Du u c 1 1 n udu cn u n ln du uc u edu e cuu 1 ln adu a cuu a sin cosudu u c cos sinudu u c sec tan2 udu u c csc cot2 uuc csc cot cscuudu uc sec tan secuudu uc 22 1 arctan du u c au a a 22 arcsin du u c au a If y = 2x + 5, dy/dx = 2.
Apr 11 5:59 pm (1 of 15) title:
Theorem let f(x) be a continuous function on the interval [a,b]. Integration is a summing process. ∫ (fx + gx).dx = ∫ fx.dx + ∫ gx.dx. ©2005 be shapiro page 3 this document may not be reproduced, posted or published without permission.
A s shaped symbol is used to mean the.
Section 5.1 simple power rule: Use the table of integral formulas and the rules above to evaluate the following integrals. The power rule, the sum and difference rules, the exponential rule, the reciprocal rule, the constant rule, the substitution rule, and the rule of integration by parts are the prominent ones. Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= +