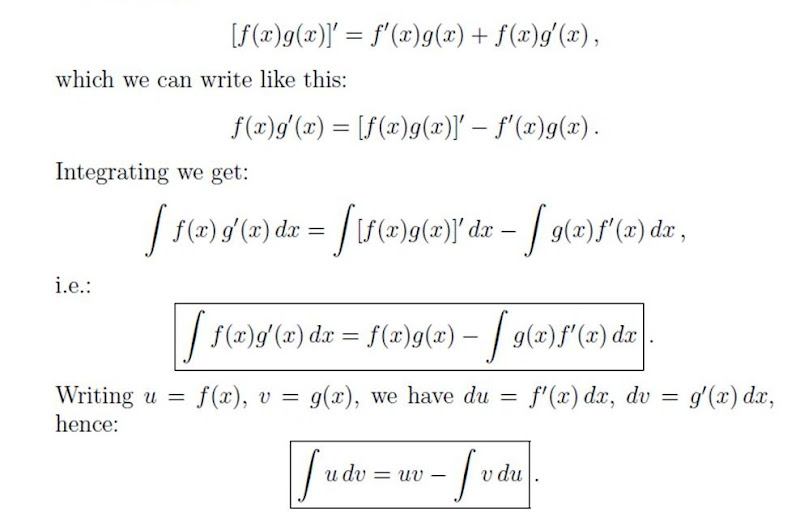( 6 9 4 3)x x x dx32 3 3. Example 7.11 let us illustrate with an example we’ve already seen. Any polynomial can be written as a product of factors of the form x
Lecture in Integration by Parts Pinoybix Engineering
There are no simple rules for deciding which order to do the integration in.
A good diagram is essential.
These integrals are called indefinite integrals or general integrals, c is called a constant of integration. •theconstantrule z a dx = ax +c •thepolynomialrule z xn dx = xn+1 n+1 +c = 1 n+1 xn+1 +c •thescalarmultiplerule z. The difference rule of integration is similar to the sum rule. U =sin x (trig function) (making “same” choices for u and dv) dv =ex dx (exponential function) du =cosx dx v =∫ex dx =ex
7.1 overview 7.1.1 let d dx f (x) = f (x).
R [(x−1)5 +3(x−1)2 +5]dx solution. X/y of right/bot ring to x/y of left/top ring limits : General integration deflnitions and methods: (5 8 5)x x dx2 2.
Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>:
F x e x3 ln , 1,0 example: Do the integration with respect to x first. ( ) 3 x dx Then, we write∫f dx()x = f (x) + c.
6.2 integration by substitution in problems 1 through 8, find the indicated integral.
Sometimes you have to integrate powers of secant and tangents too. Use the basic integration formulas to find indefinite integrals. Example 2 using the log rule with a change of variables find solution if you let then multiply and. Use substitution to find indefinite integrals.
All these integrals differ by a constant.
For example, in leibniz notation the chain rule is dy dx = dy dt dt dx. 7.1.3 geometrically, the statement∫f dx()x = f (x) + c = y (say) represents a family of. ∫ f dx + ∫ g dx: For the following, let u and v be functions of x, let n be an integer, and let a, c, and c be constants.
These are some of the most frequently encountered rules for differentiation and integration.
Du u c 1 1 n udu cn u n ln du uc u edu e cuu 1 ln adu a cuu a sin cosudu u c cos sinudu u c sec tan2 udu u c csc cot2 uuc csc cot cscuudu uc sec tan secuudu uc 22 1 arctan du u c au a a 22 arcsin du u c au a Substituting u =2x+6and 1 2 du = dx,youget z (2x+6)5dx = 1 2 z u5du = 1 12 u6 +c = 1 12 (2x+6)6 +c. ∫(f + g) dx = ∫f dx + ∫g dx. You are doing the integral:
Use the trapezoidal rule of integration to solve problems, 3.
Z x2 −2 √ u du dx dx = z x2 −2 √ udu. Power rule (n≠−1) ∫ x n dx: Xn+1 n+ 1 + c; 7.1.2 if two functions differ by a constant, they have the same derivative.
Substituting u = x−1 and du = dx,youget z £ (x−1)5 +3(x−1) 2+5 ¤ dx = z (u5 +3u +5)du = = 1 6 u6 +u3 +5u+c = = 1 6
The sum rule explains the integration of sum of two functions is equal to the sum of integral of each function. ( 2 3)x x dx 2 23 8 5 6 4. To find the integral (7.33) dx x a x b we check that (7.34) 1 x a x b 1 a b 1 x a 1 x b so that (7.35) dx x a x b 1 a b ln x a ln x b c 1 a b ln x a x b c the trick 7.34 can be applied to any rational function. If n6= 1 lnjxj+ c;
∫ex cosx dx u =cos x (trig function) dv =ex dx (exponential function) du =−sin x dx v =∫ex dx =ex ∫ex cosx dx =uv−∫vdu =cosx ex −∫ex (−sin x) dx =cosx ex +∫ex sin x dx second application of integration by parts:
Derive the trapezoidal rule of integration, 2. Use substitution to evaluate definite integrals. Dx x xx 1 5. See examples 1, 2 and 3 on page 310 and 311 of stewart.
Axis use fy( ), gy( ), ay( ) and dy.
As before, du = du dx dx and so with u = 9+x and du dx = 1 it follows that du = du dx dx = dx the integral becomes z x=3 x=1 u2 du where we have explicitly written the variable in the limits of integration to emphasise that those ∫ (f + g) dx: Basic integration formulas and the substitution rule 1the second fundamental theorem of integral calculus recall fromthe last lecture the second fundamental theorem ofintegral calculus. Some general information about each method of computing and some examples.
Axis use f x( ), gx( ), ax( ) and dx.
Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions The same is true of our current expression: Since u = 1−x2, x2 = 1− u and the integral is z − 1 2 (1−u) √ udu. To x/y of outer cyl.
Fundamental rules ( ) 𝑥 =0 ∫ 𝑥=𝑥+𝐶
Theorem let f(x) be a continuous function on the interval [a,b]. Example suppose we wish to find z 3 1 (9+x)2 dx we make the substitution u = 9+x. ∫(x + x 2)dx = ∫x dx + ∫x 2 dx = x 2 /2 + x 3 /3 + c. After reading this chapter, you should be able to:
X n+1 n+1 + c:
Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z Rings cylinders (( )22 ( ) ) a =π outer radius inner radius− a =2π(radius width / height)( ) limits: If n= 1 exponential functions with base a: Z ax dx= ax ln(a) + c with base e, this becomes:
The rate of change of sales of a brand new soup (in thousands per month) is given by r(t) = + 2, where t is the time in months that the new product has been on the market.
Use implicit differentiation to find dy/dx given e x yxy 2210 example: