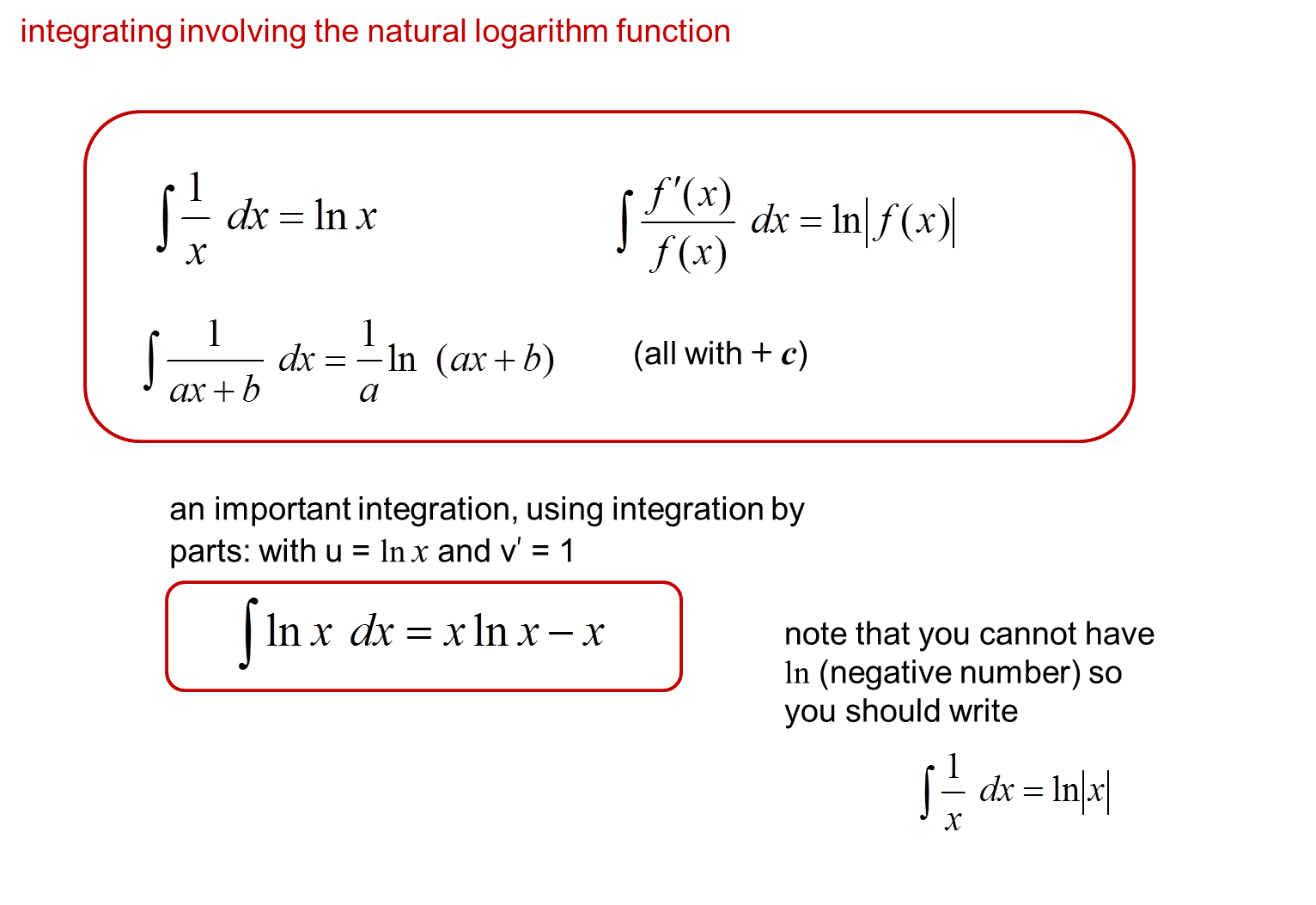
D ln(u) dx = d ln du (u) du dx = 1 u u0 ⇒ d ln(u) dx (x) = u0(x) u(x). ∫ ln ( x) d x = x ln ( x) − x + c. We use the chain rule.
LN LN JapaneseClass.jp
The log rule for integration can be written in three different ways:
With x as a variable (1) or change of variables u (2).
It gives us the indefinite integral of a variable raised to a power. In general, if ∫f(x) dx = ϕ(x) + c, then. 1 xln|x| = 1/x ln|x|. Integratingln x\ln x lnx.
So z 1 xln|x| dx = z 1/x ln|x| dx = ln|ln|x||+c.
Use implicit differentiation to find dy/dx given e x yxy 2210 example: Power rule sum rule different rule multiplication by constant product rule 1 4 ln 4x 1 c 1 4 ln u c u 4x 1. X2 ln x2 c 2 ln x c 2 x dx 2 1 x dx du u dx, d dx ln u u u d dx ln x 1 x u u dx lnexample 4.
Z undu = un+1 n +1 + c, n 6= ¡1 z du u = ln(u)+ c z eudu = eu + c z audu = au lna + c z cos(u)du = sin(u)+ c z sin(u)du = ¡cos(u)+ c z sec2(u)du = tan(u)+ c z
∫ (1 / 2) ln (x) dx = (1 / 2) ∫ ln (x) dx. Sal finds the definite integral of (6+x²)/x³ between 2 and 4. Example find z 1 xln|x| dx. Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>:
Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= +
This is the integral of ln (x) multiplied by 1 / 2 and we therefore use rule 2 above to obtain: The general rule for the integral of natural log is: For this solution, we will use integration by parts: The fundamental theorem of calculus implies ln0(x) = 1 x.
C c is the constant of integration, and this notation.
( x) as a rule or technique is unheard of. The power rule for integration, as we have seen, is the inverse of the power rule used in differentiation. 1 4 1 u du 1 4x 1 dx 1 4 1 4x 1 4 dx u 4x 1, du 4 dx. Xn+1 n+ 1 + c;
If n= 1 exponential functions with base a:
Ln 2ax+b a +2ax2+bx+c # $ % & ' (logarithms (43)!lnxdx=xlnxx (44) ln(ax) x!dx= 1 2 (ln(ax))2 (45)!ln(ax+b)dx= ax+b a ln(ax+b)x (46)!ln(a2x2±b2)dx=xln(a2x2±b2)+ 2b a tan1 ax b # $% & '(2x (47) ln(a2!b2x2)dx=xln(a2!b2x2)+ 2a b tan!1 bx a # $% & '(!2x (48)!ln(ax2+bx+c)dx= 1 a 4acb2tan1 2ax+b 4acb2 # $% & '(!!!!!2x+ b 2a +x # $% & '(ln(ax2+bx+c) (49)!xln(ax+b)dx= b. Ax n d x = a. 10 rows integral of natural logarithm (ln) function. To do that, he has to use the integral of 1/x, which is ln(x).
Z ax dx= ax ln(a) + c with base e, this becomes:
The important rules for integration are: So we rewrite the integrand slightly differently: If n6= 1 lnjxj+ c; Du u c 1 1 n udu cn u n ln du uc u edu e cuu 1 ln adu a cuu a sin cosudu u c cos sinudu u c sec tan2 udu u c csc cot2 uuc csc cot cscuudu uc sec tan secuudu uc 22 1 arctan du u c au a a 22 arcsin du u c au a
We now use formula 4.3 in the table of integral formulas to evaluate ∫ ln (x) dx.
Subtract “x” from the right side of the equation: Let’s say you had the basic function y = ln(x). Example 2 using the log rule with a change of variables find solution if you let then multiply and divide by 4. In the equation above, c.
Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z sin(x)dx= cos(x) + c z
This is a different rule from the log rule for integration, which allows you to find integrals for functions like 1/x. Which rule you use depends on what is in your denominator. In any of the fundamental integration formulae, if x is replaced by ax+b, then the same formulae is applicable but we must divide by coefficient of x or derivative of (ax+b) i.e., a. C c will be used throughout the wiki.
The integral of the natural logarithm.
F x e x3 ln , 1,0 example: Here is the power rule once more: Remember that the derivative of ln|x| is 1 x. An alternate form (3) is allowed because du = u′ dx [1].
When one speaks of techniques, they usually include integration by substitution, integration by parts, trig substitutions, partial fractions, etc.
Now the numerator is the derivative of the denominator. With introductory calculus in mind,. Ln(x) = z x 1 dt t ⇒ ln0(x) = 1 x.