I have a feeling it might have to do with Theorem let f(x) be a continuous function on the interval [a,b]. As you can see, it is just as simple to solve.
Example 24 Verify that y=c1 eax cos bx + c2 eax sin bx
= 1 a + b i e ( a + b i) x = a − b i a − b i ⋅ 1 a + b i e ( a + b i) x = a − b i a 2 + b 2 e ( a + b i) x.
∫ 1 dx = x + c.
= e a x a 2 + b 2 ( a − b i) ( cos. In what follows, c is a constant of integration and can take any constant value. To integrate e^ax, also written as ∫e ax dx, we notice that it is an exponential and one of the easiest in calculus to perform. Apr 11 6:03 pm (2 of 15) title:
If n= 1 exponential functions with base a:
Apr 11 6:04 pm (3 of 15) title: B x d x = ∫ e a x ( cos. Apr 11 6:07 pm (7 of 15). In any of the fundamental integration formulae, if x is replaced by ax+b, then the same formulae is applicable but we must divide by coefficient of x or derivative of (ax+b) i.e., a.
Z ax dx= ax ln(a) + c with base e, this becomes:
Apr 11 5:59 pm (1 of 15) title: Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + Ax n d x = a. B x) d x = ∫ e ( a + b i) x d x.
1.1 dx = x + c 1.2 k dx = k x + c , where k is a constant.
Or 2 3 ( a)3=2 4 15 x a)5=2; ∫ x n dx = ( (x n+1 )/ (n+1))+c ; , where a is any positive constant not equal to 1 and is the natural (base e) logarithm of a. There is also another version of this integral in the form, e^ax+b or sometimes written e^ (ax+b).
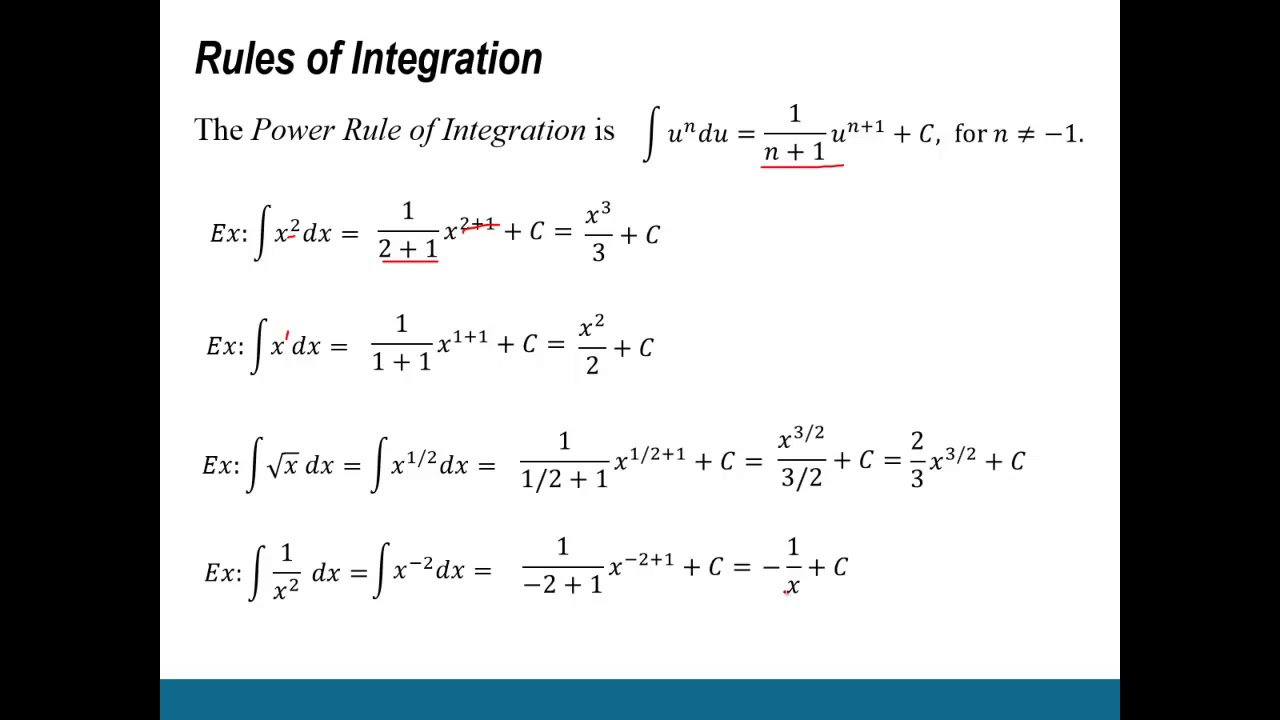
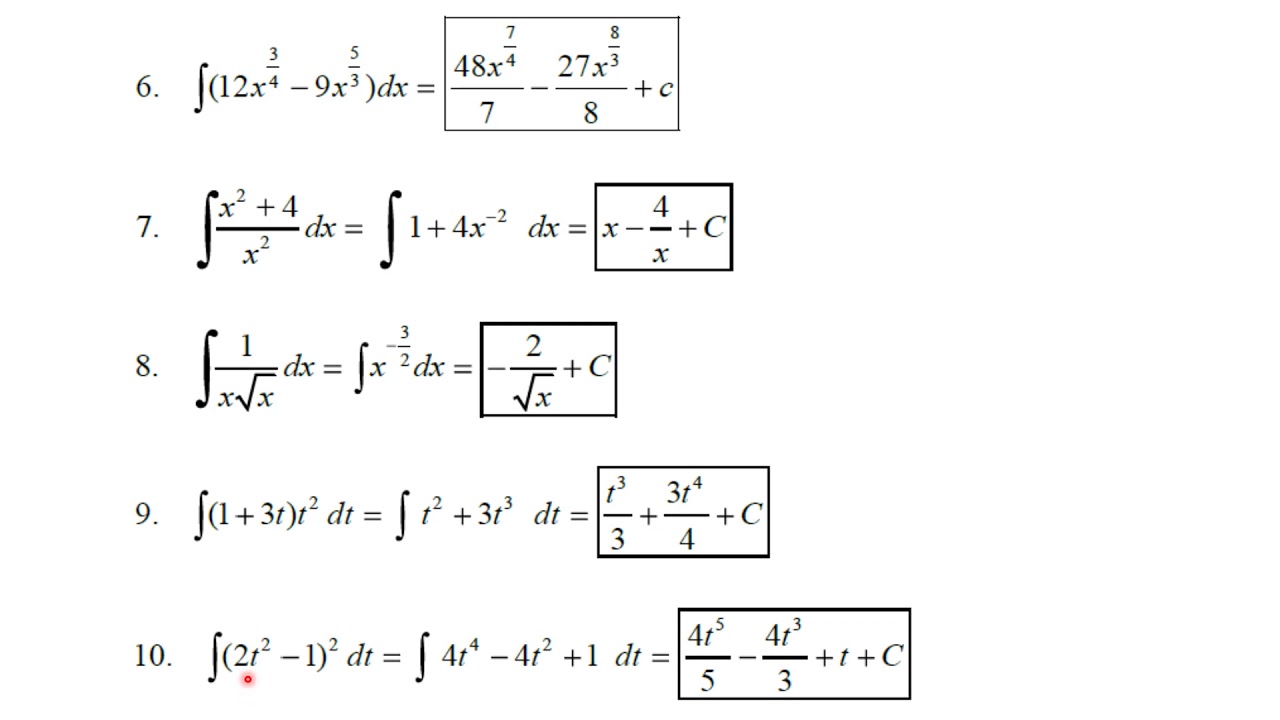
Here is the power rule once more:
3 2;cos2 ax (65) z. Basic integration formulas and the substitution rule 1the second fundamental theorem of integral calculus recall fromthe last lecture the second fundamental theorem ofintegral calculus. Apr 11 6:05 pm (4 of 15) title: ∫ cos x dx = sin x + c.
Apr 11 6:06 pm (6 of 15) title:
These formulas lead immediately to the following indefinite integrals : And dv = en ax now, find du and v. General integration deflnitions and methods: B x + i sin.
Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;
As you can see, the general rule is very simple, and worth remembering. ∫ sec 2 x dx = tan x + c. Treat a and n as constants. Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>:
∫ sec x (tan x) dx = sec x + c.
Apr 11 6:05 pm (5 of 15) title: ∫ a dx = ax+ c. The following problems involve the integration of exponential functions. B x + i sin.
Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z
2a 3 (x a)3 =2+ 2 5 (x a)5; Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here. (20) z x p x (adx= 8 <: Sin x, cos x, tan x, cot x, sec x and csc x.
We always appreciate your feedback.
Or 2 15 (2 a+ 3 x)( )3= (21) z p ax+ bdx= 2b 3a + 2x 3 p ax+ b (22) z (ax+ b)3=2 dx= 2 5a (ax+ b)5=2 (23) z x p x a dx= 2 3 (x 2a) p x a (24) z r It gives us the indefinite integral of a variable raised to a power. The power rule for integration, as we have seen, is the inverse of the power rule used in differentiation. In general, if ∫f(x) dx = ϕ(x) + c, then.
How to integrate $$\int_{0}^{\pi/2} e^{−2x}\sin(3x)\rm dx $$ i have attempted to this question with integration by parts, but i'm hitting a lot of walls.
The list of basic integral formulas are. Use integration by parts to establish the reduction formula х eax ſxc axdx *s*n_1_ axdx, ato a first, select appropriate values for u and dv.