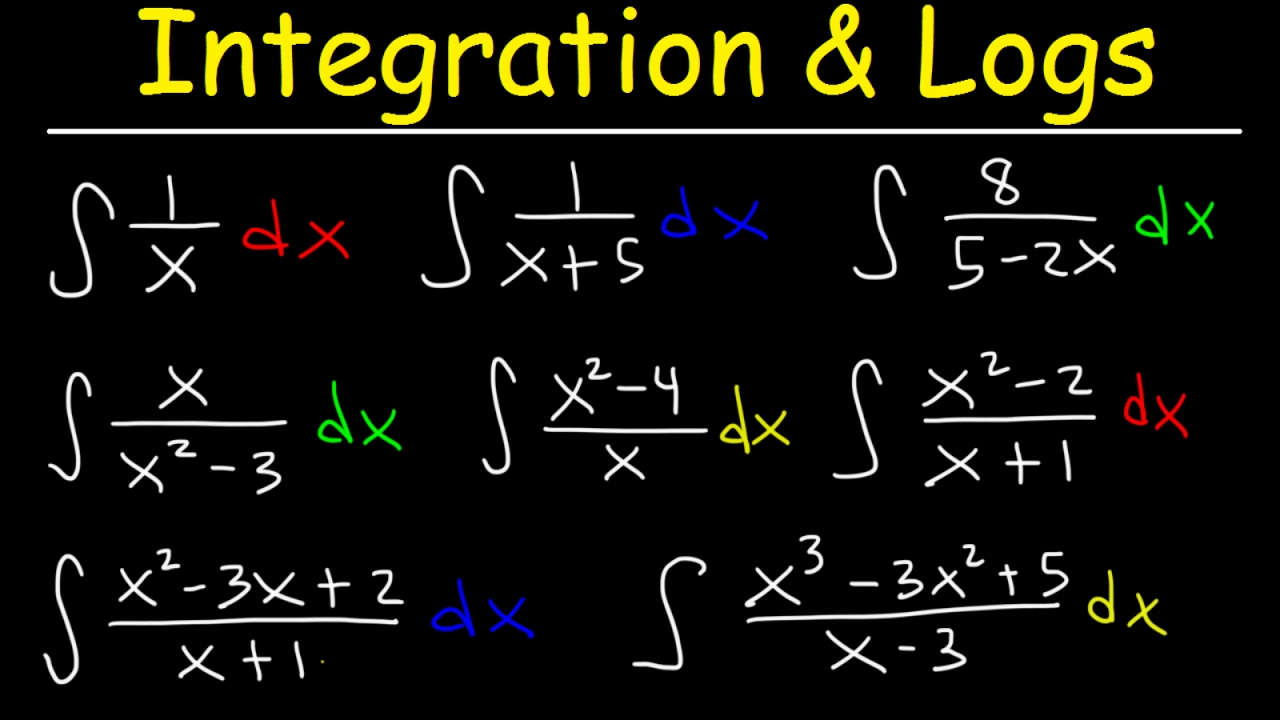In general, if ∫f(x) dx = ϕ(x) + c, then. Solve the integral using the usual rules of integration: As per the power rule of integration, we know ∫ x n dx = (x n+1)/ (n+1)+ c.
PPT Basic Integration Rules PowerPoint Presentation
There are many rules of integration that help us find the integrals.
Where “c” is the arbitrary constant or constant of integration.
³³k ( ) =k ( ) f x dx f x dx This gives you the differential du = cos x dx. (d/dx) (sinx + c)= cos x. ( f ( x)) + c.
Tan2 u sec2 u tan2 2x dx.
The formula for the integral division rule is deduced from the integration by parts u/v formula. It gives us the indefinite integral of a variable raised to a power. This section looks at integration by parts (calculus). Substitute du for cos x dx in the integral:
Section 5.1 simple power rule:
3.split the rational function into a sum of partial fractions with unknown constants on top as follows: Assume k (this just means “k” is a constant) ³ k kdx x c ³ dx x c multiples, sums, and differences: Integration using completing the square and the derivative of. Let u = sin x.
Thus by bezout (extended euclidean algorithm) there are b, c ∈ q [ x] such that b d ′ + c d = a / ( 1 − k).
Integrating functions using long division and completing the square. We obtain a quotient rule integration by parts formula: Integrands to the basic integration rules. It comes from the integration of 1 t, which is ln.
Then sin(x−1/2) x2 dx = 2cos(x−1/2) x1/2 + 2cos(x−1/2) x · 1 2 x−1/2 dx = 2cos(x−1/2) x1/2 −2 cos(x−1/2)· −
Declare a variable as follows and substitute it into the integral: 2.factor the denominator into unique linear factors or irreducible quadratics. The general rule for integrals like this is that if you have an equation of the form where the numerator is the derivative of the denominator, eg: Integration rules and summary vocabulary:
(d/dx) (sinx + c)= cos x +0.
The power rule, the sum and difference rules, the exponential rule, the reciprocal rule, the constant rule, the substitution rule, and the rule of integration by parts are the prominent ones. 1 2 tan uln 2 u 2 c 1 2 sec2 u du 1 2 du 1 2antiderivatives given by the sec2 u 1 du tan2 2x dx u 2x 1 2 tan2 u du u 2x, du 2 dx tan2 u sec2 u 1. Now you have an expression that you can integrate: What is the integration of √x?
Differentiate the function u = sin x.
Generally, we can write the function as follow: So, equation (1) can be written as. 1 2 tan 2x x c. When using this formula to integrate, we say we are integrating by parts.
The power rule for integration, as we have seen, is the inverse of the power rule used in differentiation.
In mathematics, integration by part basically uses the ilate rule that helps to select the first function and second function in the integration by parts method. Here is the power rule once more: Differentiate the function f (x) ⇒. (2) as an application of the quotient rule integration by parts formula, consider the integral sin(x−1/2) x2 dx.
This is the currently selected item.
In any of the fundamental integration formulae, if x is replaced by ax+b, then the same formulae is applicable but we must divide by coefficient of x or derivative of (ax+b) i.e., a. It is used when integrating the product of two expressions (a and b in the bottom formula). General integration deflnitions and methods: Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= +
Dv u = v u + v u2 du.
8.1 basic integration rules 511 procedures for fitting integrands to basic integration rules technique example expand (numerator). Substitute sin x for u: Integration by parts formula, ∫u (x).v (x).dx =. Let u = x1/2, dv = sin(x−1/2) x3/2 dx, du = 1 2 x−1/2 dx,v= 2cos(x−1/2).
1 1 nn x dx x c n ³ , nz 1 specific rules based on the power rule:
Also, any real number “c” is considered as a constant function and the derivative of the constant function is zero. ∫[f(x) + g(x)] dx = ∫f(x)dx + ∫g(x) dx From the product rule, we can obtain the following formula, which is very useful in integration: Apply the common integral ∫ 1 ⁄ x dx = ln |x| + c:
Du u c 1 1 n udu cn u n ln du uc u edu e cuu 1 ln adu a cuu a sin cosudu u c cos sinudu u c sec tan2 udu u c csc cot2 uuc csc cot cscuudu uc sec tan secuudu uc 22 1 arctan du u c au a a 22 arcsin du u c au a
Indefinite integrals are also called “antiderivatives”. The sum rule states that the integral of the sum of two functions is the sum of their separate integrals: Fun‑6 (eu), fun‑6.d (lo), fun‑6.d.3 (ek) google classroom facebook twitter. Ax n d x = a.
Let us take an integrand function which is equal to u (x)v (x).
By squarefree decomposing the denominator and partial fraction expanding, we reduce to integrating a / d k ∈ q ( x), where deg a < deg d k, and where d is squarefree, so gcd ( d, d ′) = 1. 1.if the degree of the numerator is greater than or equal to that of the denominator perform long division. F ( u) f ( v) \frac { f ( u ) } { f ( v ) } f (v)f (u).