Where stands for nth differential coefficient of u and stands for nth integral of v. Then the integration of 5 x 4 with respect to x is x 5. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;
Basic Integration Rules A Freshman's Guide to Integration
111 33 ( ) ( ) ( ) (( )() 23 28 5 11 3 55 33 1 5 cos cos sin sin 8 sin 1 x x dx u du u = = = − ∫∫ integration by parts :
Sum and difference rule [ ]u v u v dx d ± = ±′ 3.
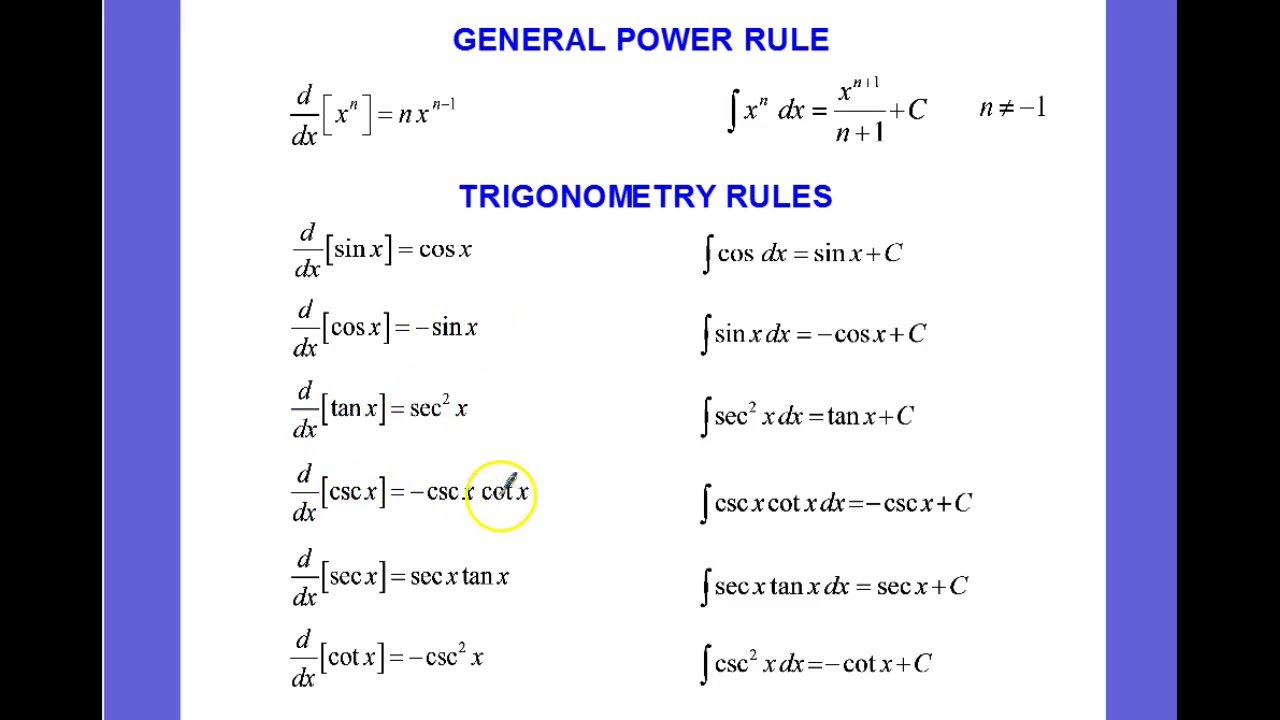
Theorem let f(x) be a continuous function on the interval [a,b]. ∫ 1 dx = x + c. If n= 1 exponential functions with base a: Du u c 1 1 n udu cn u n ln du uc u edu e cuu 1 ln adu a cuu a sin cosudu u c cos sinudu u c sec tan2 udu u c csc cot2 uuc csc cot cscuudu uc sec tan secuudu uc 22 1 arctan du u c au a a 22 arcsin du u c au a
Apr 11 6:06 pm (6 of 15) title:
∫ sec 2 x dx = tan x + c. Most of the following integral entries are written for indefinite integrals, but they also apply to. Apr 11 5:59 pm (1 of 15) title: These integrals are also called improper integrals or indefinite integrals
Now use the rules for adding:
Constant rule, [ ]c =0 dx d 6. Integration is the reverse process of differentiation. We use this in symbol as follows: The fundamental theorem of calculus.
Z ax dx= ax ln(a) + c with base e, this becomes:
Xn+1 n+ 1 + c; Constant multiple rule [ ]cu cu dx d = ′, where c is a constant. Choose from u and dv ∫ ∫udv uv vdu= − and bb b aa a ∫∫udv uv vdu= −.
Power rule [ ]x =1 dx d 8.
∫ cos x dx = sin x + c. Answer will be negative (+5) !×! Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z By systematically applying its extensive, coherent collection of symbolic integration rules, rubi is able to find the optimal antiderivative of large classes of mathematical expressions.
If n6= 1 lnjxj+ c;
∫ sec x (tan x) dx = sec x + c. ∫ a dx = ax+ c. Indefinite integrals (these rules all. Apr 11 6:05 pm (4 of 15) title:
23 ( ) 2 1 ∫ 5 cosx x dx 3 22 1 u x du x dx x dx du=⇒= ⇒ =3 3 8 xu xu=⇒== =⇒==822:
Rules and methods for integration math 121 calculus ii spring 2015 we’ve covered the most important rules and methods for integration already. Apr 11 6:03 pm (2 of 15) title: Therefore for the above examples, we usually write. ∫ x n dx = ( (x n+1 )/ (n+1))+c ;
For indefinite integrals drop the limits of integration.
Apr 11 6:05 pm (5 of 15) title: For the following, a, b, c, and c are constants ; ∫(f + g) dx = ∫f dx + ∫g dx. Derivative involving absolute value [ ]= ( )u′,u ≠0 u u u dx d 9.
Product rule [ ]uv uv vu dx d = +′ 4.
Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>: Excel integration to calculate position from velocity. This page lists some of the most common antiderivatives Now we can move on to the position data.
Suppose the derivative of x 5 is 5x 4.
For the following, the letters a, b, n , and c represent constants. Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + Apr 11 6:04 pm (3 of 15) title: 3 2;cos2 ax (65) z.
The rules only apply when the integrals exist.
Apr 11 6:07 pm (7 of 15). Basic integration formulas and the substitution rule 1the second fundamental theorem of integral calculus recall fromthe last lecture the second fundamental theorem ofintegral calculus. Power rule [ ] u nu u dx d =n −1 ′ 7. We will enter the same formula for trapezoidal area under the velocity curve to calculate position:
0 d c dx 6.
This opens the door for issuers of all sizes to have more frequent and more targeted offerings. For definite integrals, these represent real number constants. Integration is the basic operation in integral calculus.while differentiation has straightforward rules by which the derivative of a complicated function can be found by differentiating its simpler component functions, integration does not, so tables of known integrals are often useful. General integration deflnitions and methods:
Quotient rule v2 vu uv v u dx d ′− ′ = 5.