`int x tanx sec^(2)x dx` Evaluate the integral of sec x tan x + sec 2 x. X + c] as we know that by definition integration is the inverse process of the derivative, the integral sign ∫ and d d x on the right side will cancel each other out, i.e.
What is the integration of tanx? Quora
This just cancels in the end to 0 = 0.
So, let u = ln (sec x + tan x) du = sec x * [sec x + tan x] / [sec x + tan x] dx.
Please remember that the computed indefinite integral belongs to a. (du)/dx=sec^2(x)dx let's turn this derivative into a differential by multiplying both sides by dx: Starting early can help you score better! The second integral, upon multiplying both the numerator and denominator by (sec x + tan x), evaluates to log |sec x + tan x|+ c.
Integrating both sides of equation (i) with respect to x, we have.
What is the integration of {1 + 2tanx (tanx + secx)} ^1/2 dx?* ***** i=\displaystyle \int (1 + 2\tan x (\tan x + \sec x)) ^{1/2}dx =\displaystyle. Avail 25% off on study pack. X d x = ∫ d [ sec. Integral of sec^2 (x) \square!
The first one is simple, but what is the integral of.
It also allows to draw graphs of the function and its integral. Your first 5 questions are on us! $\displaystyle \int{\sec^2{x} \,}dx \,=\, \tan{x}+c$ alternative forms. We can prove that the integral of sec x to be ln | tan [ (x/2) + (π/4) ] | + c by using trigonometric formulas.
= ∫ [(sinx ∙ cosx)/ (1 + sinx + cosx)]dx.
You should be able to see the integral you want on both sides of the equation now, so. Integral of sec (x)^2 by x: I've tried a few things, the first being integration by parts with u = ln (secx + tanx+) and dv = dx. >> integrals >> integration by substitution >> evaluate inttanx/(secx + tanx) dx.
∫(sec x tan x + sec 2 x) dx = ∫sec x tan x dx + ∫sec 2 x dx = sec x + tan x + c
We can write sec x as 1/ (cos x) where cos x can again be written as sin (x + π/2). (u\cdot v)'=u\cdot v'+u'\cdot v\tag*{} integrate both sides and rearran. = ∫ [{1 (sinx ∙ cosx)}/ (sin2x + sinx + cos2x + cosx)]dx. About press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators.
Also, the integral of a sum of two functions is equal to the sum of integrals of the two functions.
Got me thinking how the product rule and integration by parts are related. Find all solutions for this book. I = ∫ [1/ (tanx + cotx + secx + cosecx)] ∙ dx. \[\frac{1}{2}\] log (sec x 2 + tan x 2) + c \[\text{ let i }= \int x \sec x^2 dx\] \[\text{ putting x}^2 = t\] \[ \rightarrow 2x \text{ dx }= dt\] \[ \rightarrow x.
The integration of secant squared of angle $x$ function with respect to $x$ is equal to sum of the tan of angle $x$ and the constant of integration.
Evaluate ∫ (sec x + tan x) tan x d x. = ∫ [1/ ( { (sinx + 1)/ (cosx)} + { (cosx + 1)/ (sinx)})]dx. Here is the product rule: The first requires integration by parts.
= ∫ 1/ (sin (x + π/2)) dx.
Sec x = sec x * [ (sec x + tan x) / (sec x + tan x)] = g(x) ln (sec x + tan x) = integral of g(x)dx. Integrating u du = u^2/2 = [ln (sec x + tan x)]^2 / 2 + c Integral of tan(x)*sec^2(x), two results off by a constant, integral of tan(x)*sec^2(x) by substitution, integral of tan(x)*sec^2(x) by u sub, calculus 2 int. We know that the integration of sec x tan x is sec x + c and the integral of sec 2 x is tan x + c.
∫ (a tan x + b cot x) 2 d x.
Tan (x) draw graph edit expression direct link to this page. Du=sec^2(x)dx we see du appears in our integral;
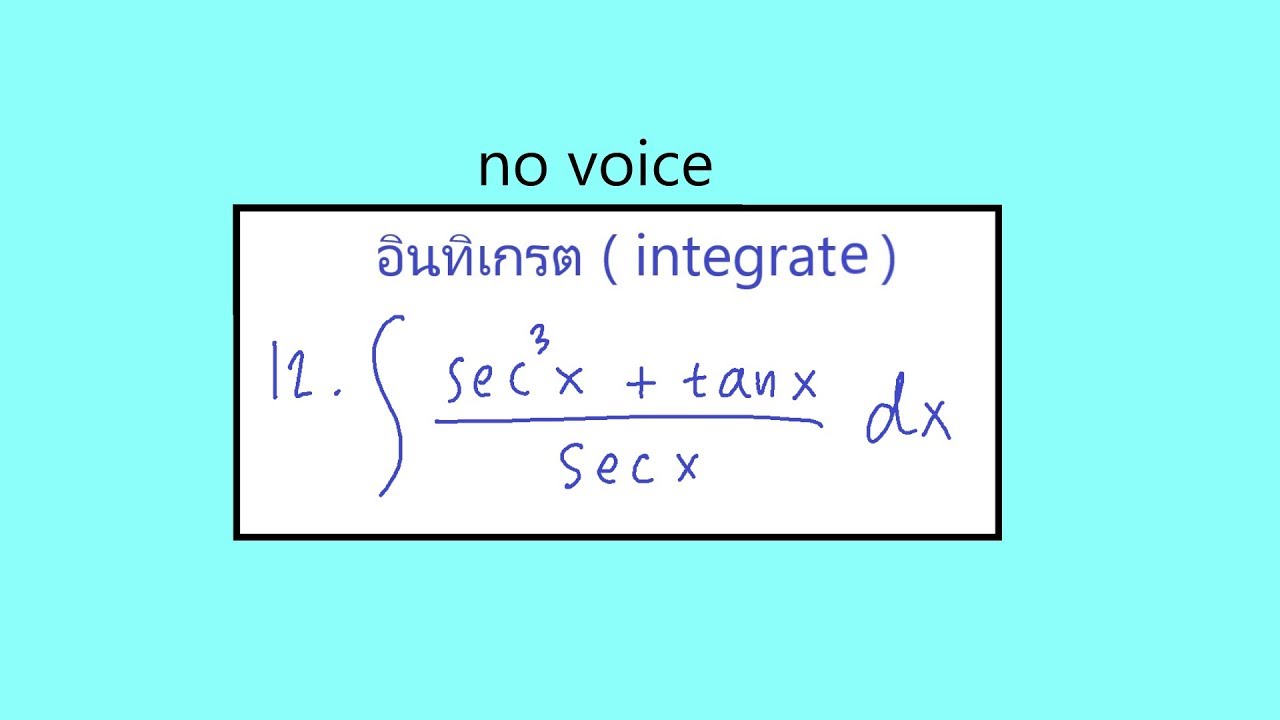


![`inte^(x)[secx+log(secx+tanx)]dx=?` YouTube](https://i.ytimg.com/vi/GfW7ul7uE-A/maxresdefault.jpg)