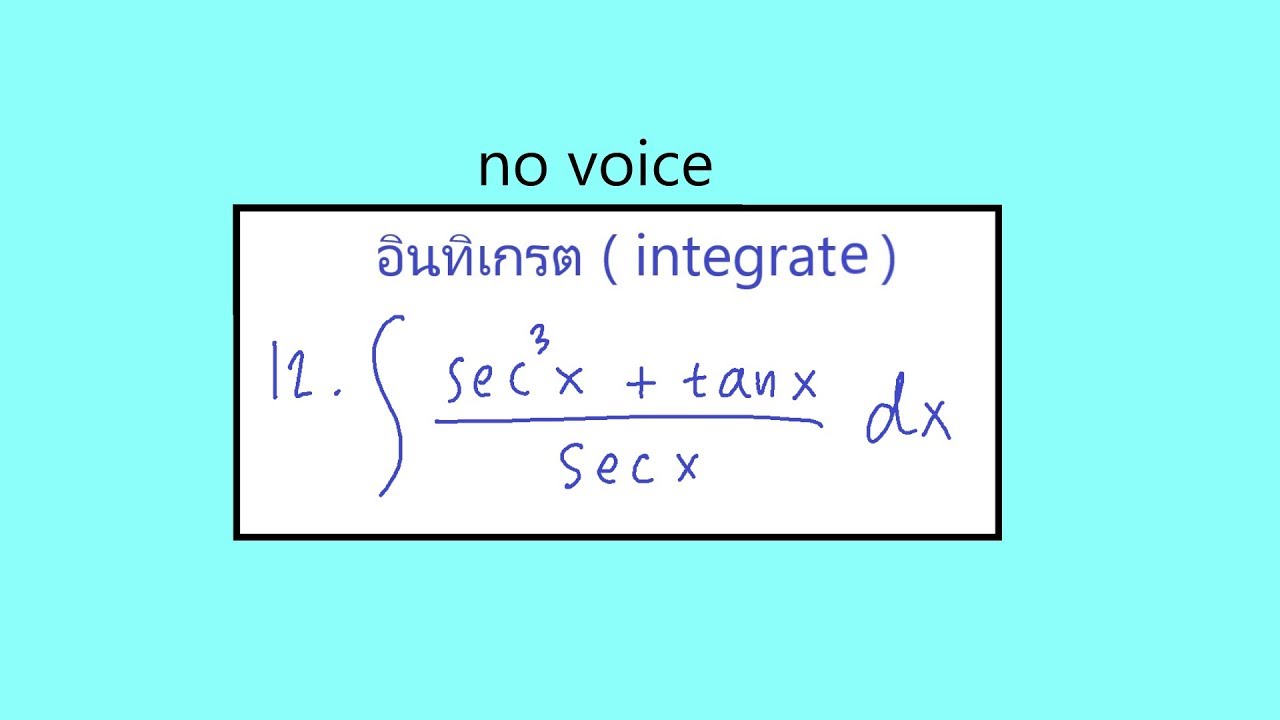
The integration of sec x tan x is sec x + c, where c is the integration constant. Differentiate sec ( x) sec ( x). E − x) = − sec x.
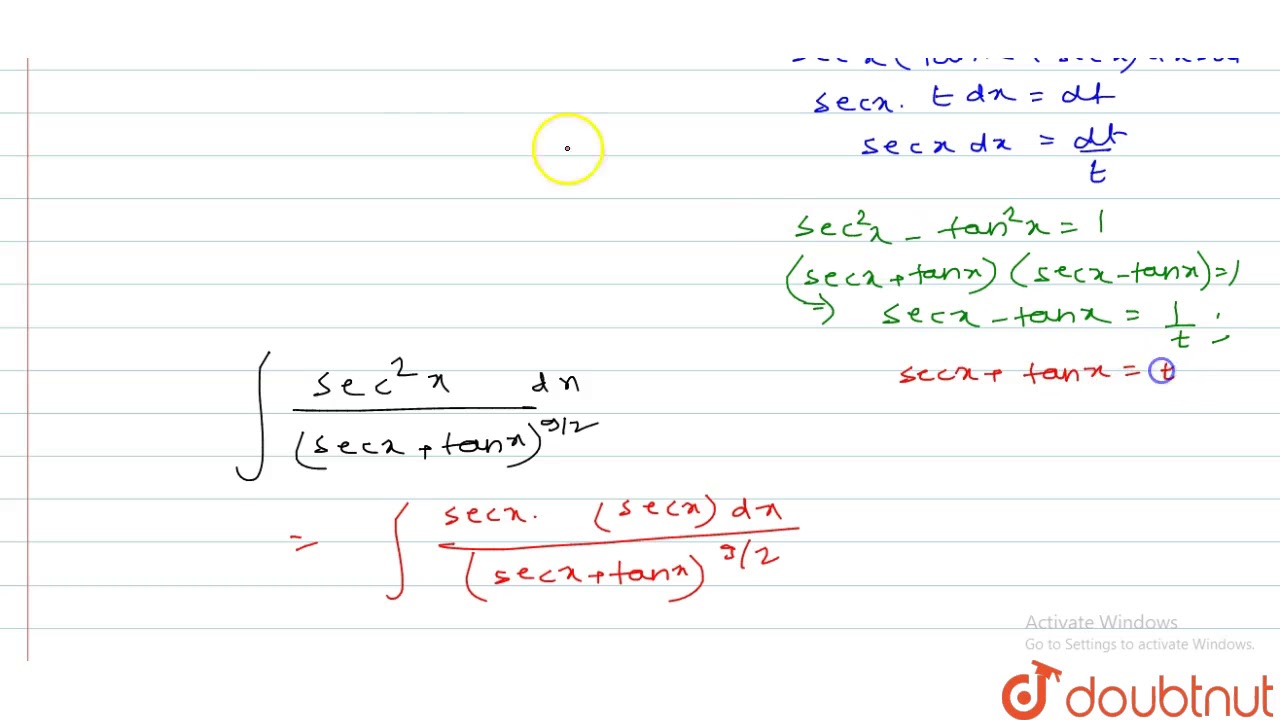
IIT JEE 2012 Integral, Integral of sec^2/(secx+tanx^(9/2
According to integral calculus, it can be expressed in mathematical form as follows.
Misc 33 important → chapter 7 class 12 integrals (term 2) serial order wise;
Kindly sign up for a personalised experience. ∫ e − x (sec x − sec x tan x) = sec x ∫ e − x − ∫ (sec x tan x ∫ e − x) − ∫ sec x tan x. Integration of the secant tangent function is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae. Integrating both sides of equation (i) with respect to x, we have.
Therefore, ∫tanxsecxdx = ∫du = u.
I = ∫ [1/ (tanx + cotx + secx + cosecx)] ∙ dx. E − x = − sec x. \[\int \tan^{2}x\sec{x} \, dx\] +. Left part is to int.
∫ 1 + tan x 1 − tan x d x =.
Queries asked on sunday & after 7pm from monday to saturday will be answered after 12pm the next working day. X function is called as the integration of product of secant and tan functions. Why is the integration of secx tanx important? E − x + ∫ (sec x tan x.
It denoted by ∫ sec x dx.
Answered by | 19th sep, 2013, 10:03: Hendikeps2 and 62 more users found this answer helpful. E − x) − ∫ (sec x tan x. The derivative of sec ( x) sec ( x) with respect to x x is sec ( x) tan ( x) sec ( x) tan ( x).
Answered apr 28, 2018 by rubby (52.4k points) selected may 27, 2018 by vikash kumar.
= (sec 2 x + sec x + tan x )dx. ∫{sec^2 x / [(sec x + tan x)^9/2]} dx = (for some arbitrary constant c) Du = ( 1 cosx)' = − 1 cos2x ⋅ − sinxdx = sinx cos2x dx. We observe that du appears in the numerator of the integral, so we may apply the substitution:
Du= (sec x + tan x + sec 2 x) dx.
Multiply secx in bracket =int. Here ln stands for natural logarithm and 'c' is the integration constant. ∫ d u u = ln | u | + c. Evaluate ∫ sec x + tan x sec x d x.
We have multiple formulas for this.
We know that the derivative of the trigonometric function sec x is sec x tan x, i.e., d (sec x)/dx = sec x tan x. So, if we integrate both sides of this equation we have ∫d (sec x)/dx dx =. Now will substitute u with x that is u = (sec x + tan x) we get, ∫ s e c x d x = ln | s e c x + t a n x | + c. But the more popular formula is, ∫ sec x dx = ln |sec x + tan x| + c.
The collection of all primitives of product of sec.
I = (u2/2) + c. The integral of sec x is ln|sec x + tan x| + c. Sudhakar sharma, added an answer, on 23/11/11. Let i = \(\int\) (tan x) dx.
= ∫ [1/ ( { (sinx + 1)/ (cosx)} + { (cosx + 1)/ (sinx)})]dx.
Int secx.tanx +int tan 2 x. Rewrite the problem using u u and d u d u. X + c] as we know that by definition integration is the inverse process of the derivative, the integral sign ∫ and d d x on the right side will cancel each other out, i.e. X d x = ∫ d [ sec.
Ex 7.1, 18 find anti derivative of ∫1 〖sec𝑥 (sec〖𝑥+tan𝑥 〗)〗dx ∫1 〖𝑠𝑒𝑐𝑥 (𝑠𝑒𝑐〖𝑥+𝑡𝑎𝑛𝑥 〗)〗 𝑑𝑥 =∫1 〖 (〖𝑠𝑒𝑐〗^2〖𝑥+〖𝑠𝑒𝑐 𝑥 𝑡𝑎𝑛〗𝑥 〗)〗 𝑑𝑥 =∫1 〖〖𝑠𝑒𝑐〗^2 𝑥 𝑑𝑥+ 〗 ∫1 (𝑠𝑒𝑐 𝑥 𝑡𝑎𝑛𝑥 ) 𝑑𝑥.
= ∫ [{1 (sinx ∙ cosx)}/ (sin2x + sinx + cos2x + cosx)]dx. By the power rule, the integral of u3 u 3 with respect to u u is 1 4u4. This is also known as the antiderivative of sec x. Then, i = \(\int\) \(sin x\over cos x\) dx.
Du/dx = {1/ (secx+tanx)} * { (secx*tanx)+ (sec2x)} du/dx = {1/ (secx+tanx)} * { (tanx)+ (secx)} * secx.
= ∫ [(sinx ∙ cosx)/ (1 + sinx + cosx)]dx. Please log in or register to add a.