∫ cosxdx 1 +sinx 1 + sinx = u cosxdx = du. According to integral calculus, it can be expressed in mathematical form as follows. ∫(sec x tan x + sec 2 x) dx = ∫sec x tan x dx + ∫sec 2 x dx = sec x + tan x + c
壮大 Tanx2 Integral 画像コレクション
𝑑𝑡/2𝑥 i1 = 1/2 ∫1 1/𝑡.
Davneet singh is a graduate from indian institute of technology, kanpur.
Rewrite in terms of sines and cosines. Multiply by the reciprocal of the fraction to divide by. ∫ 1 secx + tanx dx = dx 1 cosx + sinx cosx. Therefore, ∫tanxsecxdx = ∫du = u.
X function and the constant of integration ( c ).
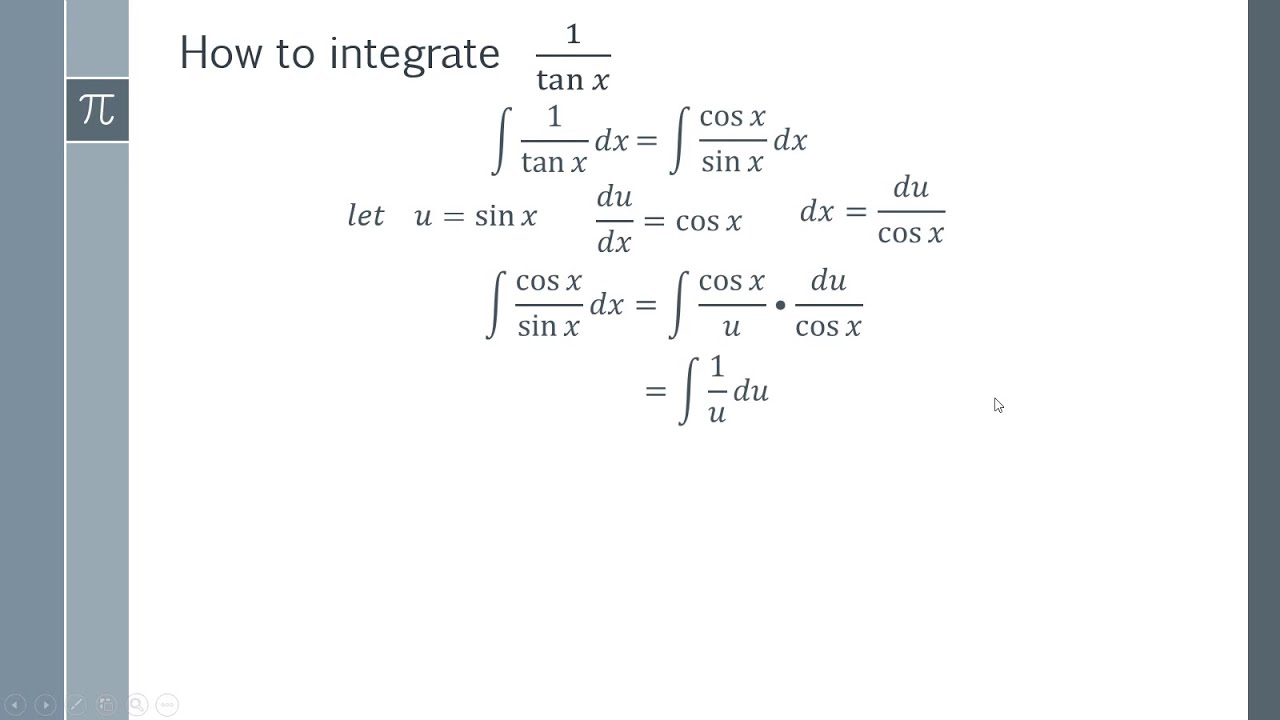
As we know that by definition integration is the inverse process of the derivative, the integral sign and on the right side will cancel each other out, i.e. (1) 1 (2) 1/2 (3) cos x (4) sec x. ← prev question next question →. ∫ 1 secx + tanx dx = ln(1 +sinx) +c.
(sec x + tan x )dx.
Answered apr 28, 2018 by rubby (52.4k points) selected may 27, 2018 by vikash kumar. He provides courses for maths and science at teachoo. Multiply by the reciprocal of the fraction to divide by. Given,y=tan −1(secx+tanx)⇒y=tan −1[tan( 4π + 2x )][∵tan( 4π + 2x )=secx+tanx]⇒y= 4π + 2x on differentiating w.r.t x we getdxdy =0+ 21 = 21.
Let u=log (secx+tanx) differentiate wrt x.
Integration of the secant tangent function is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae. Therefore, it has proved that the integration of product of secant and tan functions with respect to a variable is equal to the sum of the secant function and integral constant. And its distance from the earth is. Make in terms of sin's and cos's;
Please log in or register to add a comment.
Evaluate integral of 1/(sec(x)tan(x)) with respect to x. Integration of the secant tangent function is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae. Also, the integral of a sum of two functions is equal to the sum of integrals of the two functions. Homework statement ∫[(1+tanx.tan(x+a)]dx homework equations the attempt at a solution ∫sec^x.tan(x+a) after that i don't know as i tried method of substitution by putting x+a=t but i am not getting the answer as its form on the whole remains same
X d x = sec.
Evaluate the integral of sec x tan x + sec 2 x. The diameter of the moon is. Secx+tanx= cosx1 + cosxsinx = cosx1+sinx = cos 22x −sin 22x 1+2sin 2x cos 2x (∵sin2a=2sinacosa & cos2a=cos 2a−sin 2a)= cos 22x −sin 22x sin 22x +cos 22x +2sin 2x cos. Du = ( 1 cosx)' = − 1 cos2x ⋅ − sinxdx = sinx cos2x dx.
Du/dx = {1/ (secx+tanx)} * { d (secx)/dx + d (tanx)/dx} du/dx = {1/ (secx+tanx)} * { (secx*tanx)+ (sec2x)} du/dx = {1/ (secx+tanx)} * {.
∫tan⁻1 (secx+tanx) =∫∫tan⁻1 (1/cosx+sinx/cosx) search. 𝑑𝑡 i1 = 1/2 log〖 |𝑡|〗+𝐶1 i1 = 1/2 log〖 |1+𝑥^2 |〗+𝐶1 putting the value of i1 in (1) , ∫1 〖 tan^(−1) 𝑥 〗.𝑑𝑥=𝑥 tan^(−1) 𝑥−∫1 𝑥/(1 + 𝑥^2 ). Integral de x sec x tan x dx por partes. Follow as i have solved.
The integration of secant tangent is of the form.
We know that the integration of sec x tan x is sec x + c and the integral of sec 2 x is tan x + c. ∫ 1 secx + tanx dx = lnu + c. Musashixjubeio0 and 55 more users found this answer helpful.


![`inte^(x)[secx+log(secx+tanx)]dx=?` YouTube](https://i.ytimg.com/vi/GfW7ul7uE-A/maxresdefault.jpg)