The answer is =2sqrt(tanx)+c we need tanx=sinx/cosx sinx=cosxtanx=tanx/secx therefore, the integral is i=int(sqrt(tanx)dx)/(sinxcosx)=int(sqrt(tanx)dx)/(tanx/secx*1/secx) =int(sec^2xdx)/sqrt(tanx) let u=tanx, =>, du=sec^2xdx the integral is i=int(du)sqrt(u) =sqrt(u)/(1/2) =2sqrt(u) =2sqrt(tanx)+c We had the exact same question for our final board exams in india. Let , then we have.
What is ∫ (SQRT (tanx) +SQRT (cotx)) dx? Quora
This intimidating integration of square root of tanx can be solved by distinct techniques.
As djc told you, you may write 1+tan2x using a common denominator as cos2x+sin2x cos2x = 1 cos2x so the square root of it is simply cosx.
Sec 2 x d x = 2 t d t. Ex 7.2, 34 integrate √ (tan𝑥 )/sin〖𝑥 cos𝑥 〗 simplifying the function √ (tan𝑥 )/sin〖𝑥 cos𝑥 〗 = √ (tan𝑥 )/ (sin〖𝑥 cos𝑥 〗. Integrate wrt xsin 3xcos 3x. L e t tan x = t 2.
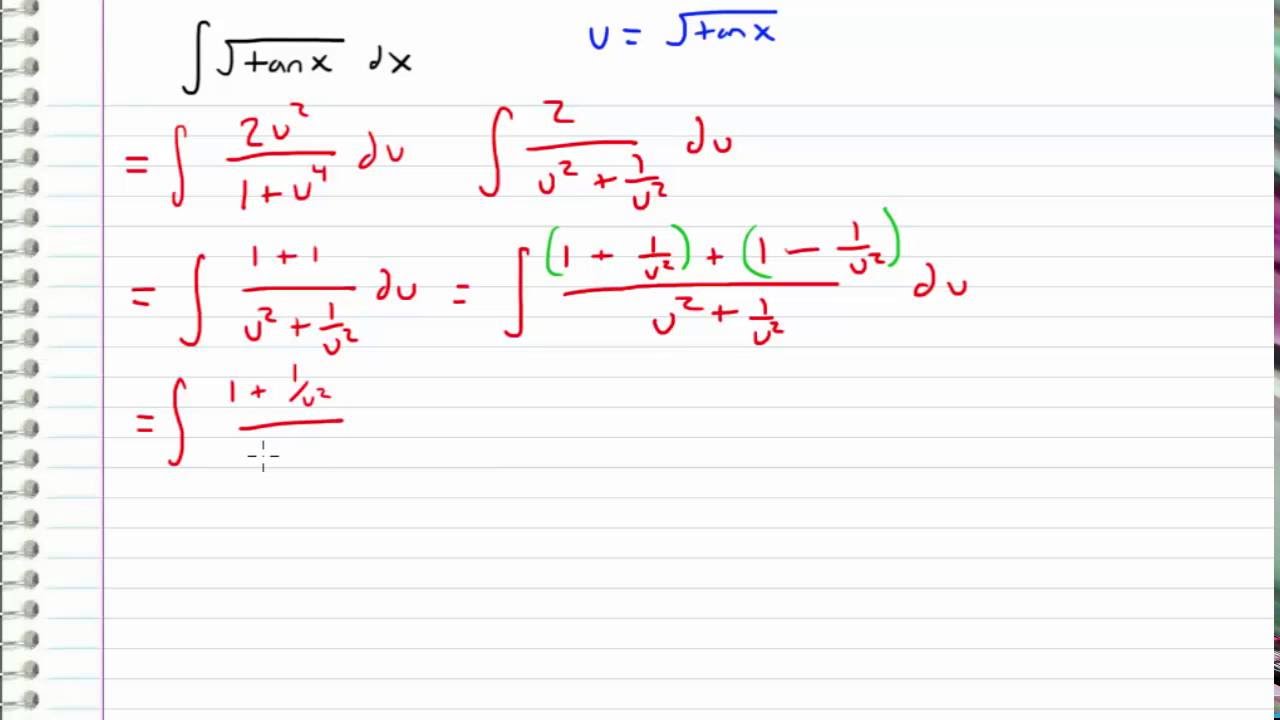
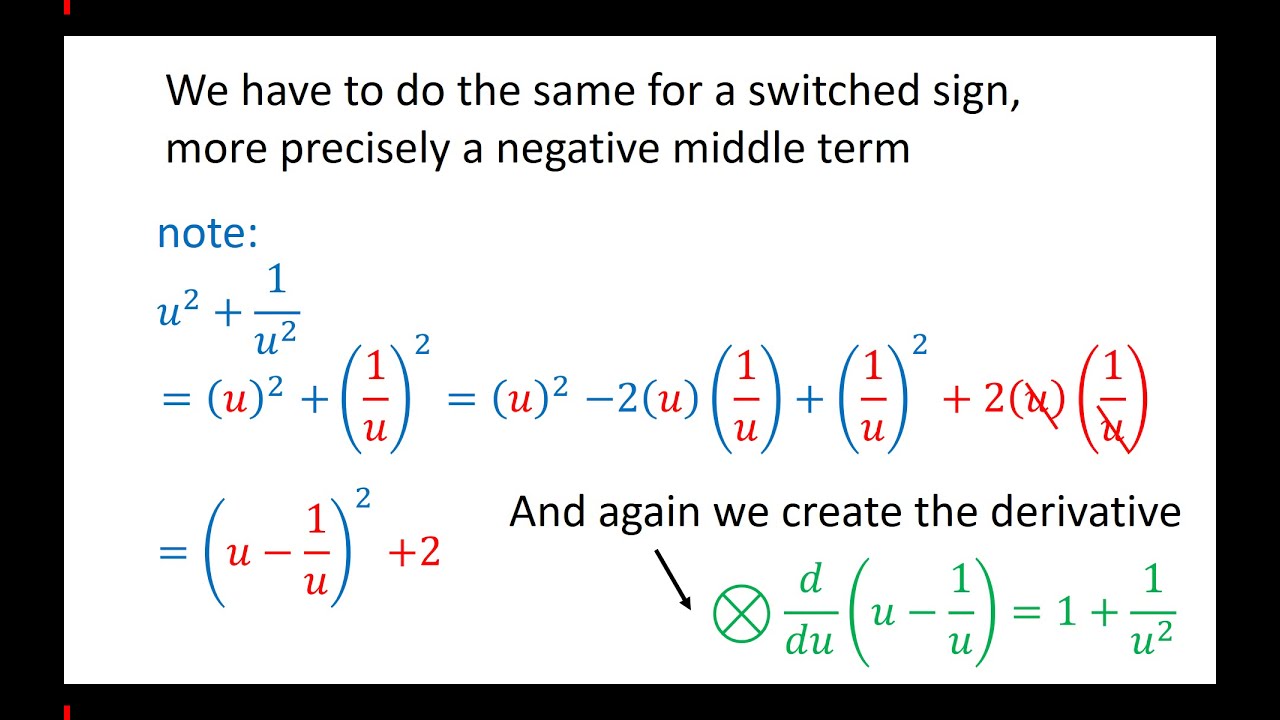
Let $u = \sqrt{\tan x}$, then $u^2 = \tan x$.
Thus $\mathrm{d}x = \dfrac{2u\;\mathrm{d}u}{u^4 + 1}$. Find integral of root tan x. I = ∫ ( cot x + tan x) d x. See the image for solution thanks
Evaluate integral of (sec (x)^2)/ ( square root of tan (x)) with respect to x.
If you want to see a more elegant and symmetric solution, you can skip the rest and go to the remark section at the end. The integral of cot (x) dx is ln (absolute value (sin (x))) + c. There are two methods to deal with 𝑡𝑎𝑛𝑥 (1) convert into 𝑠𝑖𝑛𝑥 and 𝑐𝑜𝑠𝑥 ,. The first method i will describe is the mostelegant, but requires.
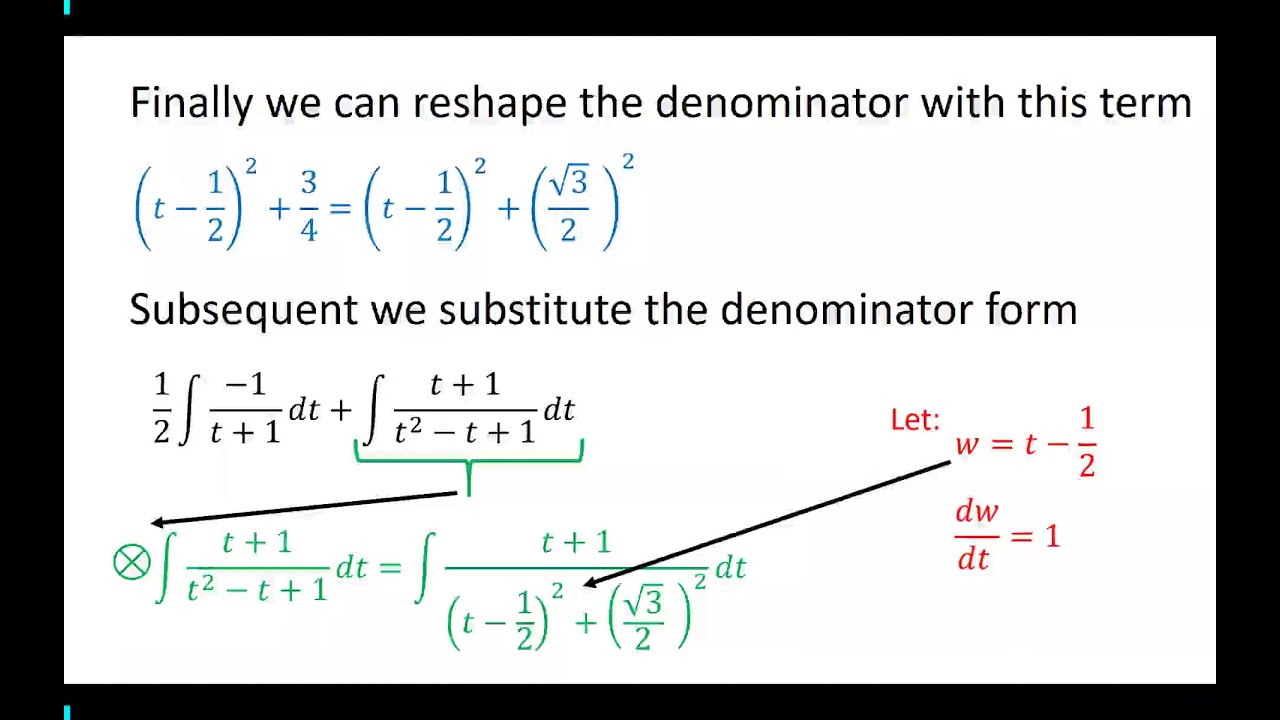
Let tan x = t 2.
Rewrite using u u and d d u u. Please 'like' if you enjoy and 'subscribe' if. Let , or , hence. $$\int\sqrt{\tan x}\;\mathrm{d}x = \int\frac{2u^2}{u^4+1}\;\mathrm{d}u$$ you.
Tanx=t^2, so that, sec^2xdx=2tdt, or, dx=(2tdt)/sec^2x=(2tdt)/(1+tan^2x)=(2tdt)/(1+t^4).
Without using the absolute value, you can use the square root of the square, i.e. Thus $2u\;\mathrm{d}u = \sec^2 x\;\mathrm{d}x = (u^4 + 1)\mathrm{d}x$. U^1/2 du.but you haven't changed dx to du.you can do this as. Cos^2𝑥/cos𝑥 ) = √ (tan𝑥 )/ (cos^2𝑥.
So the goal is to integral 1/cosx from 0 to π/4.
Stack exchange network stack exchange network consists of 179 q&a communities including stack overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. Now, i will show you how every student (specially in north america) will do next. Just follow orion's thread to see how it is done. ∫ 1 + x 6 x 2 tan − 1 ( x 3) d x.
How to write the integral of 1 + tan2x?
⇒ integral ∫ 2t 2 / (1 + t 4) dt. The integral of the square root of tangent x. In this video, the definite integral of sqrt (tan (x)) from 0 to pi/2 is evaluated using the beta function, gamma function, and euler's reflection formula. ∴ i = ∫ t ( 1 + 1 t 2) × 2 t 1 + t 4 d t.
= 2 ∫ t 2 + 1 t 4 + 1 d t.
And then find du and so the integrand changes. Let u = tan(x) u = tan ( x). ⇒ sec 2 x dx = 2t dt. Well hoot, what you have done is considered tan (x)=u and integrated.
Up to 3% cash back integral of the square root of tan (x) from 0 to pi/2.
$\int \sqrt{\tan (x)}dx $ let $\tan(x)=t^{2}$ then $dx$ will become $\frac{2t}{1+t^{4}}$ hence $\int \sqrt{\tan (x)}dx =\int\frac{2t}{1+t^4} dt. Example 42 important → chapter 7 class 12 integrals (term 2) serial order wise; Example 1 (i) example 1 (ii) example 1 (iii) example 2 (i). ⇒ d x = 2 t d t 1 + t 4.
⇒ dx = [2t / (1 + t 4 )]dt.
Then du = sec2(x)dx d u = sec 2 ( x) d x, so 1 sec2 (x) du = dx 1 sec 2 ( x) d u = d x. 1) (1 / 2 √tan x) 2) √(2 tan x) 3) 2 √(tan x) 4) √tan x. Cos𝑥/cos𝑥 ) = √ (tan𝑥 )/ (sin𝑥. Ln (square root (sin2x)) + c