Inform you about time table of exam. ⇒ 2 i = ∫ 0 2 π log. In all such cases , ,the answer will.
integration of sec x /log (sec x + tan x) dx Brainly.in
A unique platform where students can interact with teachers/experts/students to get solutions to their queries.
Log(secx + tanx) = t.
Sec x + tan x. Multiply secx in bracket =int. ( 1 + tan 2. Why create a profile on shaalaa.com?
Substitute du = (sec x tan x + sec 2 x) dx, u = sec x + tan x.
X) ⇒ 2 i = 0. Integral of (sec (x)tan (x))/ (1+sec (x)) \square! X function and the constant of integration ( c ). Integrating the secant requires a bit of manipulation.
Let u= log(secx+tanx) differentiate wrt x du/dx = {1/ (secx+tanx)} * { d(secx)/dx + d(tanx)/dx} du/dx = {1/ (secx+tanx)} * { (secx*tanx)+(sec 2 x)}.
Evaluate integral of natural log of sec (x)+tan (x) with respect to x. The first one is simple, but what is the integral of. The integration of secant tangent is of the form \[\int {\sec x\tan xdx = } \sec x + c\] to prove this formula, consider \[\frac{d}{{dx}}\left[ {\sec x + c} \right] = \frac{d}{{dx}}\sec x + \frac{d}{{dx}}c\] using the derivative formula $$\frac{d}{{dx}}\sec x = \sec x\tan x$$, we have Your first 5 questions are on us!
According to integral calculus, it can be expressed in mathematical form as follows.
Combine 1 sec(x)+tan(x) 1 sec ( x) + tan ( x) and x x. Solve this problem a block rests on a rough inclined plane making an angle of 30o with the horizontal. Click here👆to get an answer to your question ️ intsin x log (sec x + tan x)dx = f(x) + x + c then f(x) = Ex 7.1,1 ex 7.1, 2 ex 7.1, 3 ex 7.1, 4 ex 7.1, 5 ex 7.1, 6.
Therefore, it has proved that the integration of product of secant and tan functions with respect to a variable is equal to the sum of the secant function and integral constant.
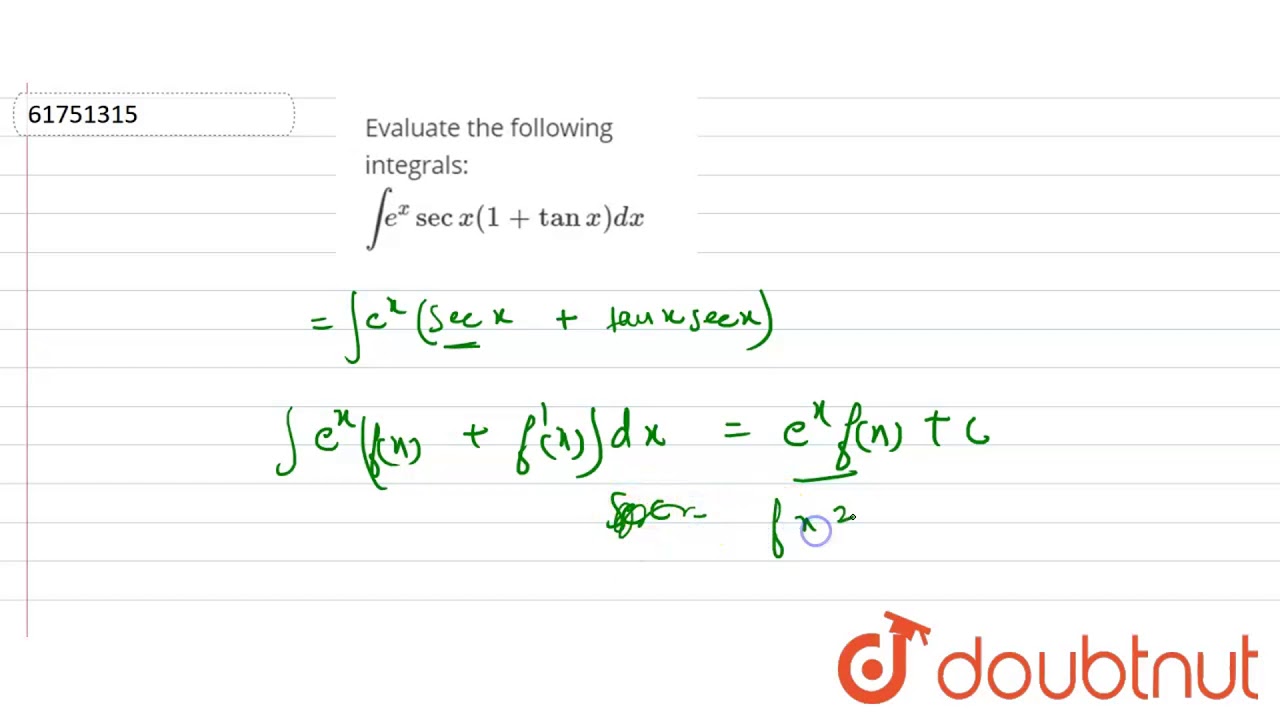
Solve the problem in photo showing each step clearly. This just cancels in the end to 0 = 0. I've tried a few things, the first being integration by parts with u = ln (secx + tanx+) and dv = dx. Here , i = ∫e x ( tan x + log secx ) dx = ∫e x (log secx + tan x )dx.
But, t = log(secx + tanx) = ln |log(secx + tanx)| + c.
Let u = sec(x)+tan(x) u = sec ( x) + tan ( x). D(log(secx + tanx)) = dt (use chain rule to differentiate) first differentiate, log(secx + tanx) then, (secx + tanx) ⇒ secx dx = dt. Students (upto class 10+2) preparing for all government exams, cbse board exam, icse board exam, state board exam, jee (mains+advance) and neet can ask questions from any subject and get quick answers by. The coefficient of static friction between the block and the plane is 0.8.
Be e x f (x) + c.
This is a question of the type ∫ ( f (x) + f’ (x) )e x dx. Put t and dt in the given equation we get, ⇒ ∫ \(\frac{dt}{t}\) =ln |t| + c. U = sec x + tan x. Sec x dx = sec x.
Ex 7.1, 19 → chapter 7 class 12 integrals (term 2) serial order wise;
Editor toolbars basic styles bold paragraph insert/remove numbered list insert/remove bulleted list insert image insert horizontal. Inform you about new question papers. Multiply secx by secx +tanx secx +tanx, which is really the same as multiplying by 1. Du = (secxtanx +sec2x)dx = (sec2x + secxtanx)dx.
∫ sec2x + secxtanx secx + tanx dx.
∫( secx(secx +tanx) secx +tanx)dx. Sec x + tan x. I = ⌡ secx*log(secx+tanx) dx.