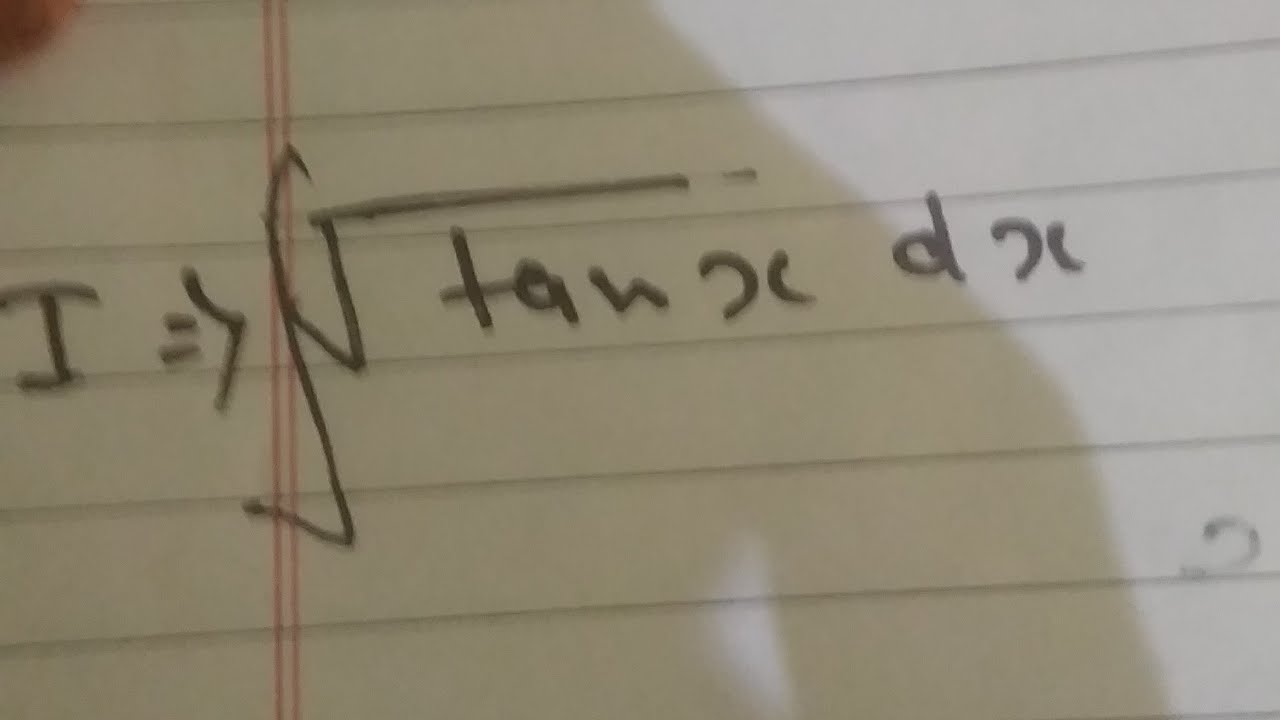Note that, for the integral you already did, you can assume tan(x) = u2 to get. ⇒ sec 2 x dx = 2t dt. The answer is =2sqrt(tanx)+c we need tanx=sinx/cosx sinx=cosxtanx=tanx/secx therefore, the integral is i=int(sqrt(tanx)dx)/(sinxcosx)=int(sqrt(tanx)dx)/(tanx/secx*1/secx) =int(sec^2xdx)/sqrt(tanx) let u=tanx, =>, du=sec^2xdx the integral is i=int(du)sqrt(u) =sqrt(u)/(1/2) =2sqrt(u) =2sqrt(tanx)+c
Tutorial How to solve the Integral of sqrt(tanx), IIT JEE
= 2 ∫ 1 + 1 t 2 t 2 + 1 t 2 d t.
For more about how to use the integral calculator, go to help or take a look at the examples.
I = ∫ ( cot x + tan x) d x. Let tan x = t 2. ⇒ integral ∫ 2t 2 / (1 + t 4) dt. ∫(tan(x))1 / 4dx = 4∫ u4 u8 + 1du.
⇒ d x = 2 t d t 1 + t 4.
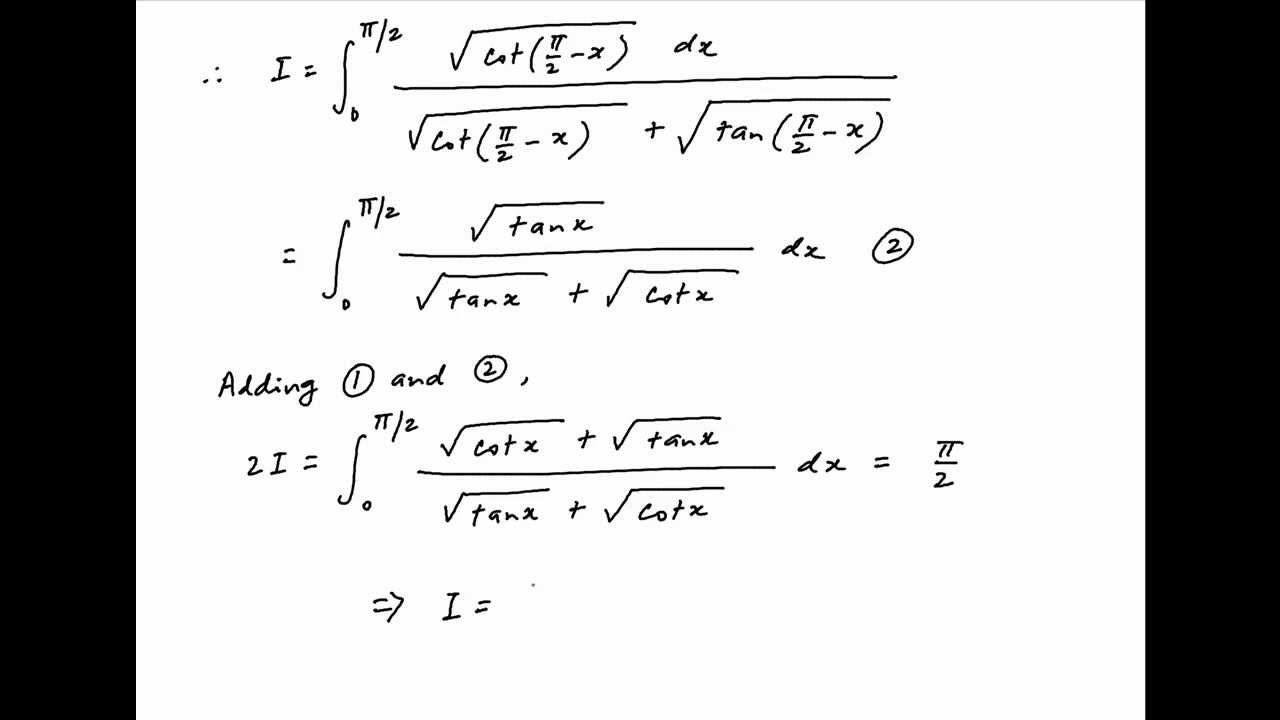
∫[(√tan x) / (sin x cos x)] dx =. Interactive graphs/plots help visualize and better understand the functions. ∴ i = ∫ t ( 1 + 1 t 2) × 2 t 1 + t 4 d t. L e t i = ∫ 0 π 4 ( tan.
Example 1 (i) example 1 (ii) example 1 (iii) example 2 (i).
= ∫ ( tan x ( 1 + cot x)) d x. Let tan x = t 2. Click here👆to get an answer to your question ️ int^ (√(tanx)+√(cotx))dx = = 2 ∫ t 2 + 1 t 4 + 1 d t.
There are two methods to deal with 𝑡𝑎𝑛𝑥 (1) convert into 𝑠𝑖𝑛𝑥 and 𝑐𝑜𝑠𝑥 ,.
And then find du and so the integrand changes. (1) putting t = 1, a + 2c + d = 1. Statement i the value of the integral ∫(x → pi/3,pi/6)dx/(1 + √tanx) is equal to pi/6. Sec 2 x d x = 2 t d t.
⇒ sec 2 x dx = 2t dt.
Sec 2 x dx = dz i.e dx = dz/(1+z 2) putting t = 0, in the above, b+d = 0. The first method i will describe is the mostelegant, but requires. ⇒ dx = [2t / (1 + t 4 )]dt. Cos^2𝑥/cos𝑥 ) = √ (tan𝑥 )/ (cos^2𝑥.
Tanx=t^2, so that, sec^2xdx=2tdt, or, dx=(2tdt)/sec^2x=(2tdt)/(1+tan^2x)=(2tdt)/(1+t^4).
You can also check your answers! (3) using (2) & (3), d = 0. Ex 7.2, 34 integrate √ (tan𝑥 )/sin〖𝑥 cos𝑥 〗 simplifying the function √ (tan𝑥 )/sin〖𝑥 cos𝑥 〗 = √ (tan𝑥 )/ (sin〖𝑥 cos𝑥 〗. Im gonna give u the solution and i hope it is the easiest, regardless of the fact that it could be a bit lengthy.
U^1/2 du.but you haven't changed dx to du.you can do this as.
Example 42 important → chapter 7 class 12 integrals (term 2) serial order wise; X ≠ kπ / 2 and tan x > 0. Cos𝑥/cos𝑥 ) = √ (tan𝑥 )/ (sin𝑥. Therefore, b = 0 using (1) a + 2c = 1.
Just follow orion's thread to see how it is done.
∫tan 1/3 x dx take tanx = z ; We had the exact same question for our final board exams in india. The integral calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables. Now, you can use some integration techniques to evaluate the integrals.
T h e n, i = ∫ 0 π 4 ( sin.
Well hoot, what you have done is considered tan (x)=u and integrated. Integral of root of tan x sin x cos x dx c x kpi 2 and tan x 0. For the other one, you can assume tan(x) = u4 to get. Integrate wrt xsin 3xcos 3x.
I hope it works out for you… thank you.
This intimidating integration of square root of tanx can be solved by distinct techniques. 1) (1 / 2 √tan x) 2) √(2 tan x). Setting $$\sqrt[5]{\tan(x)}=t$$ then we get $$x=\arctan(t^5)$$ and $$dx=5\,{\frac {{t}^{4}}{{t}^{10}+1}}dt$$ and our integral will be $$5\int \frac{t^5}{t^{10}+1}dt$$ share cite ∫ 1 + x 6 x 2 tan − 1 ( x 3) d x.
L e t tan x = t 2.
⇒ integral ∫ 2t 2 / (1 + t 4) dt.