= 1 2 ln∣∣x2 +1∣∣ +c. Evaluate the following integral using properties of integration. ∫ x x2 +1 dx.
What is the integration of x^n log x? Quora
Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi;
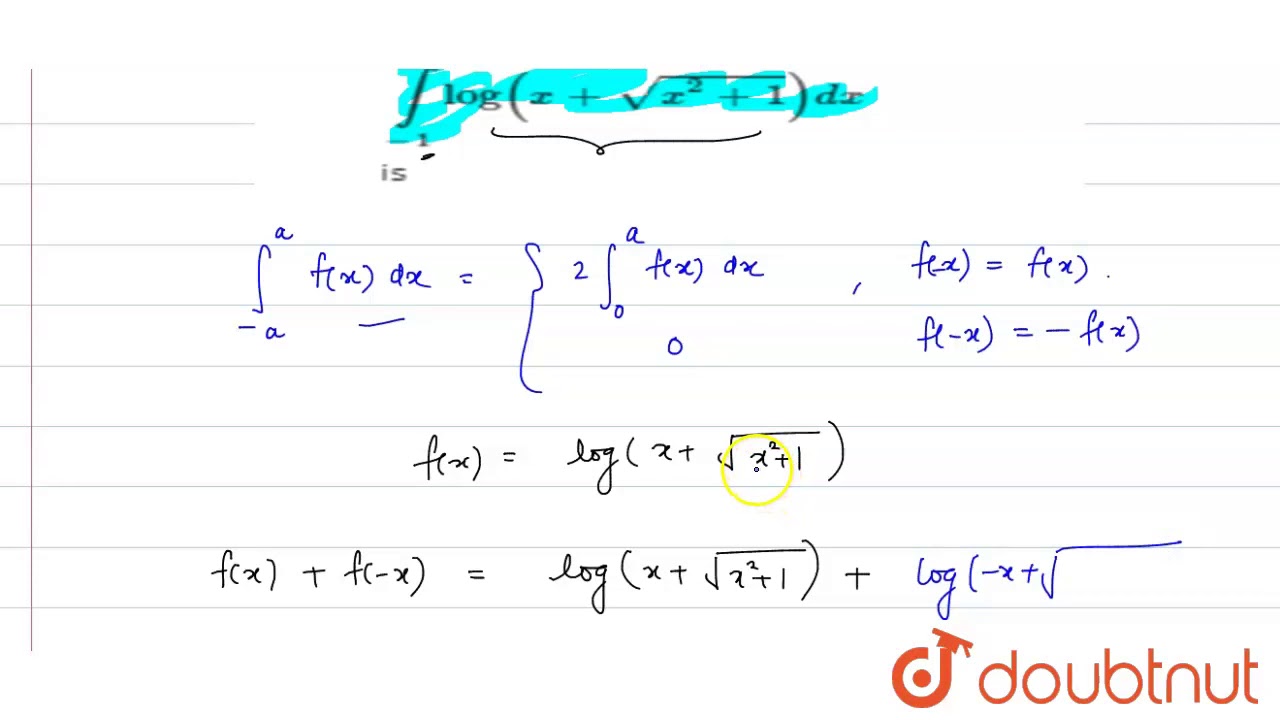
The first integral on the right is easily computed as π 5 / 80.
Integrate the functions 2x /1 + x square # ncert. Then du = cosx dx and dx = (du)/cosx. ∫ x n dx = ( (x n+1 )/ (n+1))+c ; To prove this formula, consider.
The list of basic integral formulas are.
How much interest did he pay. \(\begin{array}{l}\int \frac{x\ln \left(x\right)}{\left(1+x^2\right)^2}dx\end{array} \) \(\begin{array}{l}\frac{x\ln \left(x\right)}{\left(1+x^2\right)^2}=\left(x\ln. ∫ sec x (tan x) dx = sec x + c. ∫ 1 dx = x + c.
∫ sec 2 x dx = tan x + c.
This leaves us in good shape; This integral can be written as. Given to integrate function, let us assume. ∫ a dx = ax+ c.
( 1 − e − 2 y) d y.
When the integrand is a rational function with a quadratic expression in the denominator, we can use the following table integrals: The integral is equal to 1/3sin^3x + c let u = sinx. Answers (1) d divya prakash singh. X) d x = ∫ − π / 2 π / 2 x 4 d x − π 3 ∫ 0 ∞ log 3.
= ∫ d(u(x)) 2u(x) = 1 2 ∫ du(x) u(x) = 1 2 ln|u(x)| +c.
Find the integral of xlogx/(1+x2)2under the limits 0 to infinity. To avoid ambiguous queries, make sure to use parentheses where necessary. Will be a root the quadratic equation. Find the integral of x log x by 1 plus x 2 2 under the limits 0 to infinity.
A unique platform where students can interact with teachers/experts/students to get solutions to their queries.
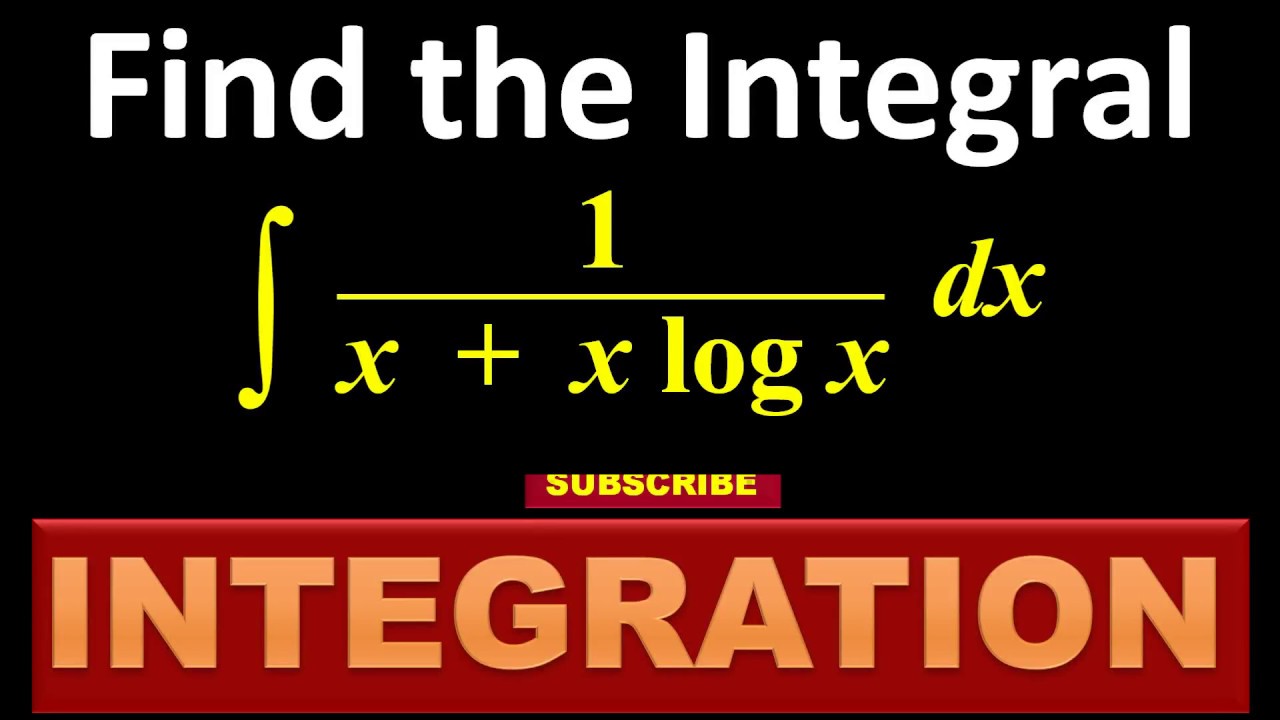
Here are some examples illustrating how to ask for an integral. The integral of 1 1 + x 2 is of the form. Integration of log x ka whole square upon x | integration of (log x) square upon xmain gulshan sharma aapke apne hi bihar munger bhagalpur jila seabout this. Certain other types of integrals.
∫ cos x dx = sin x + c.
Click here👆to get an answer to your question ️ evaluate intsec^2√(x)/√(x) dx Completing the square helps when quadratic functions are involved in the integrand. I=e t( t 2+11 )+c=e logx( (logx) 2+11 )+c. If2x+4=256,find the value of x.
He paid 7% pel annum and returned the amount after 5 years.
Let log 𝑥= 𝑡 differentiate 𝑤.𝑟.𝑡.𝑥 𝑑𝑡/𝑑𝑥= 1/𝑥 𝑑𝑡.𝑥=𝑑𝑥 𝑑𝑥=𝑑𝑡.𝑥 step 2: I = int u^2 cosx * (du)/cosx i = int u^2 du i = 1/3u^3 + c. We get, now back substituting the value of. Ex 7.2, 2 integrate the function:
Let u(x) = 1 + x2 then du(x) = 2xdx.
Integrating function ∫1 (log𝑥 )^2/𝑥 𝑑𝑥 putting (𝑙𝑜𝑔𝑥 )^2=𝑡=𝑥 𝑑𝑡 = ∫1 𝑡^2/𝑥 𝑑𝑡.𝑥 = ∫1 〖𝑡^2 𝑑𝑡〗 = 𝑡^(2+1)/(2+1)+𝐶 = 𝑡. U can use it with the formula of integration by parts😜😜😜😉😀😀easy peasy. Maharashtra state board hsc science (computer science) 12th board exam. As is positive we can write.
1) ankit borrowed $25,000 from a bank.
Because x2 +1 > 0 then ∣∣x2 + 1∣∣ = x2 + 1. Integrate 1/(cos(x)+2) from 0 to 2pi; By changing the square, we may rewrite any quadratic polynomial ax² + bx + c in the form.