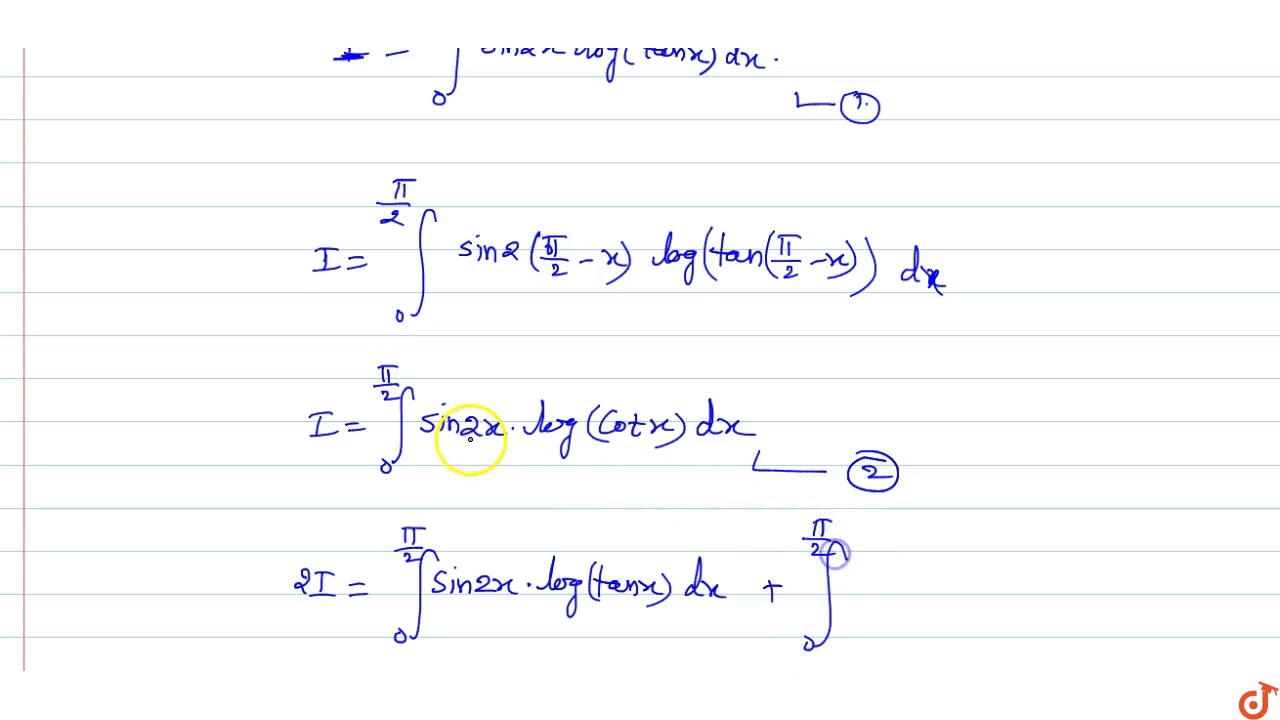Since, this is a definite integral, to integrate it we have to use the following property of definite integrals. ∫ 1 1 + tanx dx = ∫ 1 (1 + u)(1 +u2) du. Now we perform a partial fraction decomposition on the integrand.
What is the integral of under root tanx? Quora
From (i) we can substitute the value, we get.
Kindly cross check your question.
We will be using a definite integral property for solving this question. Int e^x (tan x + log (sec x)) dx. Let i = ∫ 0 π 2 log ( tan x) ⋅ d x. We want to find ∫ 1 1 +tanx dx.
Pioneer, 1 decade ago like.
Ex 7.11, 8 by using the properties of definite integrals, evaluate the integrals : If that is the question which you wanted to ask, then the solution is as follows: I = (u2/2) + c. The value of the integral ∫ log tan x dx x ∈ [0,π ⁄ 2] is equal to :
And found this value using the known sum π 2 6.
Tan𝑥 )] 𝑑𝑥 i=∫_0^ (𝜋/4) log [1+ (1 − tan𝑥)/ (1 + 1. Asked dec 4, 2019 in integrals calculus by jay01 (39.5k points) evaluate: If the answer is not available please wait for a while and a community member will probably answer this soon. ( x) d x = − π 2 24.
If ∫ cos x log(tan (x/2))dx = sin x log(tan (x/2))+ f(x) then f(x) is equal to, (assuming c is a arbitrary real constant) q.
Lata in calculus 1 decade ago, total answer(s): ∫sin 2x log(tan x) dx for x ∈ [0,π/2]. Integration of log(1 + tan x) from 0 to π/4 is equal to π/8 log 2. On combining the like terms we get.
Post your answer (best answer will be rewarded with handsome gifts) please login or register for upload image.
Let u=log (secx+tanx) differentiate wrt x. Answered dec 4, 2019 by abhilasha01 (37.6k points) selected dec 5. Editor toolbars basic styles bold paragraph insert/remove numbered list insert/remove bulleted list insert image insert horizontal. I arrived at this integral while trying different ways to evaluate 1 1 2 + 1 2 2 + 1 3 2 +.
Du/dx = {1/ (secx+tanx)} * { d (secx)/dx + d (tanx)/dx} du/dx = {1/ (secx+tanx)} * { (secx*tanx)+ (sec2x)} du/dx = {1/ (secx+tanx)} * { (tanx)+ (secx)} * secx.
(a) π/4 (b) π/2 (c) 0 (d) π I = ( [log (secx+tanx)] 2/2) + c. We start by substituting u = tanx and du = sec2xdx = (1 + tan2x)dx = (1 +u2)dx. I would like to see a direct proof of the integral.
1 = a(1 + u2) + (bu +c)(1 + u)
∫_0^ (𝜋/4) log (1+tan𝑥 ) 𝑑𝑥 let i=∫_0^ (𝜋/4) log〖 (1+tan𝑥 )〗 𝑑𝑥 ∴ i=∫_0^ (𝜋/4) log [1+tan (𝜋/4−𝑥) ] 𝑑𝑥 i=∫_0^ (𝜋/4) log [1+ (tan 𝜋/4 −tan𝑥)/ (1 +〖 tan〗 𝜋/4. Limit is 0 to π/4.? = ∫ 0 π 2 log ( 1 tan x) ⋅ d x. Integration of tanx or tan x.
Tan𝑥 )] 𝑑𝑥 ex 7.11, 8 by using the properties of definite.
The questions and answers of what is the integration of log (tanx)? Solve the problem in photo showing each step clearly. 1 (1 + u)(1 +u2) = a 1 + u + bu + c 1 + u2. ∫ 0 π / 2 log.
Here, a = π 2.
Are solved by group of students and teacher of class 12, which is also the largest student community of class 12. 1) let x + log(secx) = t 2) on differentiating it with respect to x you get 1+(1/secx*secx.tanx)=dt/dx this has become so because derivative of x w.r.t to x is 1 , derivative of log x is 1/x and chain rule is also used. Click here👆to get an answer to your question ️ evaluate: I = ∫ 0 π / 4 l o g ( 2 1 + t a n x) d x.
Integral 0 to pi/2 of sin 2x log tanx dx.
= ∫ 0 π 2 log ( cot x) ⋅ d x. If ∫ cos x l o g ( t an 2 x ) d x = s in x l o g ( t an 2 x ) + f(x) then f(x) is equal to, (assuming c is a arbitrary real constant) Dx let us consider log(sinx) = z cosx/sinx = z → ∫zdz = z²/2 + c hope it helps you ! This is easy but very important definite integration 0 to pi/2 log(tanx) || cbse ncert xii state broads ||international students ||#definiteintegration #avte.
The answer is log|x+log(secx)| we perform this integration like this int (1+tanx)/(x+log(secx))dx using substitution method :