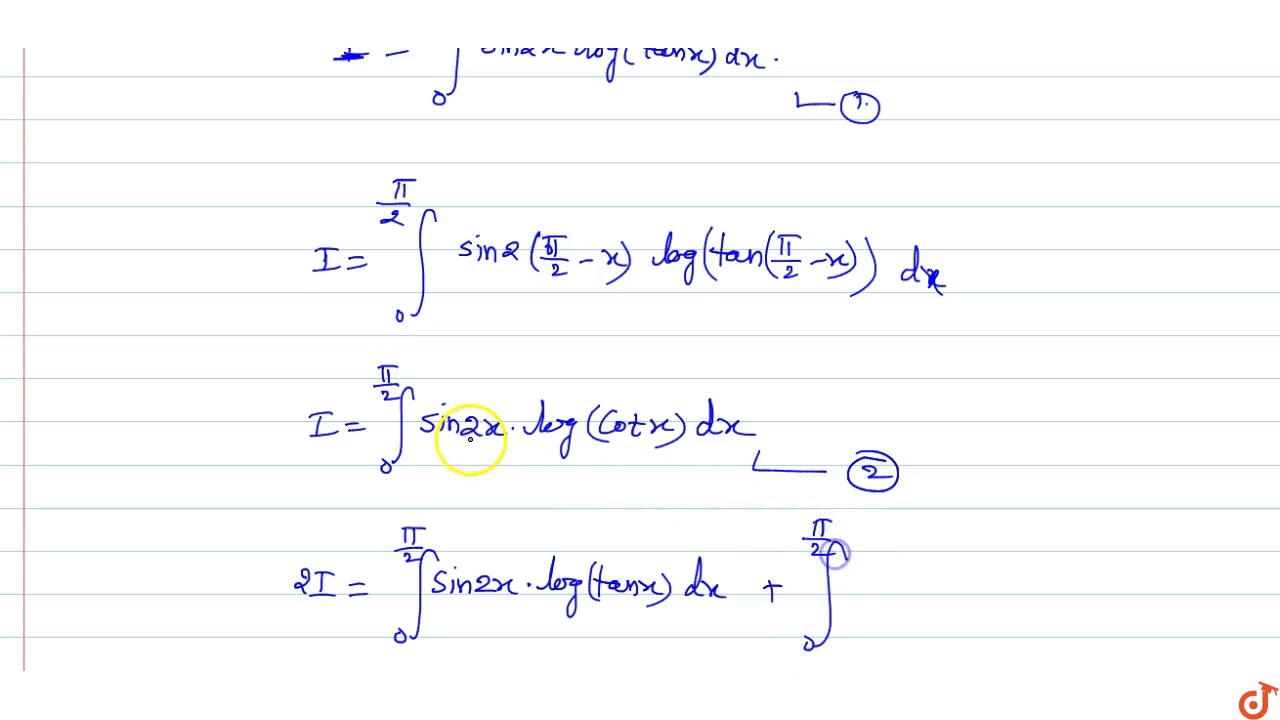I = ∫ 0 π 4 ln. Share it on facebook twitter email. Davneet singh is a graduate from indian institute of technology, kanpur.
Evaluate∫log(1+tanx)dx for x→0,π/4. Sarthaks eConnect
Derivatives derivative applications limits integrals integral applications integral approximation series ode multivariable calculus laplace transform taylor/maclaurin series fourier series functions line equations functions arithmetic & comp.
And found this value using the known sum π 2 6.
Here you will learn proof of integration of tanx or tan x and examples based on it. Integration of log(1 + tan x) from 0 to π/4 is equal to π/8 log 2. Let [math]let t = u^2 => 2u.du = dt => u.du = dt /2 \\[/math] use integration by parts : I = ∫ 0 π / 4 l o g ( 2 1 + t a n x) d x.
(1) (π / 8) loge 2.
∫ 0 π x f ( sin x) d x. Evaluate the integral∫ 0π/4 log(1+tanx)dx. ∫ 0 π / 2 log. He has been teaching from the past 12 years.
Log (1 + tan x) dx =.
Check answer and solution for above mathema tardigrade Asked dec 4, 2019 in integrals calculus by jay01 (39.5k points) evaluate: Let i=int1/(1+tanx)dx apply the substitution tanx=u: To calculate integral of log tan(x), let us put it in the math editor format :
4) (π / 8) loge (1 / 2) solution:
1) (π / 8) loge 2. 3) (π / 4) loge 2. The value of the integral ∫ log tan x dx x ∈ [0,π ⁄ 2] is equal to : I would like to see a direct proof of the integral.
Lata in calculus 1 decade ago, total answer(s):
This is easy but very important definite integration 0 to pi/2 log(tanx) || cbse ncert xii state broads ||international students ||#definiteintegration #avte. Let i = \(\int_{0}^{\pi/4}\) log(1 + tan x) dx. Post your answer (best answer will be rewarded with handsome gifts) please login or register for upload image. By using the properties of definite integrals, evaluate the integral ∫ 0 4 π lo g ( 1 + tan x) d x.
Since, this is a definite integral, to integrate it we have to use the following property of definite integrals.
Here, a = π 2. Integral 0 to pi/2 of sin 2x log tanx dx. Pioneer, 1 decade ago like. ( x) d x = − π 2 24.
Let i = ∫ 0 π 2 log ( tan x) ⋅ d x.
2) (π / 4) log2 e. I=int1/((1+u^2)(1+u))du apply partial fraction decomposition: Dx let us consider log(sinx) = z cosx/sinx = z → ∫zdz = z²/2 + c hope it helps you ! I = ∫ 0 π / 4 l o g ( 1 + 1 − t a n x 1 + t a n x) d x.
The value of the integral ∫ limits0(π/2) log (tan x)dx= (a) 0 (b) 1 (c) (π/2) (d) (π/4).
(a) π/4 (b) π/2 (c) 0 (d) π. Answered dec 4, 2019 by abhilasha01 (37.6k points) selected dec 5. I arrived at this integral while trying different ways to evaluate 1 1 2 + 1 2 2 + 1 3 2 +. ∫sin 2x log(tan x) dx for x ∈ [0,π/2].
Solve the problem in photo showing each step clearly.