Find the formula of integral of ln x whole square using the formula of integral of ln x. Or i = − ( π 2)log2. There is no integral rule for the natural logarithmic function in the integral calculus.
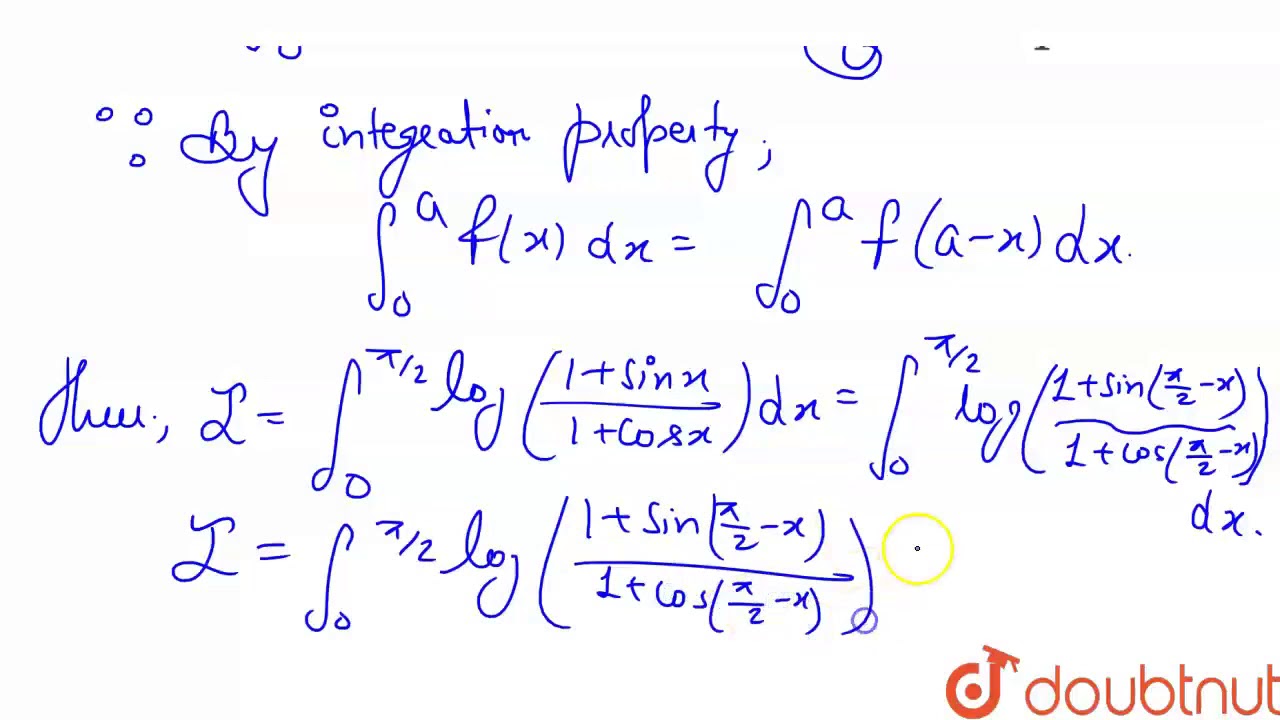
`int_(0)^(pi//2)log ((1+ sinx)/ (1+ cos x )) dx=` YouTube
Where c is the integration constant.
Hence, it is not possible to find the.
Buy now knockout jee main 2022 (one month) personalized ai tutor and adaptive time table, self study material, live classes, unlimited mock tests and personalized analysis reports, 24x7 doubt chat support,. I=∫sin(logx)×1dx =sin(logx)×x−∫cos(logx)×( x1 )×xdx =xsin(logx)−∫cos(logx)×1dx =xsin(logx)−[cos(logx)×x−∫sin(logx)×( x1 )×xdx] ∴i=xsin(logx)−cos(logx)×x−∫sin(logx)dx or2i=x[sin(logx)−cos(logx)] ∴i=( 2x )[sin(logx)−cos(logx)] I 1 = 1 2∫ π 0 logsintdt = ∫ π 2 0 logsintdt = i. Your first 5 questions are on us!
So the final answer is.
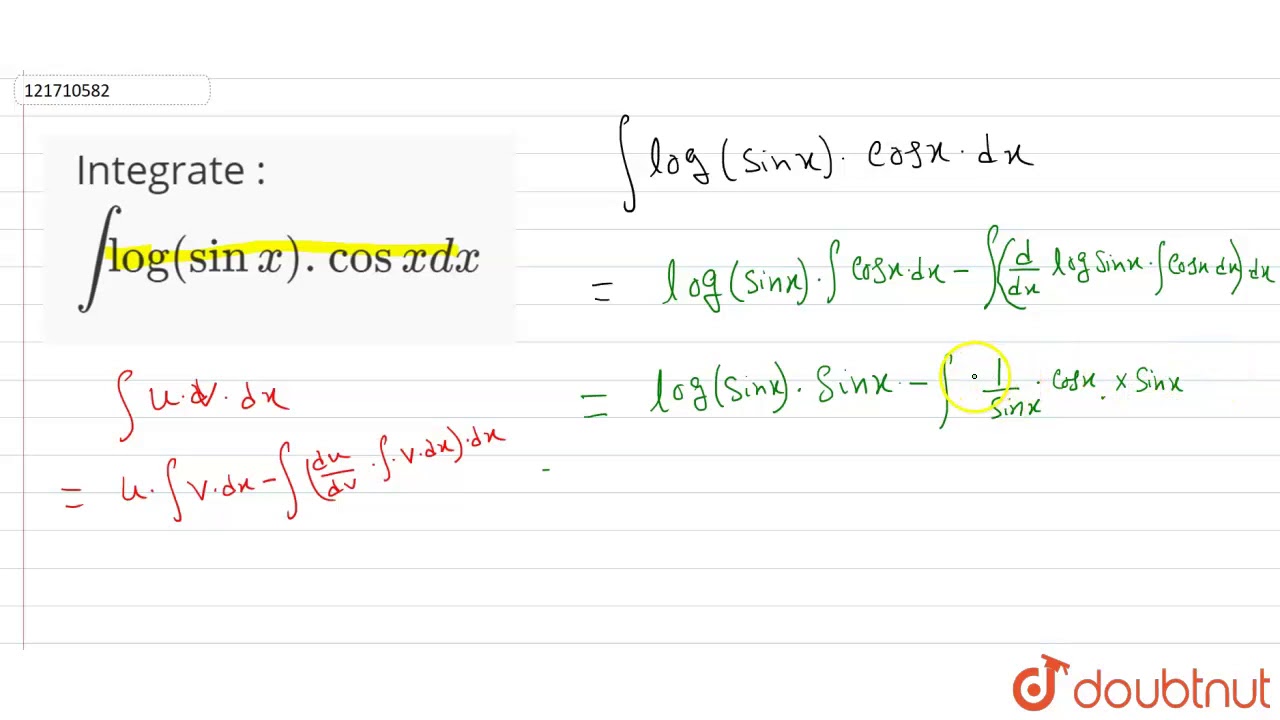
Consider first function as log t and second function as 1. ∫ (1 / x) x cos (log x) + sin (log x)] dx. Where log x is the first function and 1 is the second function according to ilate rule. Integral of log (x) \square!
Note that integrating log(sinx) from 0 to π / 2 is the same as integrating log(cosx) so that ∫π / 2 0 log(sinx)dx = 1 2∫π / 2 0 log(sinxcosx)dx = 1 2∫π / 2 0 log(sin2x)dx − π 4log2.
Putting = & = cot = cot. Starting early can help you score better! ∫cos(log(x))dx = xsin(log(x)) +xcos(log(x)) −∫cos(log(x))dx. = x sin (log x) + c.
Finally, as with all integration without limits, there must be a constant added, which i'll call c.
A definite integral is given: Do the same trick again to get. (3) x sin (log x) + c. Hence, the indefinite integration of the natural logarithmic function with respect to x can be written in one of the following forms in the integral calculus.
The integral of cos (x) is equal to sin (x).
Hence (a) becomes 2i = i −( π 2)log2. Integrating the function cot log sin. Let log sin = differentiating both sides. Integrate 1/(cos(x)+2) from 0 to 2pi;
= 1 + 1 1 + 1 + = 2 2 + = +.
Integral from 0 to pi of sin x dx. ∫ [sin (log x) + cos (log x)] dx. Co = cot = = cot step 2: We can check this by differentiating sin (x), which does indeed give cos (x).
To find the integral of (ln x) 2, we will use the integration by.
⇒ 2 ∫ sin (logx) dx. Now we can integrate v = ∫cos(log(x)) ⋅ 1 x dx = sin(log(x)) (use substitution with w = log(x)) parts gives us: Avail 25% off on study pack. It can be written as.
We will use integration by parts formula to prove this, let i = \(\int\) log x.1 dx.
To avoid ambiguous queries, make sure to use parentheses where necessary. A) approximate the definite integral with the trapezoidal rule and n =. Here are some examples illustrating how to ask for an integral. After a change of variables, this can be rearranged to get the result.
Here you will learn proof of integration of sin inverse x or arcsin x and examples based on it.
Now replace t as cos x. Again by integrating the second term. Ex 7.2, 29 cot log sin step 1: Where c is the integration constant.