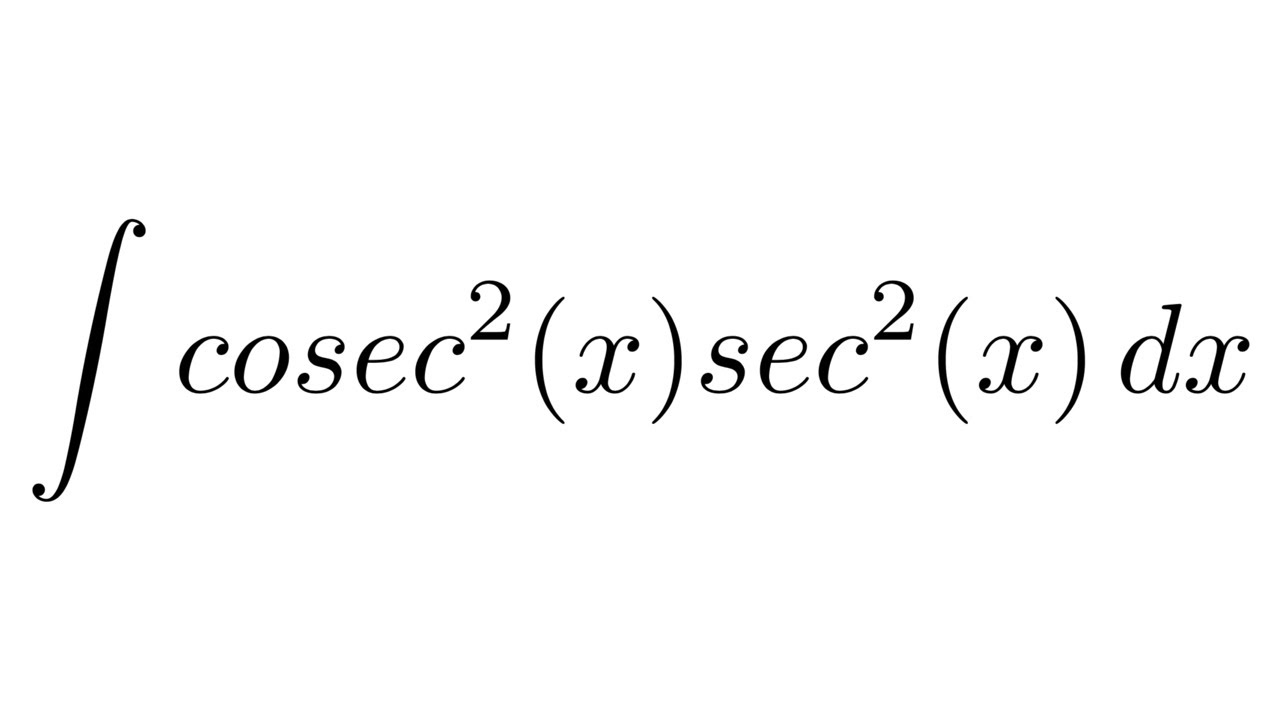Or, \(\int\) cosec x = \(log |tan {x\over 2}|\) + c. Sec² (x/2)/ 2*tan (x/2) dx = dt. Lets assume u = tanx then du/dx = sec 2 x.
cosecx=1+cotx YouTube
Dx/ 2 cos² (x/2) tan (x/2) = dt.
Please log in or register to add a.
X 2 × 1 cos 2. Editor toolbars basic styles bold paragraph insert/remove numbered list insert/remove bulleted list insert image insert horizontal. This is the calculus part of the question complete, it now remains to show that this solution is equivalent to the given solution; Click here👆to get an answer to your question ️ integrate cosecx dx
Log tan (x/2) = t.
There are no standard forms in the edexcel book and i can't work it out. = 1/ 2tan (x/2) cos² (x/2) * log tan (x/2) now assume. We recall the standard trig identity for cosecx, and square both sides. Misc 33 important → chapter 7 class 12 integrals (term 2) serial order wise;
To integrate cosec^2x, also written as ∫cosec 2 x dx, cosec squared x, cosec^2 (x), and (cosec x)^2, we start by using standard trig identities to simplify the integral.
Let i = \(\int\) cosec x dx. And so our integral becomes: Commented feb 9, 2019 by ayush bhatt (19 points) +1. = ln|cscx − cotx| + c.
Let i = ∫ cos2(1+logtan 2x )cosec x dxput 1+logtan 2x = t⇒ tan2x 1.sec2 2x.21 dx = dt⇒ 2sin 2x cos 2x 1 dx = dt⇒ cosec xdx = dt∴ i = ∫ cos2tdt = ∫ sec2tdt= tant +c= tan(1+logtan 2x )+ c.
For cot x you follow a similar principle, cot x= cos x/sin x so integral = ln (sin x) Integration of cosec x dx in terms of tan. We divide the numerator and denominator by cos squared x. Integral of cosec x by trigonometric formulas.
Hope now you can do it further.
Secx*log(secx+tanx) dx let u=log(secx+tanx) differentiate wrt x du/dx = {1/(secx+tanx)} * { d(secx)/dx + d(tanx)/dx} du/dx = {1/(secx+tanx)} * { (secx*tanx)+(sec2x)}. We can write cosec x as 1/(sin x). Answered apr 21, 2018 by rubby (52.4k points) selected apr 22, 2018 by vikash kumar. Cosec 2 x = (1 / cos 2 x) / (sin 2 x / cos 2 x) put 1 / cos 2 x = sec 2 x and sin 2 x / cos 2 x = tan 2 x.
Multiply by cos (x/2) in both numerator or denominator.
Solve the problem in photo showing each step clearly. Use the identity that integral of f' (x)/f (x) = ln f (x) + c. So final expression for integration is ∫ (cosec 2 x) dx = ∫ (sec 2 x / tan 2 x) dx. To find the integral of tan square x, we can use the trigonometric identities such as tan x = sin x/cos x and 1 + tan 2 x = sec 2 x.
X 2 × d x = d t.
\int cosec x\ dx =\int \frac{1}{\sin x}\ dx =\int \frac{1}{\frac{2\tan\frac x2}{1+\tan^2\frac x2}}\ dx =\int \frac{1+\tan^2\frac x2}{2\tan\frac x2}\ dx =\int \frac{\sec^2\frac x2}{2\tan\frac x2}\ dx =\int \frac{1}{\tan\frac x2}\cdot \frac12\sec^2\frac x2\ dx =\in. Where c is the integration constant. We will get cosec 2 x = sec 2 x / tan 2 x. Click here👆to get an answer to your question ️ integration sec^2x.cosec^4x
∫ cscx dx = ∫ 1 u du.
Lets start the integration of cosec 2x. Integration of cosec x formula. Integration of cosec x dx. Cscx − cotx = 1 sinx − cosx sinx.
We can prove that the integral of cosec x to be ln | tan (x/2) | + c by using trigonometric formulas.
Integration of cosecx or cosec x.