To integrate cosec^2x, also written as ∫cosec 2 x dx, cosec squared x, cosec^2 (x), and (cosec x)^2, we start by using standard trig identities to simplify the integral. Here you will learn proof of integration of sec inverse x and cosec inverse x. Integral of square cosecant $$\int \csc^{2}x \ dx$$ maybe some of them cause a bit of conflict the resolution of this integral, but we know very well that the integrals and the derivatives are linked by a very strong friendship bond, which means that if someone derives something and the result of that something is integrated, the result will be that something with.
`int("cosec x")/(cos^(2)(1+log tan.(x)/(2)))dx` is equal
∫ cscx dx = ∫ 1 u du.
X d x = ln.
How to integrate tan x. Where c is the integration constant. Lets assume u = tanx then du/dx = sec 2 x. Lets start the integration of cosec 2x.
Log a ^b = b log a log a b = b log a.therefore, − ln | cosec x + cot x | = ln 1/ | cosec x + cot x |.
= ln|cscx − cotx| + c. We can prove that the integral of cosec x to be ln | tan (x/2) | + c by using trigonometric formulas. We can write cosec x as 1/(sin x). So final expression for integration is ∫(cosec 2 x) dx = ∫(sec 2 x / tan 2 x) dx.
The indefinite integration of product of cosecant and cot functions with respect to x is equal to the sum of negative cosecant function and an integral constant.
Where c is the integration constant. Let us assume that log. Integration of cosec x formula. Here is the list of some important and most commonly asked formulas on advanced integration functions:
Cscx − cotx = 1 sinx − cosx sinx.
Integration of cosecx or cosec x. In this method of integration by substitution, any given integral is transformed into a simple form of integral by substituting the independent variable by others. Integration of cosec x in terms of log. Cosec 2 x = (1 / cos 2 x) / (sin 2 x / cos 2 x) put 1 / cos 2 x = sec 2 x and sin 2 x / cos 2 x = tan 2 x.
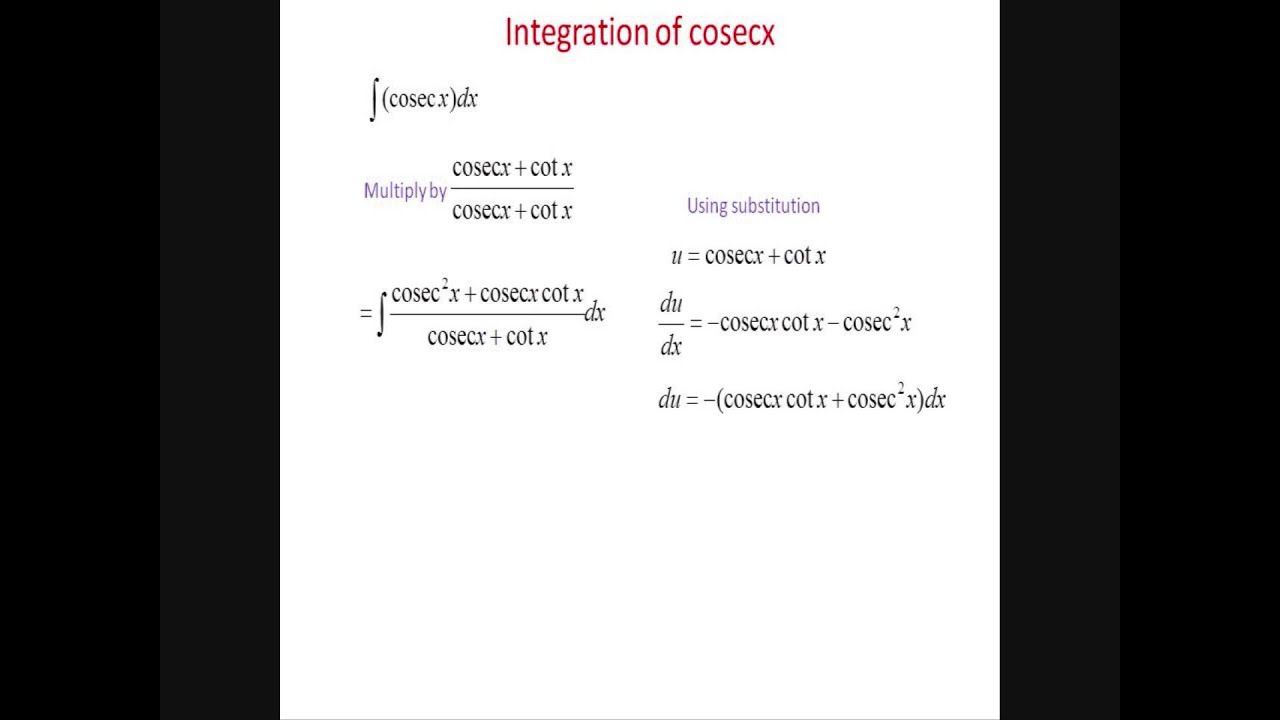
Integration of cosec x dx.
Or, \(\int\) cosec x = \(log |tan {x\over 2}|\) + c. X functions with respect to x is written in the following mathematical form in integral calculus. X 2 × 1 cos 2. ( x 2)) + c.
Use this property of logarithm:
Integration of cosec x dx in terms of tan. Let i = \(\int\) cosec x dx. U = cscx −cotx ⇒ du dx = −cscxcotx + csc2x, and so our integral becomes: Combine this result with the use of integration by parts, specifically, let u = x d u = d x and d v = 1 sin.
Integral of cosec x by trigonometric formulas.
[latex] ∫ cosec(x−a)cosecxdx = ∫ 1 sin(x−a)sinx dx = 1 sina ∫ sin(x−(x−a)) sinxsin(x−a) dx = 1 sina ∫ sinxcos(x−a)−cosxsin(x−a) sinxsin(x−a) dx = 1 sina ∫(cot(x−a)−cotx)dx = 1 sina(lnsin(x−a)|−ln|sinx ∣)+c = 1 sina(ln∣∣sin x−a sinx∣∣) +c ∫ cosec. We will get cosec 2 x = sec 2 x / tan 2 x; Let i = ∫ cos2(1+logtan 2x )cosec x dxput 1+logtan 2x = t⇒ tan2x 1.sec2 2x.21 dx = dt⇒ 2sin 2x cos 2x 1 dx = dt⇒ cosec xdx = dt∴ i = ∫ cos2tdt = ∫ sec2tdt= tant +c= tan(1+logtan 2x )+ c. This is the calculus part of the question complete, it now remains to show that this solution is equivalent to the given solution;
We recall the standard trig identity for cosecx, and square both sides.