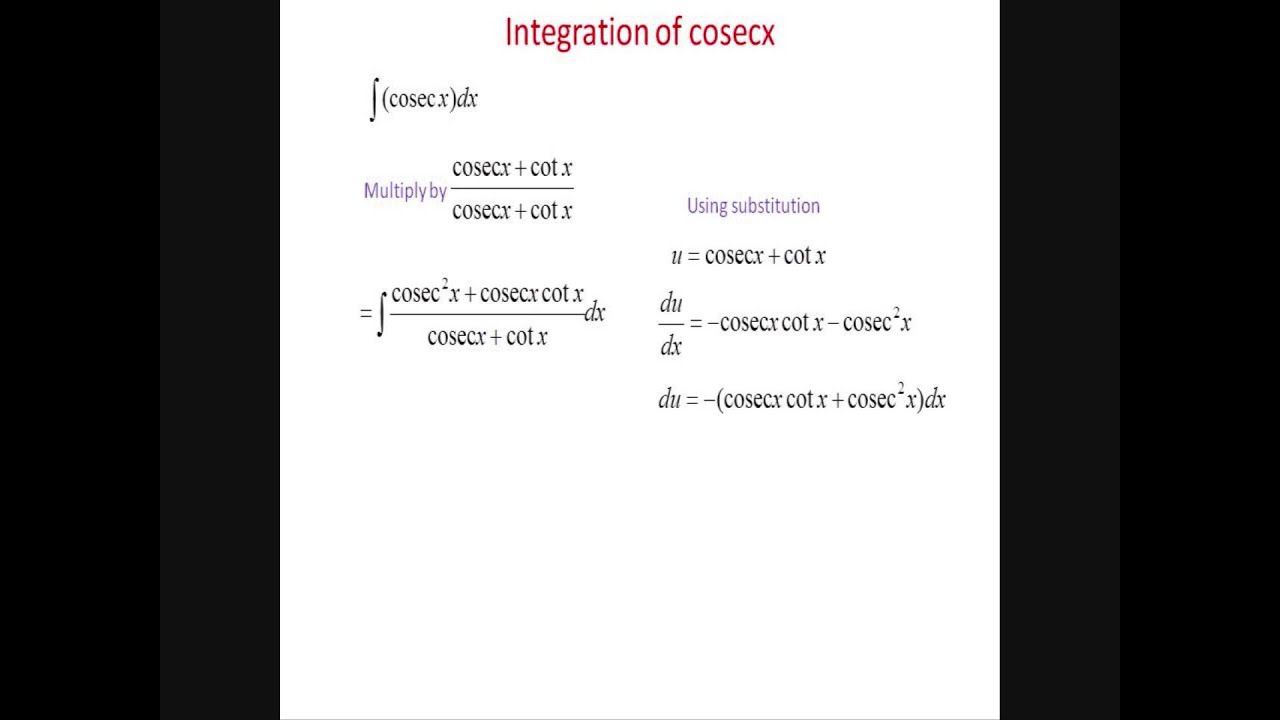
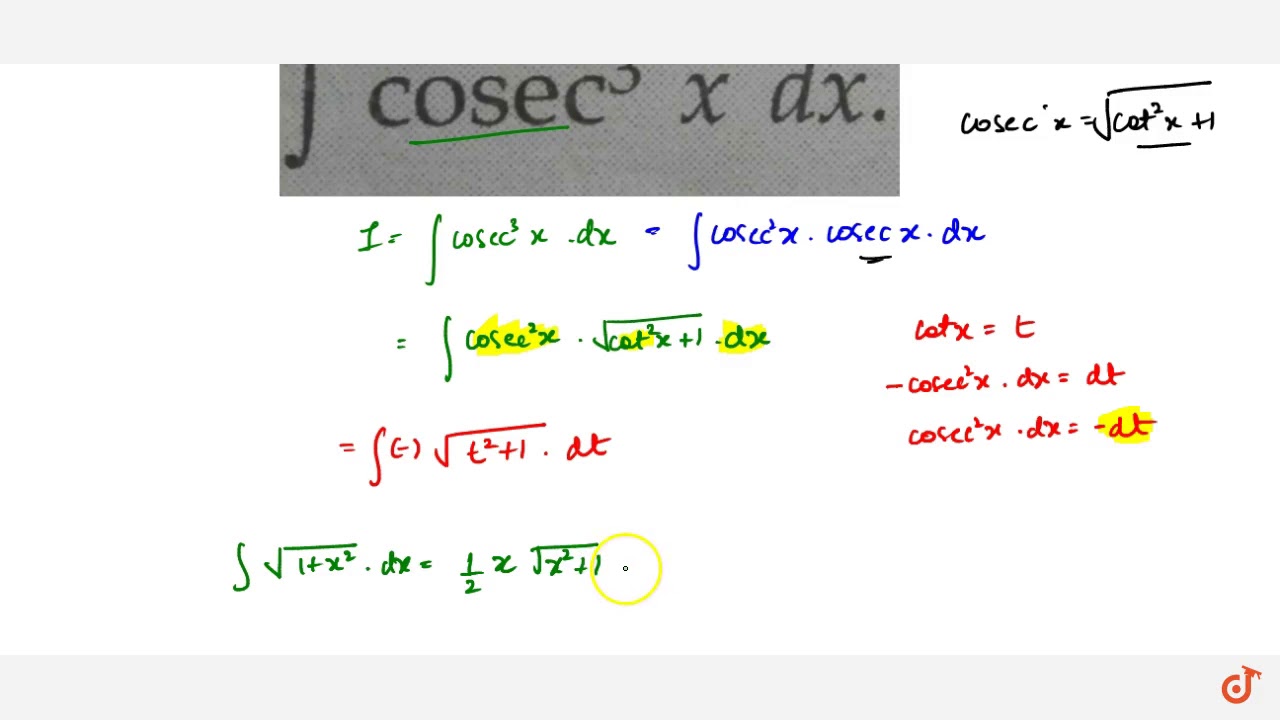
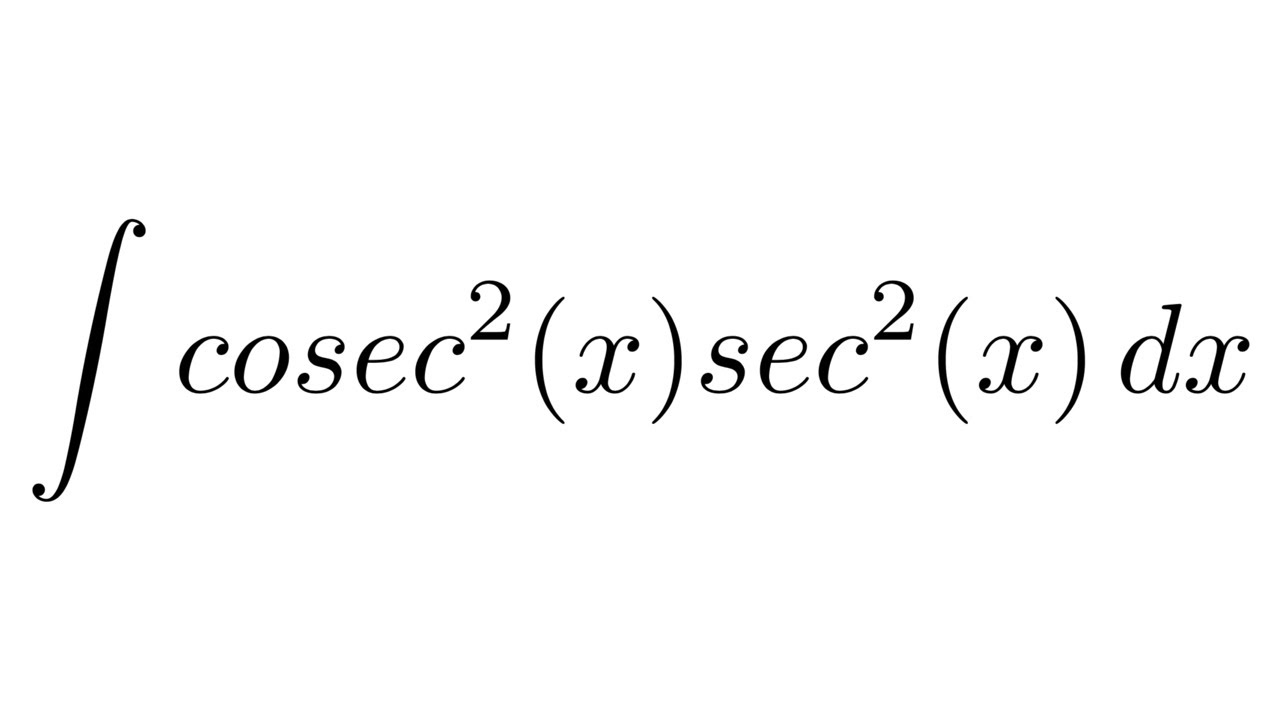Log a ^b = b log a log a b = b log a.therefore, − ln | cosec x + cot x | = ln 1/ | cosec x + cot x |. = ∫ cscx − 1 cot2x dx. Log(secx + tanx) then, (secx + tanx) ⇒ cscx dx = dt.
Integration of cosecx YouTube
Use this property of logarithm:
1 2 ∫ − 1 u d u.
Or, ∫ cosec x = l o g | t a n x 2 | + c. Put log x = t. In this method of integration by substitution, any given integral is transformed into a simple form of integral by substituting the independent variable by others. Here ln represents the natural logarithmand 'c' is the constant of integration.
= ∫ cscx −1 (cscx − 1)(cscx + 1) dx.
Put t and dt in given equation we get. Once we have u and d u, we will substitute them in the integral, as follows: Integration of cosec x dx in terms of tan. By substituting the value of t.
Integration of cosec x in terms of log.
Applying properties of integrals we will extract the negative of the integral: Solve the problem in photo showing each step clearly. ∫ ( textcosecx / cos2(1+ log tan (x)2)) dx = q. Let u=log (secx+tanx) differentiate wrt x.
Multiple formulas for the integral of cosec x are listed below:
Please log in or register to add a comment. Let u = cos x and then proceed by partial fractions after the substitution. Using the subsitution $u = \tan \left(\frac{x}{2}\right)$, we get: Integration of cosec x formula.
According to the logarithms, the natural logarithm is also.
Where c is the integration constant. We have multiple formulas for this. ∫ 1 1 + cscx dx. Click here👆to get an answer to your question ️ integrate cosecx dx
Rep gems come when your posts are rated by other community members.
Take log x = t. Substituting the values oft and dt we get Let us assume that log. Selected jun 27, 2020 by vikram01.
Yes, $x\,\mathrm{cosec}\,x$ is integrable and has a closed form.
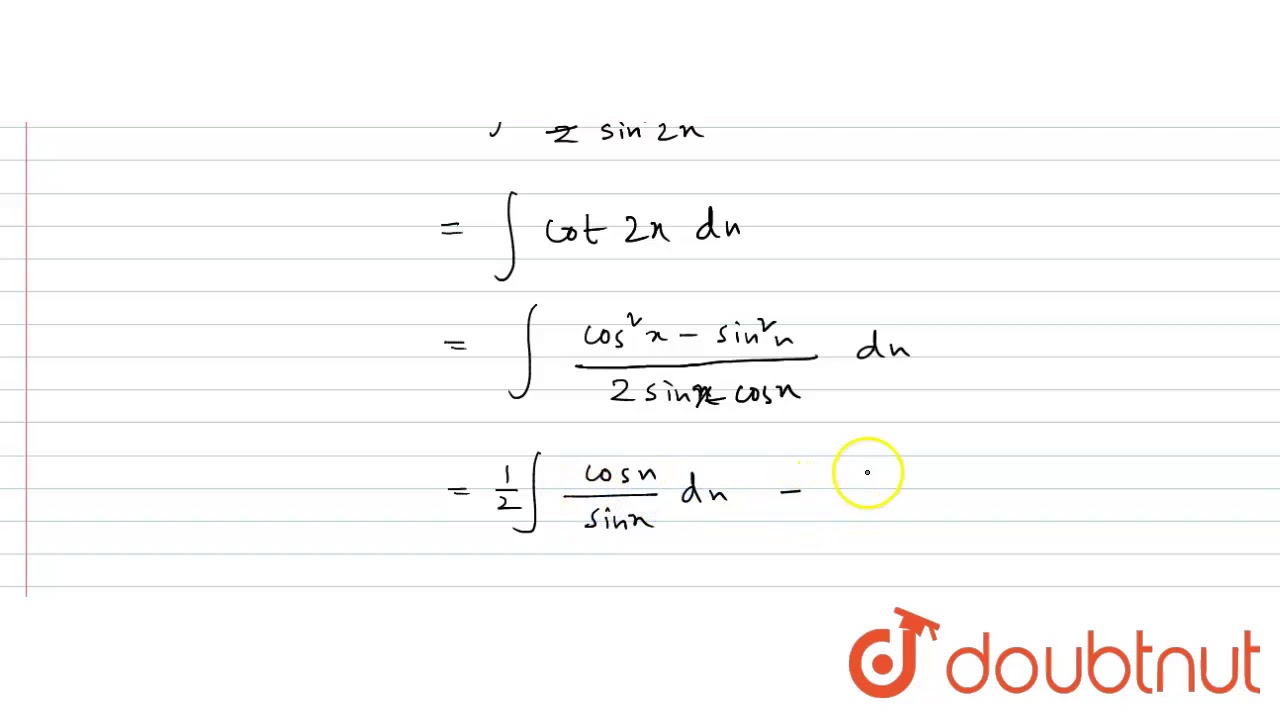
= ∫( sinx cos2x −sec2x + 1)dx. Editor toolbars basic styles bold paragraph insert/remove numbered list insert/remove bulleted list insert image insert horizontal. X 2 × 1 cos 2. Integration of cosec x dx.
∴ dx = e t.dt.
− 1 2 ∫ 1 u d u. $$\int \frac{1}{\sin x} \, \mathrm{d}x = \ln \left(\tan \left(\frac{x}{2}\right)\right) + \mathrm{c}$$ ∫ c o s 2 ( 1 + l o g t an 2 x ) cosecx d x = 1436 21 kcet kcet 2006 integrals report error = ∫ cscx −1 csc2x −1 dx.
Du/dx = {1/ (secx+tanx)} * { d (secx)/dx + d (tanx)/dx} du/dx = {1/ (secx+tanx)} * { (secx*tanx)+ (sec2x)} du/dx = {1/ (secx+tanx)} * {.
= cosec cosec ∫ e t [ cosec t + d d t ( cosec t)]. = ∫( sin2x sinxcos2x −tan2x)dx.