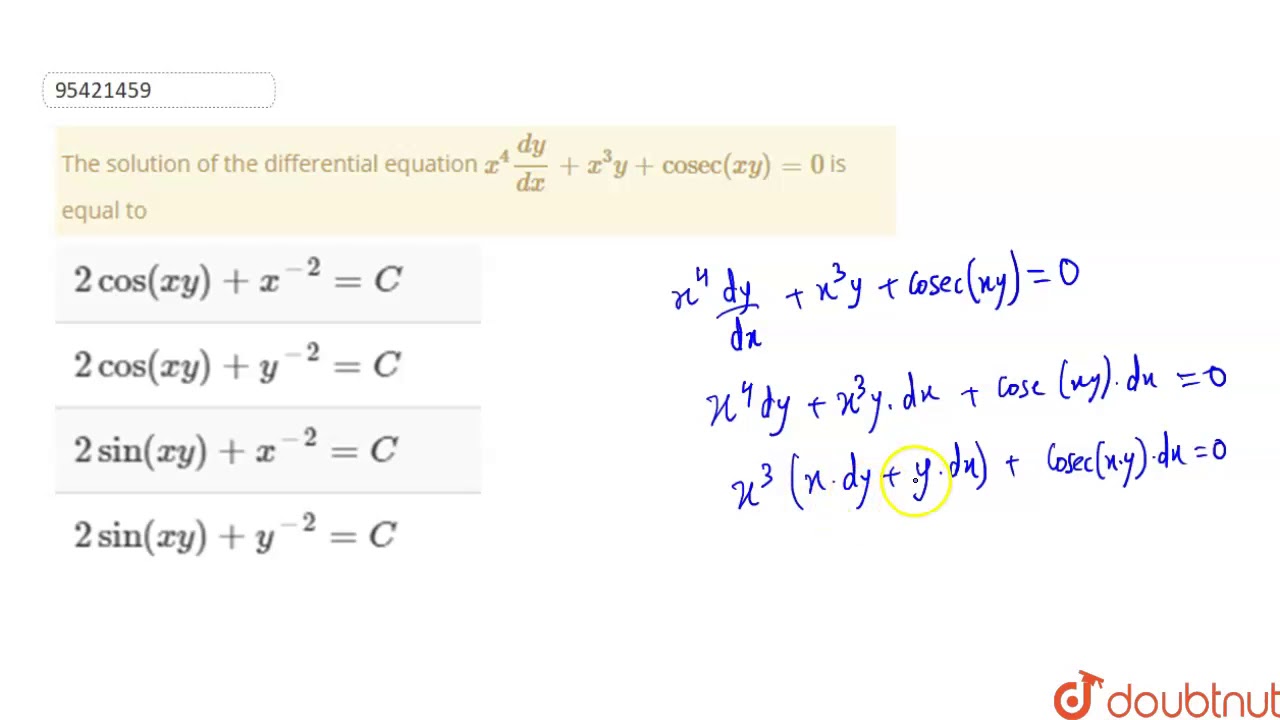
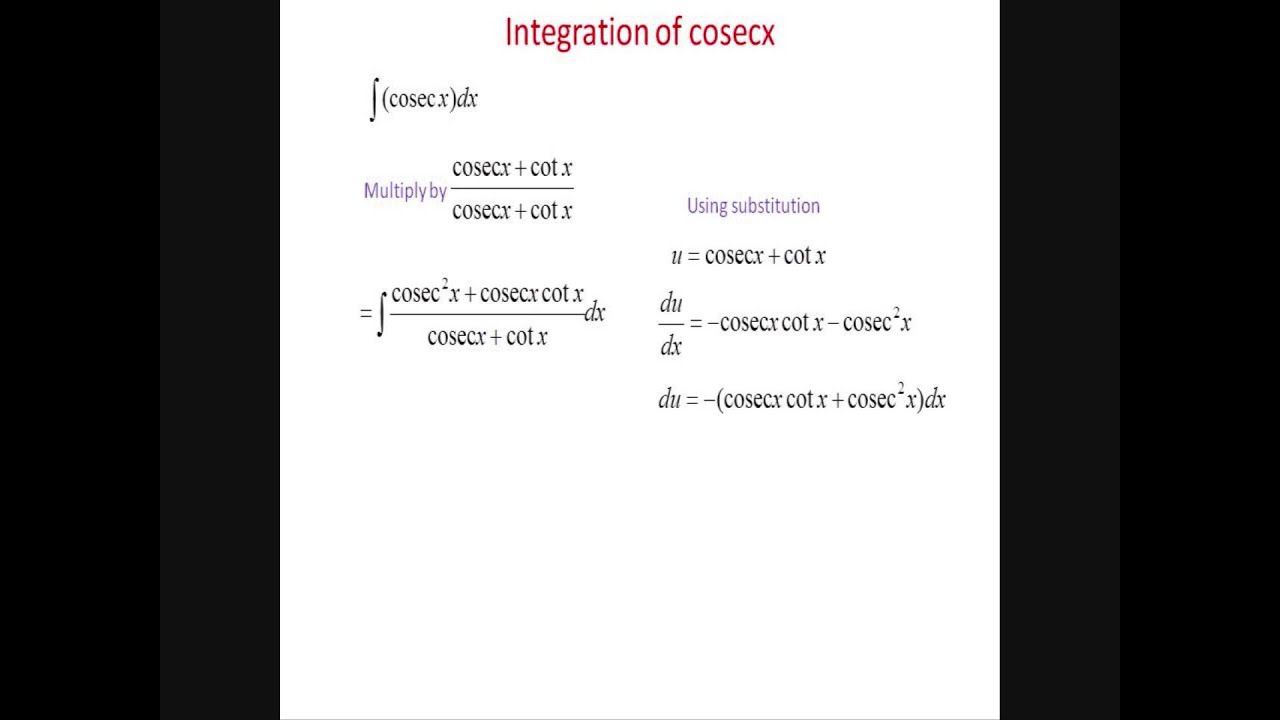
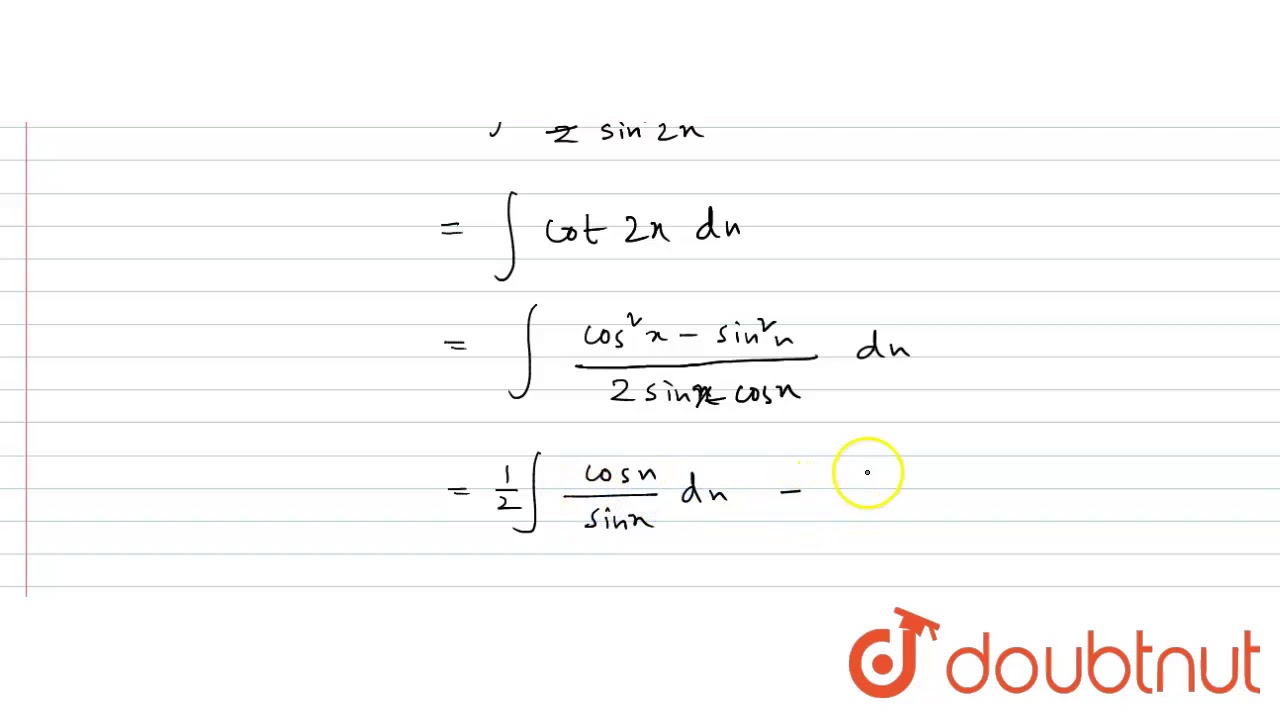
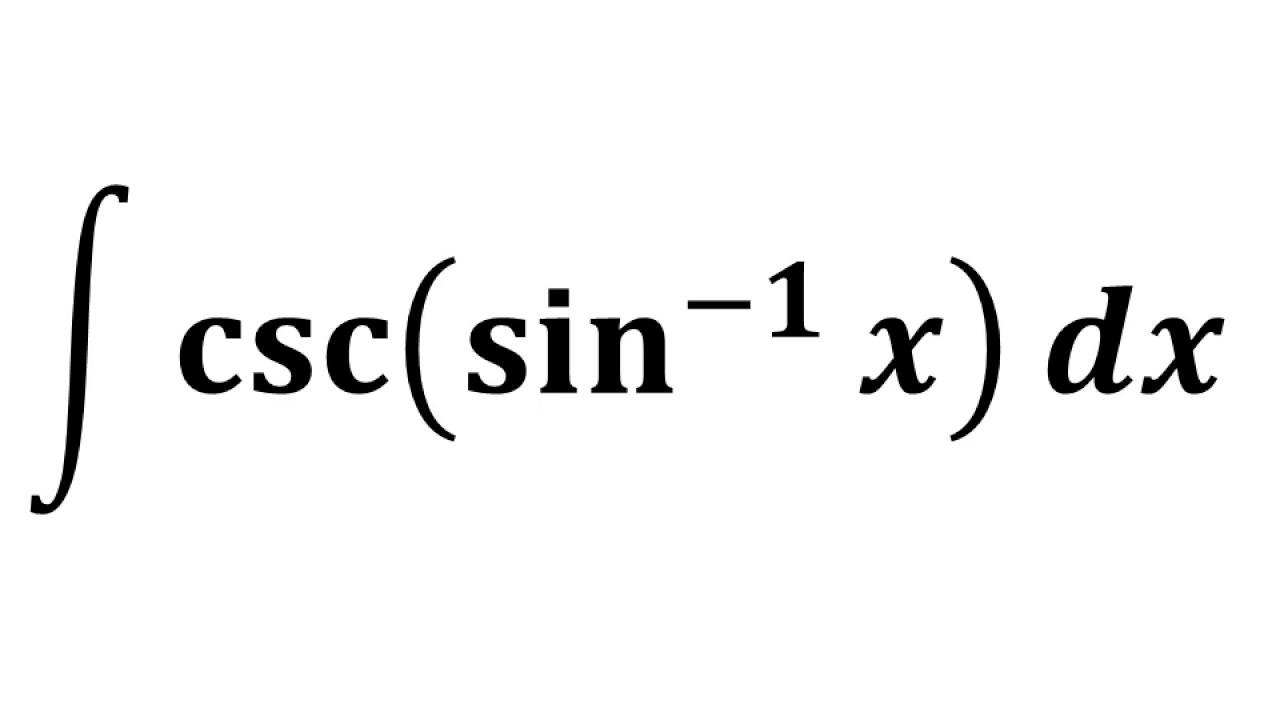And let dv = csc2(x). Integration of type ∫sin mx. ∫ a dx = ax+ c.
[無料ダウンロード! √] — Ž€ŠC•¶ ‘ŠO“T 264726C o t e
This is also known as the antiderivative of cosecx.
We can rewrite integration of cosec 2x as ∫cosec 2 x dx, or integral of cosec square x or cosec^2x or (cosec x) 2.we will use trigonometric identities to find the integral of cosec^2x.
As you know that cosec x = 1/sinx so cosec 2 x = 1/ sin 2 x. Where c is the integration constant. To integrate cosec^2x, also written as ∫cosec 2 x dx, cosec squared x, cosec^2 (x), and (cosec x)^2, we start by using standard trig identities to simplify the integral. Du = (n − 2)cscn−3(x) ⋅ ( − csc(x)cot(x))dx.
∫ sec x dx = log|sec x + tan x| + c.
Integration of cosecx or cosec x. Although i'd like to mention that the identity $sin(2x) = \frac{2tan(x)}{1+tan^2x}$ is worth memorizing. ∫ sec 2 x dx = tan x + c. ∫ x n dx = ( (x n+1 )/ (n+1))+c ;
Some generalised results obtained using the fundamental theorems.
To prove this formula, consider. Or, ∫ cosec x = l o g | t a n x 2 | + c. We recall the standard trig identity for cosecx, and square both sides. ∫ cosec 2 x dx =.
Integration of cosecant squared of x is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae.
Applying this, ∫ sec x dx = ∫ 1 / [ 2 sin [ (x/2) + (π/4) ] cos [ (x/2) + (π/4) ] ] dx. ∫ cot x dx = log|sin x| + c. = ∫ 1/ (sin (x + π/2)) dx. Integral formulas of trigonometric functions:
We can write sec x as 1/ (cos x) where cos x can again be written as sin (x + π/2).
The list of basic integral formulas are. If m is an odd natural number then put cos x = t. ∫ cos x dx = sin x + c. We divide the numerator and denominator by cos squared x.
Multiple formulas for the integral of cosec x are listed below:
$\begingroup$ $cosec(2x) = \frac{1}{sin(2x)} = \frac{1}{2sin(x)cos(x)} = \frac{cosx}{2sin(x)cos^{2}x} = \frac{sec^{2}x}{2\frac{sinx}{cosx}} = \frac{1+tan^2x}{2tanx}$. The integration of cosecant squared of x is of the form. ∫ cos x dx = sin x + c. I = − cot(x)cscn−2(x) − (n − 2)∫cscn−2(x)cot2(x)dx.
∫ 1 dx = x + c.
∫ sec x dx = ∫ 1/ (cos x) dx. Let u = cos x and then proceed by partial fractions after the substitution. ∫ tan x dx = log|sec x| + c. 6 rows basic formulas on integration.
The integral of the cosecant of angle x with respect to x is equal to the natural logarithm of the subtraction of cot of angle x from cosecant of angle x, and.
∫ sec 2 x dx = tan x + c. Integrating this gives v = −cot(x). Now, through the pythagorean identity, let cot2(x) = csc2(x) −1. A ( d r) + b d d x ( d r) + c.
We have multiple formulas for this.
Now we will divide both numerator and denominator by cos 2 x.