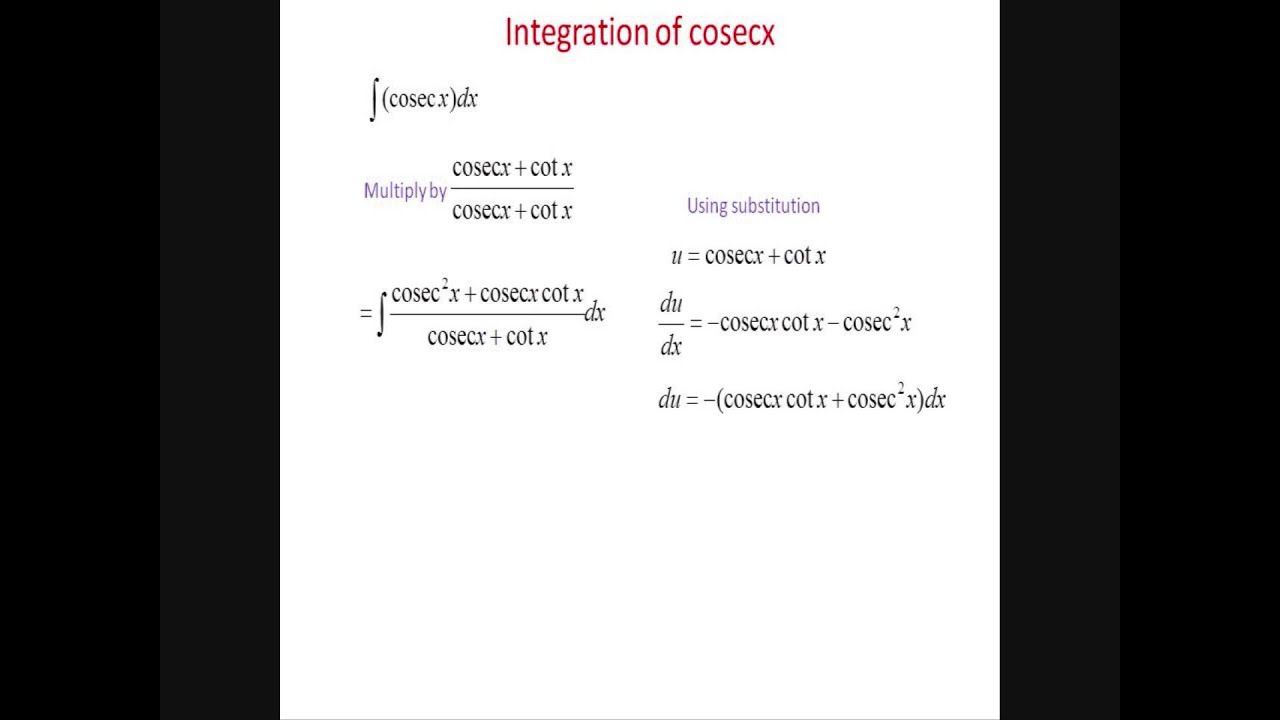
∫(cosec 2 x) dx = ∫(sec 2 x / tan 2 x) dx; The purpose of making this change will become apparent in the next two steps. We have multiple formulas for this.
Misc 13 Find particular solution dy/dx + y cot x = 4x
∫ sinx dx = −cosx+c 2.
Using the substitution method, ∫ sec x dx = ln |sec x + tan x| + c;
∫ a dx = ax+ c. Now, perform integration by parts on this, taking the form ∫udv = uv − ∫vdu. X d x = sin. Lets start the integration of cosec 2x.
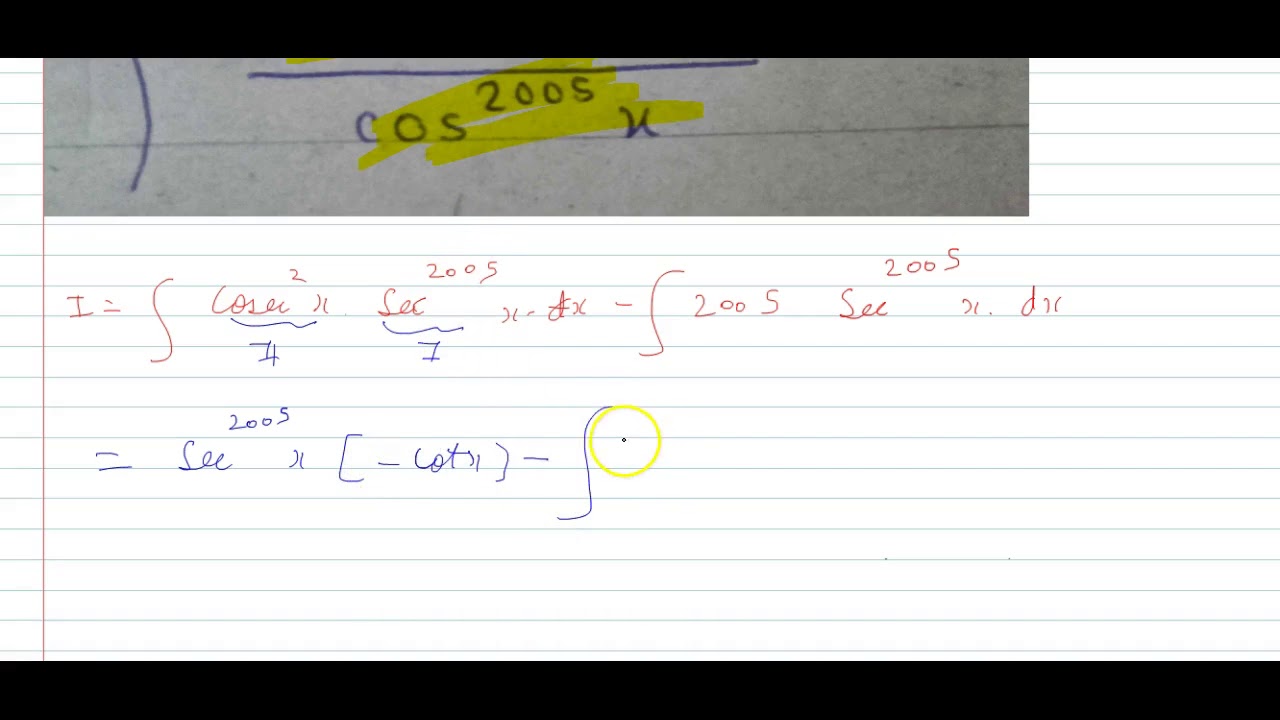
= ∫ cosecn x dx, n being a positive integer, n ≥ 2 and hence deduce the value of ∫ cosec5 x dx.
𝑑𝑥〗multiplying and dividing by 𝑐𝑜𝑠𝑒𝑐 𝑥+𝑐𝑜𝑡 𝑥 f(𝑥)=∫1 (𝑐𝑜𝑠𝑒𝑐 𝑥 (𝑐𝑜𝑠𝑒𝑐 𝑥 + 𝑐𝑜𝑡 𝑥))/(𝑐𝑜𝑠𝑒𝑐 𝑥 + 𝑐𝑜𝑡 𝑥) 𝑑𝑥let c𝑜𝑠𝑒𝑐 𝑥+𝑐𝑜𝑡 𝑥. However, in any specific problem one has to keep it in mind. ∫e dx exx =+ c (xv) 1 log| | d x dx x = ; X d x = − cot.
Here a and b are the constants.
Du = (n − 2)cscn−3(x) ⋅ ( − csc(x)cot(x))dx. 1 dx xlog| | c x ∫ =+ (xvi) x da ax dx log a = ; So final expression for integration is ∫(cosec 2 x) dx = ∫(sec 2 x / tan 2 x) dx. C x a dxx a log a ∫ =+ note in practice, we normally do not mention the interval over which the various functions are defined.
I = ∫cscn(x)dx = ∫cscn−2(x)csc2(x)dx.
And let dv = csc2(x). The indefinite integral of cosecant squared of angle x function with respect to x is equal to sum of the negative cotangent of angle x and a constant of integration. ∫ 1 dx = x + c. ( − c o s e c x c o t x + c o s e c 2 x) dx = dt.
Hence, we get a new expression for cosec squared x.
∫ cos x dx = sin x + c. X d x or ∫ cosec 2. The list of basic integral formulas are. This is also known as the antiderivative of cosecx.
In this video, we will learn to integrate the cosecant of a general linear form ax + b.
We rearrange it for du. ∫ cosx dx = sinx+c 1. New we can rearrange it as du = sec 2 x dx because same term is available in our integration expression. X d x = − cos.
Students, teachers, parents, and everyone can find solutions to their math problems instantly.
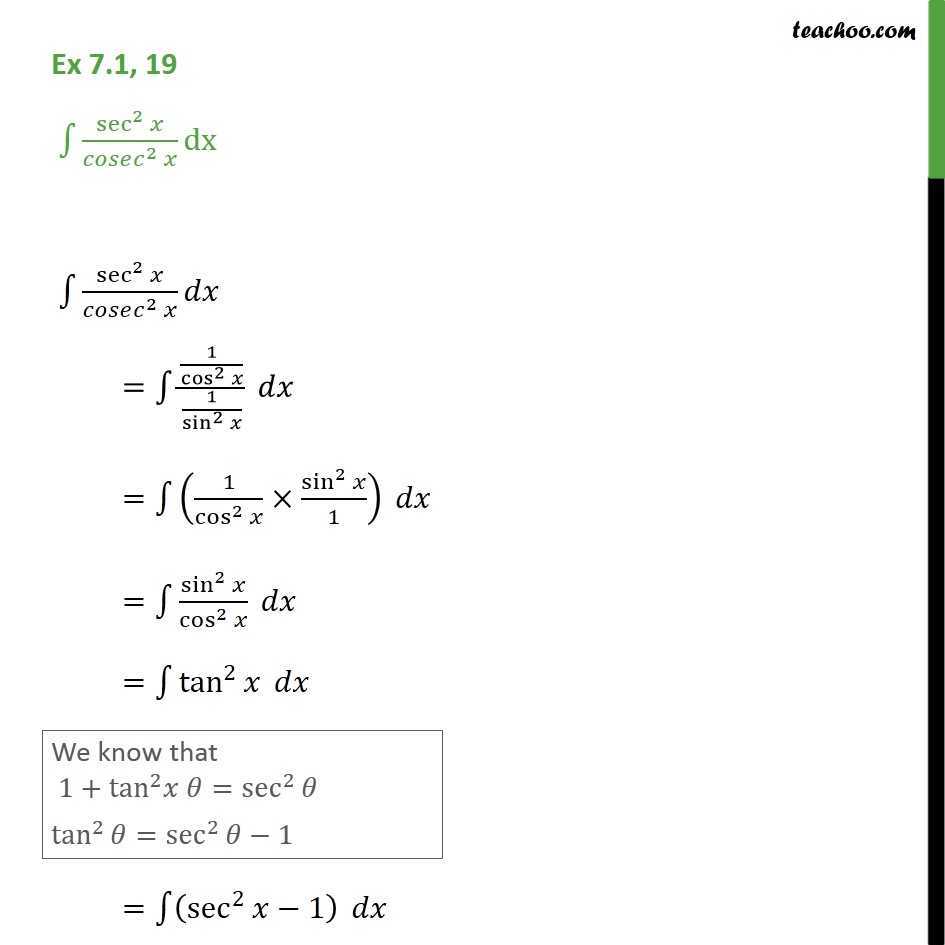
∫ x n dx = ( (x n+1 )/ (n+1))+c ; Lets assume u = tanx ∫ sec 2 x dx = tan x + c. Here are the formulas of integral of secant x with the respective methods of proving them.
We let u = tanx.
Integrating this gives v = −cot(x). Hence, we get a new integration expression on the rhs, that means the same thing as the lhs. Then, du/dx = sec 2 x. Ex 7.9, 8∫_(𝜋/6)^(𝜋/4) 〖𝑐𝑜𝑠𝑒𝑐 𝑥〗𝑑𝑥 let f(𝑥)=∫1 〖𝑐𝑜𝑠𝑒𝑐 𝑥.
Lets assume u = tanx then du/dx = sec 2 x.
Important notes related to integration of sec x: As you can see, sec 2 x dx are the same terms in our integration problem, hence we can. Free math lessons and math homework help from basic math to algebra, geometry and beyond.