Integration Of Cosec X Cot X Dx `int(1)/(tanx+cotx+secx+"cosec "x)dx` Is Equal To YouTube

Asked feb 10, 2020 in indefinite integral by kumkumbharti (53.9k points) evaluate ∫cosecx[cosecx + cotx]dx. We know that ∫ cosec 3 x dx = 1. Hence, we get a new expression for cosec squared x.

Integral cot^4 x dx YouTube

We will use the identity cosec 2 x = 1 + cot 2 x to integrate cot 2 (x)dx.

Then, du/dx = sec 2 x.

`int sqrt (1 + 2 cot x ( cot x + cosecx ) ) dx` =. Each of the integral above being of standard form, we straighta. If i = ∫ sec 2 x c o s e c 4 x d x = a cot 3 x + b tan x + c cot x + d then. ( − c o s e c x c o t x + c o s e c 2 x) dx = dt.

This is the calculus part of the question complete, it now remains to show that this solution is equivalent to the given solution;

Let i = ∫[(1/tanx) + cosec x + cot x + sec x] dx. Integration of the cosecant cotangent function is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae. Now, ∫cot 2 (x)dx = ∫(cosec 2 x−1)dx =∫cosec 2 (x)dx − ∫1.dx =−cotx −x +c. We let u = tanx.

Let's integrate cot 2 (x)dx.

∴ i = ∫(1/tanx) dx + ∫cosec x dx + ∫cot x dx + ∫sec x dx = ∫cot x dx + ∫cosec x dx + ∫cot x dx + ∫sec x dx = 2∫cot x dx + ∫cosec x dx + ∫sec x. ∫₀π/2 cosec x dx is obtained by applying the limits 0 and π/2 for this. $\displaystyle\int \frac{dx}{\tan x + \cot x + \csc x + \sec x}=$ $\displaystyle\int\frac{\sin x\cos x}{1+\sin x+\cos x}\,dx=\int\frac{\sin x\cos x}{1+\sin x+\cos x. We know that, cosec 2 x = 1 + cot 2 x.

Share it on facebook twitter email.

∫ cscx dx = ∫ 1 u du. X d x = ∫ cosec 2. Cscx − cotx = 1 sinx − cosx sinx. The indefinite integration of product of cosecant and cot functions with respect to $x$ is equal to the sum of negative cosecant function and an integral constant.

Int sqrt (1 + 2 cot x ( cot x + cosecx ) ) dx.

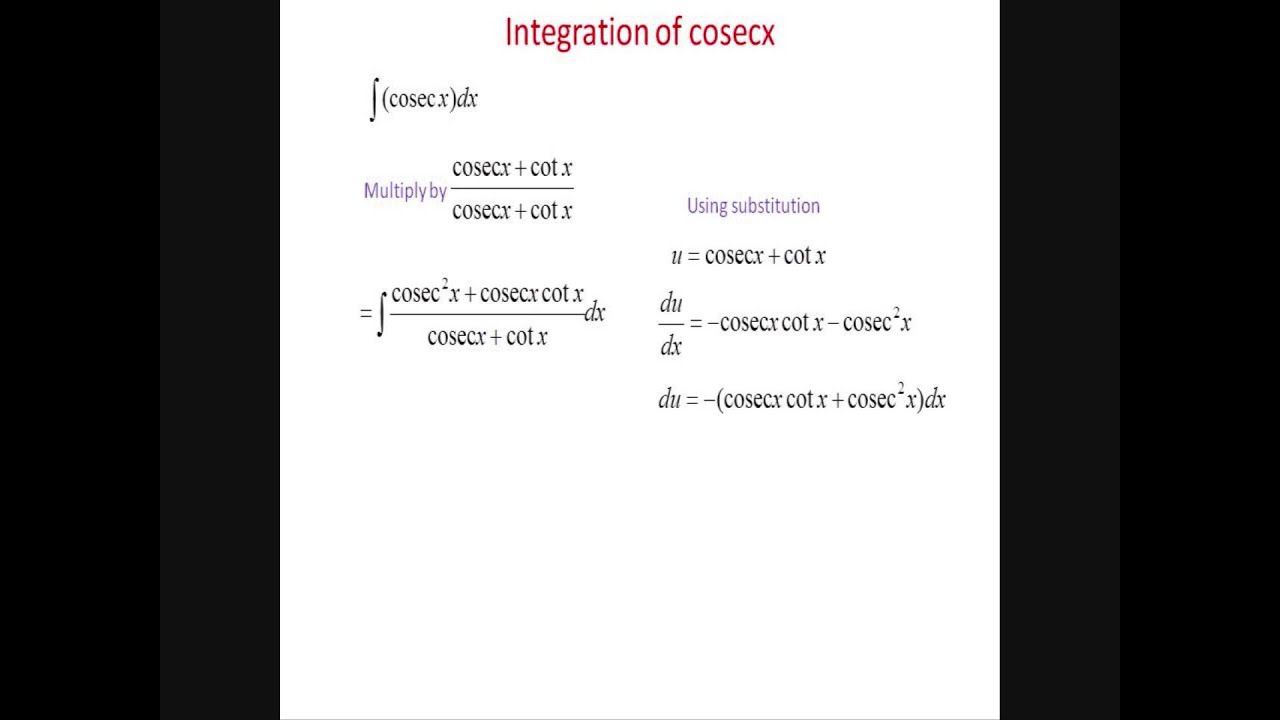
Your email address will not be published. The integral of the cosecant of angle x with respect to x is equal to the natural logarithm of the subtraction of cot of angle x from cosecant of angle x, and. U = cscx −cotx ⇒ du dx = −cscxcotx + csc2x, and so our integral becomes: = ln|cscx − cotx| + c.

1) cot x + (cot3 x / 3) + c.

The integration of cosecant cotangent is of the form. The integral of cosec x canbe determined by substitution method. This video shows the solution to the problem integration of cosecx cotx dx.please subscribe to this channel for more math help, a. ∫ 1 + cot x c o s e c 2 x d x.

The purpose of making this change will become apparent in the next two steps.

Hence, we get a new integration expression on the rhs, that means the same thing as the lhs. The integral of product of cosecant and cot functions formula can be written in terms of any variable in integral calculus. We know that integral of sum of functions equals sum of integrals of the functions taken separately. As you can see, sec 2 x dx are the same terms in our integration problem, hence we can.