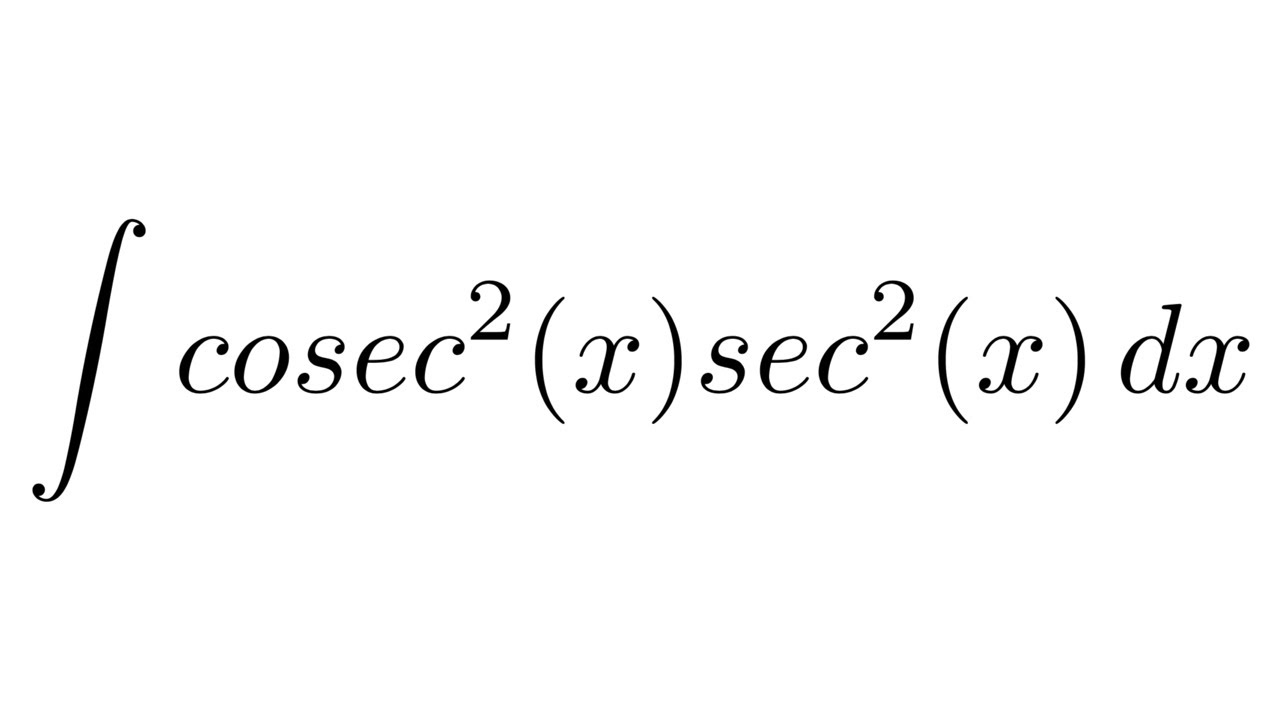∫ 1 + c o s 2 x 1 hard. X 2 × 1 cos 2. In this method of integration by substitution, any given integral is transformed into a simple form of integral by substituting the independent variable by others.
sec^2(x) li integraller, cosec^2(x) li integraller
⇒ dy dx = − 2cotxcsc2x.
U = cos x d u = − sin x d x d v = cos x sin 2 x d x v = − 1 sin x.
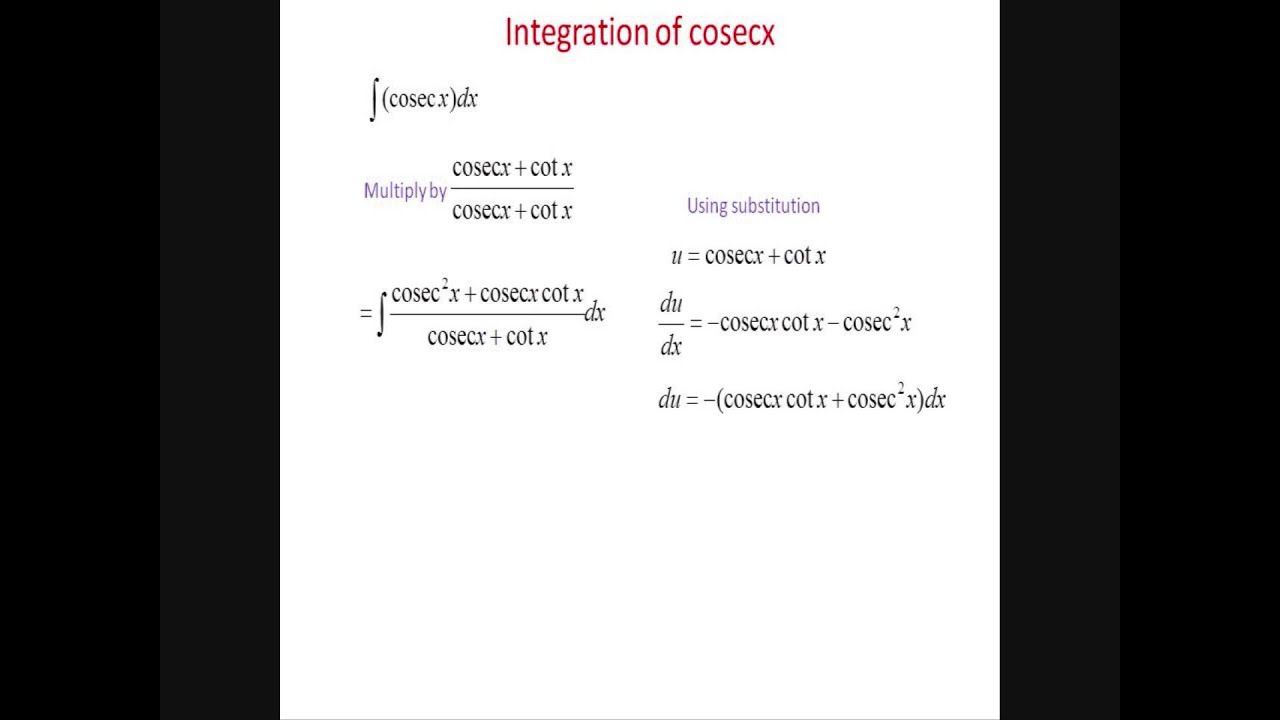
Let i = \(\int\) cosec x dx. Any help would be greatly appreciated thanks First, the only singularity would be z = 0, but it is only an apparent singularity so the residue is 0. So far iv tried a few different things.
I no that cosec^2=1/sin^2x but this doesnt help much.
Lets start the integration of cosec 2x. Using these facts and formulas of trigonometry, we have. How to find the integral of cosec x by substitution? Integration of cosecant squared of x is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae.
To integrate cot^2x, also written as ∫cot2x dx, cot squared x, (cot x)^2, and cot^2(x)we start by using standard trig identities to simplify the integral to a form we can work with.
⇒ dy dx = − 2(sinx)−3 × d dx (sinx) ⇒ dy dx = − 2cosx sin3x. View solution > view more. The integration of cosecant squared of x is of the form. Differentiate using the chain rule.
Integrate by parts and simplify:
Where c is the integration constant. X d x = − cot. The value of ∫ − π / 2 π / 2 [c o t − 1 x] d x (where [.] denotes greatest integer function ) is equal to view solution evaluate the following integral: So final expression for integration is ∫ (cosec 2 x) dx = ∫ (sec 2 x / tan 2 x) dx.
X d x or ∫ cosec 2.
To prove this formula, consider. ⇒ dy dx = − 2 × cosx sinx × 1 sin2x. Given y = f (g(x)) then. ∫ sin x 2 d x.
We recall the standard trig identity for cosecx, and square both sides.
∫ cos 2 x sin 2 x d x + ∫ d x = − cos x sin x − ∫ − sin x − sin x d x + ∫ d x = − cot x + c. Memorization tricks > mindmap > important diagrams > common misconceptions > problem solving tips > I would like to evaluate it via contour integration (the path is a semicircle in the upper plane), but i have some problems: We divide the numerator and denominator by cos squared x.
Edited may 27 '14 at 20:46.
We will get cosec 2 x = sec 2 x / tan 2 x. 2 x − 1 x 2 d x. Dy dx = f '(g(x)) × g'(x) ← chain rule. View chapter > shortcuts & tips.
Couldn't get much further with that.
Consider the following improper integral: Ex 7.1, 19 sec 2 2 dx sec 2 2 = 1 cos 2 1 sin 2 = 1 cos 2 sin 2 1 = sin 2 cos 2 = tan 2 = sec 2 1 = sec 2 Let us assume that log. Using this formula of trigonometry, we can evaluate the integral of cot^2x.
Integrate the following functions w.r.t.
The integral of cosec x canbe determined by substitution method. Where c is the integration constant. Prepare for integration by parts: Learn integral of square of secant function with introduction and proof for integration of sec²(x) rule with respect to x to prove ∫sec²xdx = tanx+c.
Show activity on this post.
To integrate cosec^2x, also written as ∫cosec 2 x dx, cosec squared x, cosec^2 (x), and (cosec x)^2, we start by using standard trig identities to simplify the integral. Integrate 1 − x 2 x w.r.t x medium. The indefinite integral of cosecant squared of angle x function with respect to x is equal to sum of the negative cotangent of angle x and a constant of integration. Here y = csc2x = 1 sin2x = (sinx)−2.
Follow this answer to receive notifications.
Lets assume u = tanx then du/dx = sec 2 x. We rearrange the pythagorean for cos2x so that we can substitute it into our previous trig identity.