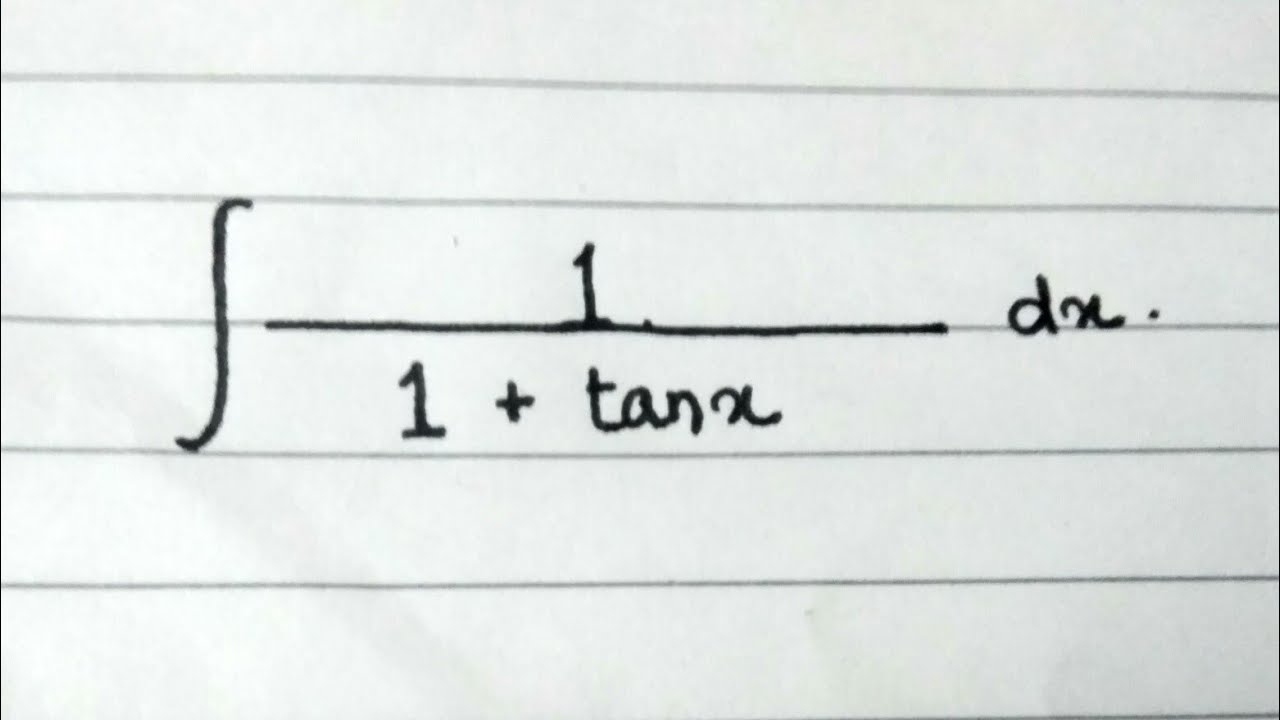Integration Of 1tanx Dx `int (tan^(1)x)dx` YouTube

Alternatively, a similar approach taken to the one in hrishabh's answer seems to work out much better: Dear student, please find below the solution to the asked query : What is the integral root of cosx?

`inte^(x)(1tanx)secx dx` is equal to YouTube

To solve such integrals involving trigonometric terms in numerator and denominators.

= ∫ cosx sinx dx.

∫ 1 + x 2 1 − x dx. We use the basic substitution method and to apply this simply we follow the undermentioned procedure. = ∫ 1 u du. I = ∫ 0 π / 4 l o g ( 1 + 1 − t a n x 1 + t a n x) d x.

Ridhi arora, tutorials point india private li.

∫ 1 tanx dx = ∫cotxdx. Question bank, mock tests, exam papers. If not, you won't be able to do the second one. Limit asintegration of 1 to 2 (3x^2+5x)dx;

What is the integral of root tanx?

Then the following integral reduces to { 1/ [ (1+t) (1+2t)] }/2 on adding and subtracting 't' and rearranging a bit on the numerator the following integral reduces. Stack exchange network consists of 178 q&a communities including stack overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. $$\int (1+ \tan x).\tan (x+a).dx=\int \tan (x+a).dx + \int \tan x.\tan (x+a).dx$$ the first integral should be easy. Ex 7.2, 33 integrate 1/ (1 − 𝑡𝑎𝑛⁡𝑥 ) ∫1 1/ (1 − tan⁡𝑥 ) 𝑑𝑥 = ∫1 1/ (1 − sin⁡𝑥/cos⁡𝑥 ) 𝑑𝑥 = ∫1 1/ (〖cos⁡𝑥 − sin〗⁡𝑥/cos⁡𝑥 ) 𝑑𝑥 = ∫1 cos⁡𝑥/〖cos⁡𝑥 − sin〗⁡𝑥 𝑑𝑥 = ∫1 (2 cos⁡𝑥)/2 (〖cos⁡𝑥 − sin〗⁡𝑥 ) 𝑑𝑥 = ∫1 (cos⁡𝑥 + cos⁡𝑥)/2 (〖cos⁡𝑥 − sin〗⁡𝑥 ) 𝑑𝑥 = ∫1 (cos⁡𝑥 + cos⁡𝑥 + sin⁡𝑥 − sin⁡𝑥)/2 (〖cos⁡𝑥 − sin.

Christopher lucas, added an answer, on 24/1/18.

Statement i the value of the integral ∫(x → pi/3,pi/6)dx/(1 + √tanx) is equal to pi/6. Let u = sinx, so du = cosxdx to get. \displaystyle\int\dfrac{1}{1 + \tan x}\,dx =\displaystyle\int\dfrac{1}{1 + \dfrac{\sin x}{\cos x}}\,dx =\displaystyle\int\dfrac{\cos x}{\cos x. X d x = ∫ 0 ∞ 1 ( 1 + u) ( 1 + u 2) d u.

Manbar singh, meritnation expert added an answer, on 17/10/15.

Since, this is a definite integral, to integrate it we have to use the following property of definite integrals. Let i = ∫ cos 2 x. ∫ (tanx) / (a+b tan 2 x) dx , plz answer asap, thank you. Find the integral of the function w.

1 tanx = cotx = cosx sinx.

Integration of 1/1+tanx *dx report ; Cbse > class 12 > mathematics 1 answers. Let i = ∫ 0 π 2 log ( tan x) ⋅ d x. First write down the whole fuction using sines and cosines, then you will notice that the you can con

Posted by roopan sandhu 3 years, 4 months ago.

Then let t = u where d t = 1 2 u d u and the integral becomes. Editor toolbars basic styles bold paragraph insert/remove numbered list insert/remove bulleted list insert image insert horizontal line insert special character tools maximize others wiris editor math wiris editor chemistry. The given integral is easy just multiply and divide by sec 2 x and put tan 2 x = t , which gives 2tanx*sec 2 xdx = dt. ∫_0^(𝜋/4) log⁡(1+tan⁡𝑥 ) 𝑑𝑥 let i=∫_0^(𝜋/4) log⁡〖 (1+tan⁡𝑥 )〗 𝑑𝑥 ∴ i=∫_0^(𝜋/4) log⁡[1+tan⁡(𝜋/4−𝑥) ] 𝑑𝑥 i=∫_0^(𝜋/4) log⁡[1+(tan⁡ 𝜋/4 −tan⁡𝑥)/(1 +〖 tan〗⁡ 𝜋/4.

Here, a = π 2.

I = ∫ 0 ∞ 2 t ( 1 + t) ( 1 + t 4) d t. From (i) we can substitute the value, we get. = ∫ 0 π 2 log ( cot x) ⋅ d. Click here👆to get an answer to your question ️ integrate:

To find the second integral, here's a hint.

If i has the form $\int\frac{asinx+bcosx+c}{dsinx+ecosx+f}$ dx. I = ∫ 0 π / 4 l o g ( 2 1 + t a n x) d x. Find the integral of root tanx? Which can then be solved by repeated partial fraction decomposition.