There are three common notations for inverse trigonometric functions. Cosec2 𝑥 𝑑𝑥 = − cot 𝑥 ∫ d u a 2 + u 2 = 1 a arctan u a + c.
12X1 T05 05 integration with inverse trig (2011)
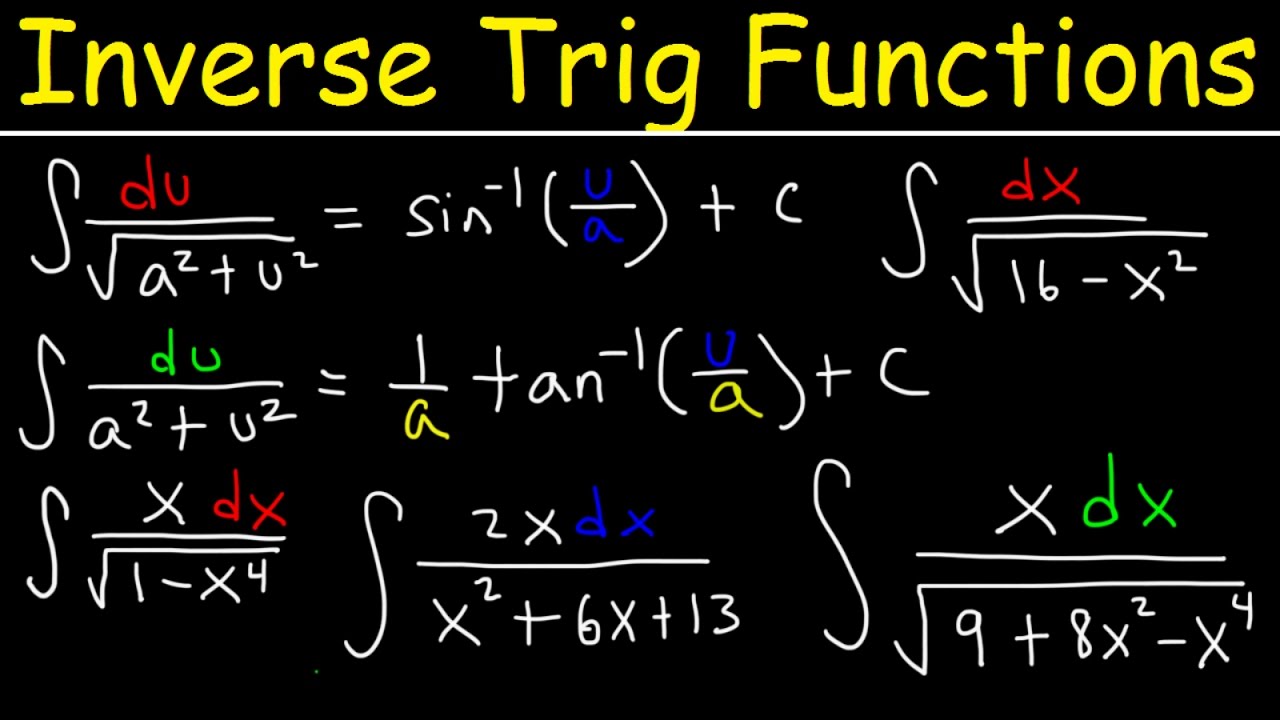
∫ du √a2−u2 = sin−1 u a +c ∫ d u a 2 − u 2 = sin − 1 u a + c ∫ du a2+u2 = 1 a tan−1 u a +c ∫ d u a 2 + u 2 = 1 a tan − 1 u a + c ∫ du u√u2−a2 = 1 a sec−1 u a +c ∫ d u u u 2 − a 2 = 1 a sec − 1 u a + c
∫sec x dx = ln|tan x + sec x| + c.
𝑥 𝑛+1 𝑥 𝑛 𝑑𝑥 = , 𝑛 ≠ −1 𝑛+1. Completing the square helps when quadratic functions are involved in the integrand. We mentally put the quantity under the radical into the form of the square of the constant minus the square of the variable. Sin 𝑥 𝑑𝑥 = − cos 𝑥.
Q.1 exercises , inverse trigonometric and hyperbolic functions%%%%%.
22 arcsin du u c au a ³ 2. Below are the list of few formulas for the integration of trigonometric functions: X d x = − cos. X d x = x sin − 1.
1 𝑑𝑥 = log 𝑥 𝑥.
Such that f (g (y))=y and g (f (y))=x. For example, the quadratic x2 + bx + c can be written as the difference of two squares by adding and. ∫tan x dx = ln|sec x| + c. ∫ d u u u 2 − a 2 = 1 a a r c s e c u a + c.
The only difference is whether the integrand is positive or negative.
U = x, a = 2. ∫ sin−1x dx= xsin−1x+√1−x2+c 1. Integral formulas involving inverse trigonometric functions can be derived from the derivatives of inverse trigonometric functions. In integration we basically take the infinitesimal data as the combination.
The integration formulas for inverse trigonometric functions can be disguised in many ways.
Unfortunately, this is not typical. 𝑒 𝑥 𝑑𝑥 = 𝑒 𝑥. ∫ d u a 2 − u 2 = arcsin u a + c, a > 0. However, only three integration formulas are noted in the rule on integration formulas resulting in inverse trigonometric functions because the remaining three are negative versions of the ones we use.
D dx arcsin x 1 1 x2 theorem 5.17 integrals involving inverse.
To determine the sides of a triangle when the remaining side lengths are known. This calculus video tutorial focuses on integration of inverse trigonometric functions using formulas and equations. D dx arccos x 1 1 x2. X d x = sin.
Example of inverse trigonometric functions:
The arcsine function, for instance, could be written as sin −1 , asin , or, as is used on this page, arcsin. 142 dx x ³ 2. Using the substitution however, produces with this substitution, you can integrate as follows. Integration 381 example 2 integration by substitution find solution as it stands, this integral doesn’t fit any of the three inverse trigonometric formulas.
The following integration formulas yield inverse trigonometric functions:
Integration formula of the trigonometric function. Integration of inverse trigonometric functions problems and solutions. Cos 𝑥 𝑑𝑥 = sin 𝑥. In a simple straight language integration can be defined as the measure, which basically assigns numbers to the several functions.the numbers are basically assigned which may describe the displacement,volume or area etc of such concerned function.
Integration of inverse trigonometric functions.
∫ 0 1 2 d x 1 − x 2 = sin −1 x | 0 1 2 = sin −1 1 2 − sin −1 0 = π 6 − 0 = π 6. 1 3 arcsec 2x 3 c u 2x, a 3 dx x 4x2 9 2 dx 2x 2x 2 32 1 3 2 arctan 3x 2 c u 3x, a 2 dx 2 9 x2 1 3 3 dx 2 23 dx 4 x2 arcsin x 2 c arcsin x 1 1 x2, arccos x. 22 1 arctan du u c a u a a ³ 3. D dx arccos x 1 1 x2.
D dx arcsin x 1 1 x2 theorem 5.17 integrals involving inverse.
5.9 lesson filled in.notebook february 21, 2014. There are six inverse trigonometric functions. 2 12 19 dx ³ x The integration formulas for inverse trigonometric functions can be disguised in many ways.
Using our knowledge of the derivatives of inverse trigonometric identities that we learned earlier and by reversing those differentiation processes, we can obtain the following integrals, where `u` is a function of `x`, that is, `u=f(x)`.
1 3 arcsec 2x 3 c u 2x, a 3 dx x 4x2 9 2 dx 2x 2x 2 32 1 3 2 arctan 3x 2 c u 3x, a 2 dx 2 9x2 1 3 3 dx 2 23x dx 4 x2 arcsin x 2 c arcsin x 1 1 x2, arccos x. For each inverse trigonometric integration formula below there is a corresponding formula in the list of integrals of inverse hyperbolic functions. Examples include techniques such as int. ∫cos x dx = sin x + c.
22 1 sec du u arc c u u a aa ³ why are there only three integrals and not six?
Integration of inverse trigonometric functions problems and. ∫ cosx dx = sinx+c 1. Below are some of the important formulas of inverse trigonometric functions in the integration. ∫ 0 1 2 d x 1 − x 2 = sin −1 x | 0 1 2 = sin −1 1 2 − sin −1 0 = π 6 − 0 = π 6.
Sec 2 𝑥 𝑑𝑥 = tan 𝑥.
Unfortunately, this is not typical. Up to 24% cash back section 5.7 inverse trigonometric functions: We can go directly to the formula for the antiderivative in the rule on integration formulas resulting in inverse trigonometric functions, and then evaluate the definite integral. X + 1 − x 2 + c.
#bsmaths #mscmaths #complexanalysis #elementaryfunctionschapter #3 elementary functions:
∫ sinx dx = −cosx+c 2.