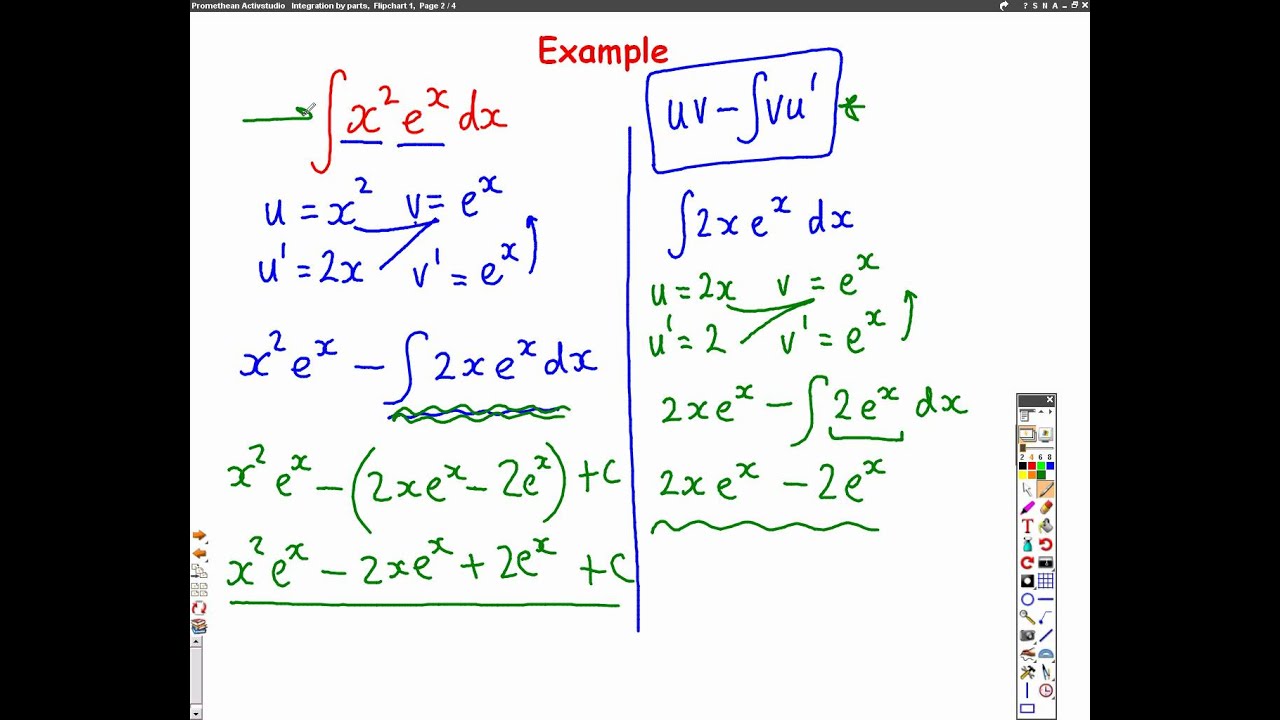
This is the currently selected item. Integration by parts practice problems. U = x, dv = ex dx 2) ∫xcos x dx;
Ap Calculus Calculus Problems Worksheet Ap Calculus
Solve the following integrals using integration by parts.
We need to apply integration by partstwicebeforeweseesomething:
Begin with the substitution w= p t.) 2. Z 2 1 (lnx)2 x3 dx= (lnx) 2x2 2 1 + z 2 1 lnx x3 dx do integration by parts again. This worksheet asks students to practice using integration by parts with four integrals. Math 114 worksheet # 1:
Let u= (lnx)2;dv = 1 x3 dx.
These worksheets precede gradually from some simple to complex exercises, to help students efficiently learn this complex topic of integration. Then du= 1 x dx;v= 1 2x2: By practicing maximum problems included in these worksheets students will gain the correct. Discover learning games, guided lessons, and other interactive activities for children
(a)let u= x3=2 +1 (b)then du= 3 2 x 1=2dxor 2 3 du= x dx (c)now substitute z x1 =2 p x3 +1 dx = z p x3=2 +1x1=2 dx = z p u 2 3 du = z 2 3 u1=2 du = 2 3 u3=2 2 3 +c = 4 9 u3=2 +c = 4 9 (x3=2 +1)3=2 +c 20.
∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1 3 x d x solution. Given r b a f(g(x))g0(x) dx, substitute u = g(x) )du = g0(x) dx to convert r b a f(g(x))g0(x) dx = r g( ) g( ) f(u) du. Using repeated applications of integration by parts: Let u= lnx;dv= 1 x3 dx.
Apply twice, start with g(x) = (ln(x))2 10.
Apply three times, start with g(x) = x3 8. Dv= sinxdx = du= dx; 5) ∫xe−x dx 6) ∫x2cos 3x dx 7) ∫ x2 e2x (4) x sec x tan x dx solution.
( 2 − 3 x) d x solution.
Z lnx x3 dx= lnx 2x2 2 1 + z 2 1 1 2x3 dx= lnx 2x2 1 4x2 2 1 plugging this into the original integral we get: Then du= 2lnx x dx;v= 1 2x2: Start of with integration by parts. Pick u to be the ’inside’ function
Which of the following integrals should be solved using.
∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts: Integrals evaluate the following inde nite integrals: We have z xsinxdx u= x; U and dv are provided.
Next use this result to prove integration by parts, namely that z u(x)v0(x)dx = u(x)v(x) z v(x)u0(x)dx.
Used by 10m students worldwide This is appropriate for ap calculus bc. Some of the below are integration by substitution worksheets, learn how to use substitution, as well as the other integration rules to evaluate the given definite and indefinite integrals with several practice problems with solutions. I pick the representive ones out.
U = x, dv = 2x dx 4) ∫x ln x dx;
Sometimes integration by parts must be repeated to obtain an answer. (6) x2 e 2x solution. Z p x q x p x+1 dx you should rewrite the integral as z x1 =2 p x3 +1 dx to help identify u. Ccdf add to my workbooks (0) embed in my website or blog add to google classroom
(1) u= ex dv= sin(x) du= exdx v= −cos(x) (2) u= ex dv= cos(x) du= exdx v= sin(x) exsin(x)dx= −excos(x)+ excos(x)dx = −excos(x)+ exsin(x)− exsin(x)dx = −excos(x)+exsin(x)− exsin(x)dx noticethatnowtheintegralweareinterestedin, exsin(x)dx,appearsonboththe.
Here is a set of practice problems to accompany the integrals involving trig functions section of the applications of integrals chapter of the notes for paul dawkins calculus ii course at lamar university. (5) 2 x e3x solution. Z 2 1 (lnx) x3 dx= (lnx) 2 2x2 lnx 2x2 1 4x2 2 1 = 1. The student is guided through the process of picking u, du, v, and dv.
Use the product rule to nd (u(x)v(x))0.
The solutions are not proven Ad parents nationwide trust ixl to help their kids reach their academic potential. Integration by parts date_____ period____ evaluate each indefinite integral using integration by parts. Ixl is easy online learning designed for busy parents.
You may also need to use substitution in order to solve the integral.) a) z (x+2)exdx b) z 2 1 x3 lnxdx c) z arcsinxdx d) z x3ex2 dx e) 1 0 y p 3y+1 dy f) cos tdt(hint:
Many exam problems come with a special twist. For some of you who want more practice, it™s a good pool of problems. Practice problems on integration by parts (with solutions) this problem set is generated by di. Apply twice, start with g(x) = x2 3.
A detailed answer key is provided.
Integration by parts is a method of breaking down equations to solve them more easily. U = x, dv = cos x dx 3) ∫x ⋅ 2x dx; (2) x cos x solution. V= cosxdx xcosx+ z cosxdx = xcosx+sinx notice that in the above, setting u= xyields du dx = 1 (i.e., du= dx), which is simpler
Apply twice, start with g(x) = x2 +1 6.
U = ln x, dv = x dx evaluate each indefinite integral.