( 2 − 3 x) d x solution. But do try without looking at them first, chances are you won’t get hints on your exam. Practice problems on integration by parts (with solutions) this problem set is generated by di.
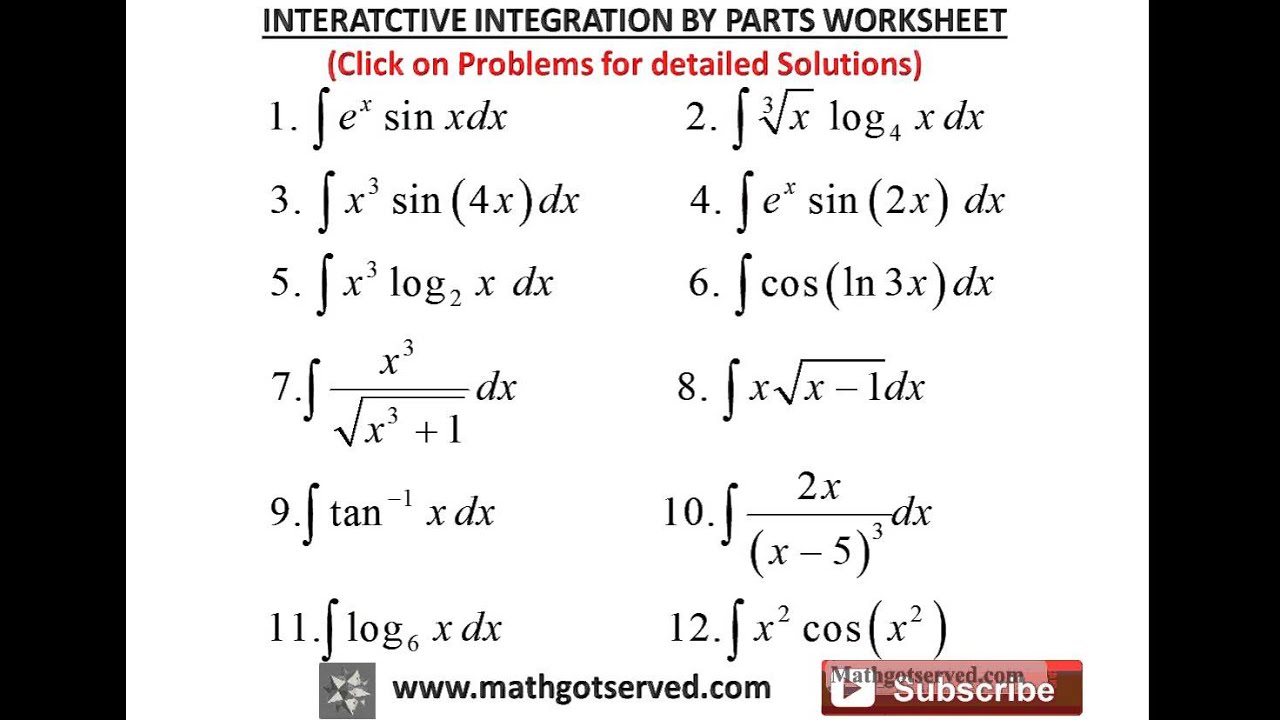
integration by parts interactive worksheet YouTube
Practice problems for class 12 maths chapter 7.
∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1 3 x d x solution.
Substituting u =2x+6and 1 2 The solutions are not proven Ill 161 find the exact value of x2ex dr. Then du= cosxdxand v= ex.
Solve the practice problem given below:
See also problem 1 in this quiz and the answer. Integration practice questions with solutions. Let u= cosx, dv= exdx. For some of you who want more practice, it™s a good pool of problems.
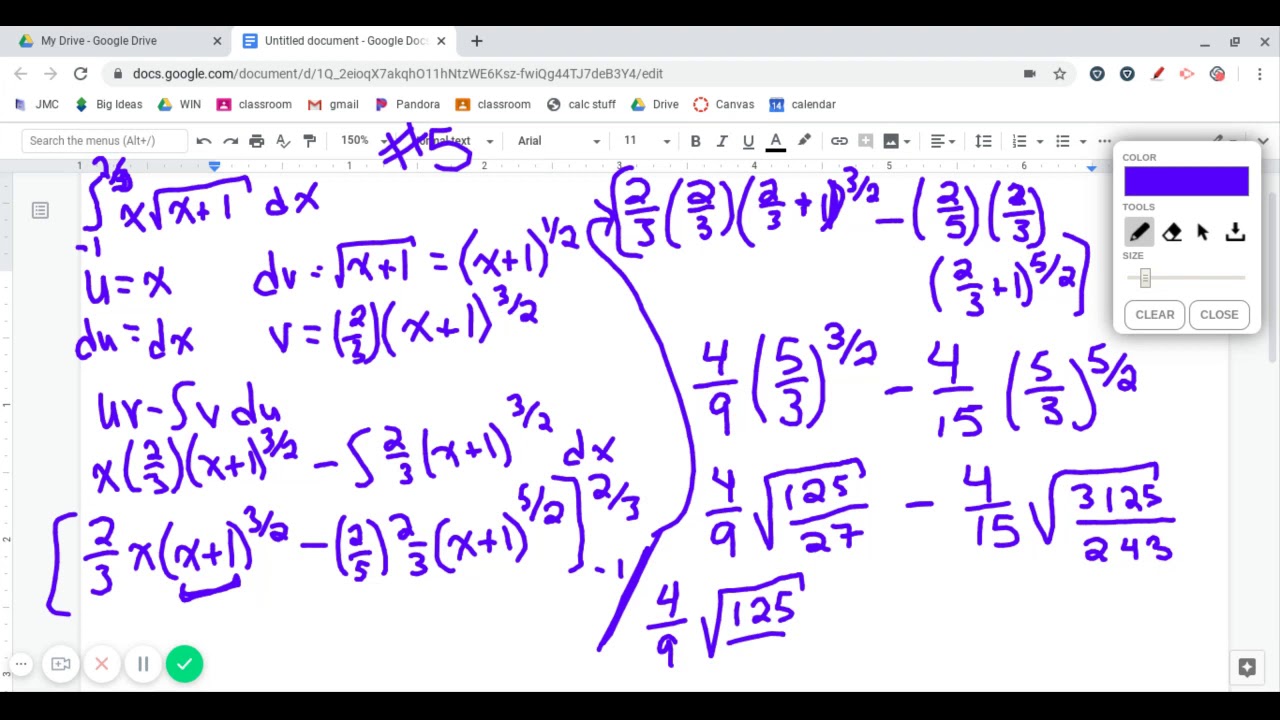
R udv = uv r vdu (try to substitute uso that du dx is simpler than uand so that vis no more complicated than dv.) example.
We have quizzes covering each and every topic of integration by parts. Study material for differentiation, differentiation test, differentiation solutions, differentiation test papers, integration, integration test, integration solutions covers algebra, circle, sphere, triangles, trigonometry, linear equations, quadratic. Integrate the function using integration by substution method: Let u= sinx, dv= exdx.
It is assumed that you are familiar with the following rules of differentiation.
Integrate the following with respect to x. Then du= sinxdxand v= ex. To play this quiz, please finish editing it. What is the integration by parts formula?
Evaluate the integral (cos 2x+ 2 sin 2 x)/cos 2 x;
Evaluate the integral using integration by parts. Here is a set of practice problems to accompany the integrals involving trig functions section of the applications of integrals chapter of the notes for paul dawkins calculus ii course at lamar university. Integration practice questions with solutions. V= cosxdx xcosx+ z cosxdx = xcosx+sinx notice that in the above, setting u= xyields du dx = 1 (i.e., du= dx), which is simpler
Many exam problems come with a special twist.
To play this quiz, please finish editing it. Questions and answers ( 3,913 ) quizzes (1) use integration by parts to integrate the following. Substitution and integration by parts ; Find ∫ (2x 2 +e x) dx.
Sometimes integration by parts must be repeated to obtain an answer.
Integration by parts with u = in(t + 2) and 6t2 to obtain f(t) ± ) in(t+2) — (dt) cao result from part (i) seen in integrand; We have z xsinxdx u= x; There are hints on the next page! Another (including some partial fractions) with answers.
[5] (i) use integration by parts to find x sec2x dr.
15 attempts made on this topic. Find ∫ [ (x 3 +3x+4)/√x] dx. Find ∫sin 2x cos 3x dx. Using repeated applications of integration by parts:
(ii) hence find xtan2x dr.
Evaluate ∫sec x (sec x+tan x) dx. This is the currently selected item. [4] [3] evaluate x cosx dx, giving your answer in an exact form [5] Dv= sinxdx = du= dx;
Find the integration of 2x/1+x 2.
∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts: The following are solutions to the integration by parts practice problems posted november 9. If you get stuck, don’t worry! ∫1/(1 + tan x) dx;
1)view solution 2)view solution 3)view solution 4)view solutionpart (a):
Different examples of integration questions are provided with step by step solution and you will learn how to solve integration questions using integration b. Then z exsinxdx= exsinx excosx z exsinxdx [6] find the exact value of x inxdx. (ii) hence use integration by parts to find the exact value of cosx sinx dr.
Then z exsinxdx= exsinx z excosxdx now we need to use integration by parts on the second integral.
Search by content rather than week number. I pick the representive ones out.