1 2 sin(2x) z ex. (6) x2 e 2x solution. Z sin 1(x) dx 2.
HOW TO DO INTEGRATION BY PARTS INTEGRAL OF X^2 LOGX
1 2 sin(2x)dx= exsin(2x) 2 + 1 2 z exsin(2x)dx we’ll.
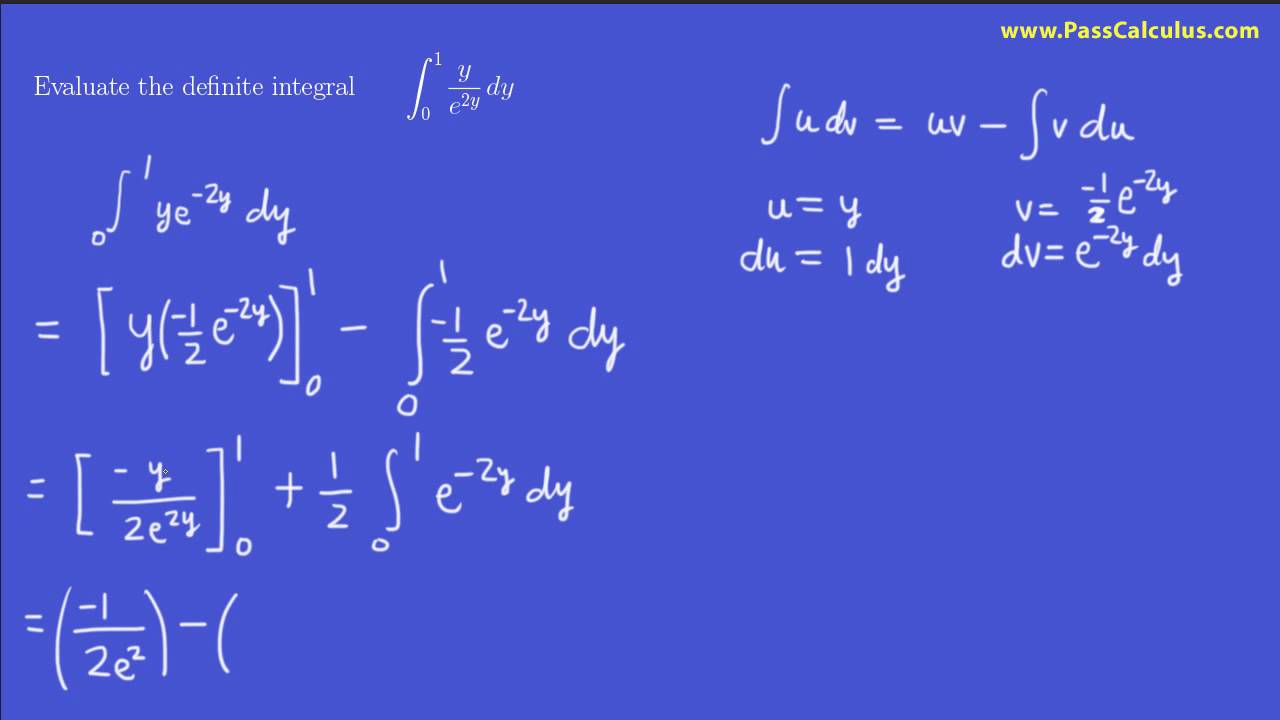
R udv = uv r vdu (try to substitute uso that du dx is simpler than uand so that vis no more complicated than dv.) example.
Level 5 challenges integration by parts find the indefinite integral 43 ∫ x ln x d x , \displaystyle{ 43\int{x \ln x dx} }, 4 3 ∫ x ln x d x. Evaluate inde nite integrals using integration by parts: \displaystyle \int x^ {3}\ln\ x\ dx ∫ x3ln x dx, using integration by parts. Practice problems on integration by parts (with solutions) this problem set is generated by di.
Let u= cosx, dv= exdx.
U = sin ( 1 4 z) → d u = 1 4 cos ( 1 4 z) d z d v = e 2 z d z → v = 1 2 e 2 z u = sin ( 1 4 z) → d u = 1 4 cos ( 1 4 z) d z d v = e 2 z d z → v = 1 2 e 2 z show step 5. If you get stuck, don't worry! Sometimes integration by parts must be repeated to obtain an answer. There are hints on the next page!
Of course, we are free to use different letters for variables.
7 practice problems concerning integration by parts 1. I pick the representive ones out. Z ex sin(x) dx 7. I hope you find this helpful!
The following are solutions to the integration by parts practice problems posted november 9.
*at first it appears that integration by parts does not apply, but let: For example, if , then the differential of is Written by victoria kala vtkala@math.ucsb.edu november 9, 2014 this is a list of practice problems for math 3b. (2) x cos x solution.
Then du= cosxdxand v= ex.
This is the currently selected item. We’ll now need to do integration by parts again and to do this we’ll use the following choices. Z xex ln(x 1) dx 3. Z ln(x) x2 dx 5.
Feel free to work with a group on any problem.
∫x 3 e^x 2 dx. U sin 1 x (inverse trig function) dv 1 dx. V= cosxdx xcosx+ z cosxdx = xcosx+sinx notice that in the above, setting u= xyields du dx = 1 (i.e., du= dx), which is simpler But do try without looking at them first, chances are you won't get hints on your exam.
Dv= sinxdx = du= dx;
After each application of integration by parts, watch for the appearance of a constant multiple of the original integral. For some of you who want more practice, it™s a good pool of problems. It is important to read the next section to understand where this comes from. Let t = x 2 dt = 2x dx.
We will be using the later way of dealing with the limits for this problem.
If you get stuck, don’t worry! Many exam problems come with a special twist. (5) 2 x e3x solution. ∫x 3 e^x 2 dx = ∫x ⋅ x 2 e^x 2 dx.
There are hints on the next page!
These problems are intended to enhance your knowledge and give you something to bring a boring party back to life. We have z xsinxdx u= x; This method uses the fact that the differential of function is. (4) x sec x tan x dx solution.
Z ex cos(x) dx 5 challenge problems concerning integration by parts 1.
Let u and v be functions of t. Integration by parts practice problems. Using repeated applications of integration by parts: Then z exsinxdx= exsinx z excosxdx now we need to use integration by parts on the second integral.
Z x2 sin(x) dx 6.
For example, if , then the differential of is. The method of integration by parts all of the following problems use the method of integration by parts. U= exdv= cos(2x)dx du= exdx v=1 2sin(2x) integrating by parts, z excos(2x)dx= ex. Z x4 tan 1(x) dx 5.
Then z exsinxdx= exsinx excosx z exsinxdx
∫ x 3 ln x d x. Plugging these into the integral from step 3 gives, The integration by parts equation comes from the product rule for derivatives. (a) neither term will get simpler through di erentiation, so let’s try some choice for uand dv, and see how it works out (we can always go back and try the other choice if it does not).
X dx = dt/2 = ∫ t e t (dt/2) = (1/2) ∫ te t dt.
Let u= sinx, dv= exdx. Then du= sinxdxand v= ex. The solutions are not proven But do try without looking at them first, chances are you won’t get hints on your exam.
∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts:
So, plugging u u, d u d u, v v and d v d v into the integration by parts formula gives, ∫ x 2 cos ( 4 x) d x = 1 4 x 2 sin ( 4 x) − 1 2 ∫ x sin ( 4 x) d x ∫ x 2 cos ( 4 x) d x = 1 4 x 2 sin ( 4 x) − 1 2 ∫ x sin .