Z x2 sin(x) dx 6. Z tan 1(x) dx 3. U = ln x, dv = x dx 2x 3 2 ln x 3 − 4x 3 2 9 + c
Pdf of integration by part
Z x3 p x2 +1 dx (a)let u= x 2 1.
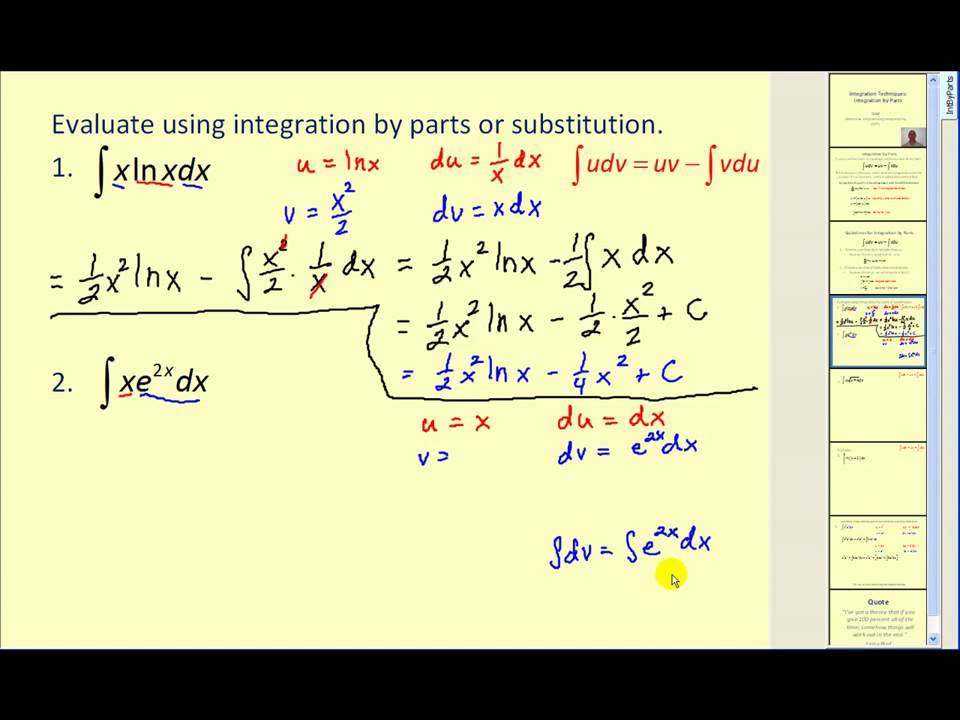
[6] find the exact value of x inxdx.
Evaluate inde nite integrals using integration by parts: Integral version of the product rule, called integration by parts, may be useful, because it interchanges the roles of the two factors. 7 practice problems concerning integration by parts 1. ∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application.
Ill 161 find the exact value of x2ex dr.
(e) z sin(p x) dx. In this tutorial, we express the rule for integration by parts using the formula: Sometimes integration by parts must be repeated to obtain an answer. To see this, make the identifications:
[5] (i) use integration by parts to find x sec2x dr.
2.express in simpli ed form the value of z e 1 2x4 ln(x) dx. Tabular method makes it rather quick: Z u dv dx dx = uv − z du dx vdx but you may also see other forms of the formula, such as: [4] [3] evaluate x cosx dx, giving your answer in an exact form [5]
Z ln(x) x2 dx 5.
Apply three times, start with g(x) = x3 8. Z p x q x p x+1 dx you should rewrite the integral as z x1 =2 p x3 +1 dx to help identify u. We can use integration by parts on this last integral by letting u= 2wand dv= sinwdw. Then use the formula z u dv dx dx = uv − z v du dx dx :
Apply twice, start with g(x) = (ln(x))2 10.
Many exam problems come with a special twist. U = x, dv = ex dx xex − ex + c 2) ∫xcos x dx; Integration by parts (section 2) version a rensselaer calculus skills practice page no calculators will be allowed and no partial credit will be given. Tabular integration by parts [see for example, g.
∫xexdx, ∫xxsin d, ∫xxln dx, etc.
The solutions are not proven U = g(x) and v = f(x). 1.express the inde nite integral z 2 ln(x) dx in terms of elementary functions. Z 2wsinwdw= 2sin 1 xcos(sin 1 x) + 2sin(sin 1 x) or you can look at the triangle formed by our substitution for w.
For some of you who want more practice, it™s a good pool of problems.
(b) z x sin(x) dx. From the product rule for differentiation for two functions u and v: Inverse trig logarithm algebraic (polynomial) trig exponential 2. Z f(x)g(x)dx = f(x)g(x)− z f(x) dg dx dx where df dx = f(x) of course, this is simply different notation for the same rule.
Using repeated applications of integration by parts:
Apply twice, start with g(x) = x2 +1 6. Substituting u =2x+6and 1 2 V= cosxdx xcosx+ z cosxdx = xcosx+sinx notice that in the above, setting u= xyields du dx = 1 (i.e., du= dx), which is simpler Use integration by parts to nd the following inde nite integrals.
Integrate by parts twice, and solve for i.
Example find z x2e3x dx. (7.16) xcosxdx d xsinx sinxdx Z sin 1(x) dx 2. (ii) hence find xtan2x dr.
U = x, dv = cos x dx xsin x + cos x + c 3) ∫x ⋅ 2x dx;
Logarithmic inverse trigonometric algebraic trigonometric exponential if the integrand has several factors, then we try to choose among them a which appears as high as possible on the list. Z ex cos(x) dx 5 challenge problems concerning integration by parts Z x cosxdx = x sinx− z (sinx)·1dx = xsinx +cosx+c where c is the constant of integration. (c) z e 1 ln(x) dx.
I pick the representive ones out.
Practice problems on integration by parts (with solutions) this problem set is generated by di. Integration by parts date_____ period____ evaluate each indefinite integral using integration by parts. We have z xsinxdx u= x; (a)let u= x3=2 +1 (b)then du= 3 2 x 1=2dxor 2 3 du= x dx (c)now substitute z x1 =2 p x3 +1 dx = z p x3=2 +1x1=2 dx = z p u 2 3 du = z 2 3 u1=2 du = 2 3 u3=2 2 3 +c = 4 9 u3=2 +c = 4 9 (x3=2 +1)3=2 +c 20.
Apply twice, start with g(x) = x2 3.
Ì4𝑥𝑒 7 ë > 5𝑑𝑥 5. Generally, picking u in this descending order works, and dv is what’s left: (d) z ex cos(x) dx. In the next example we will see that it is sometimes necessary to apply the formula for integration by parts more than once.
This is unfortunate because tabular integration by parts is not only a valuable tool for finding integrals but can also be applied to more advanced topics including the derivations of some important
Z 2wsinwdw= 2wcosw+ 2sinw at this point you can plug back in w: Z ex sin(x) dx 7. ∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1 3 x d x solution. Use the symbol c to denote an arbitrary constant.
R udv = uv r vdu (try to substitute uso that du dx is simpler than uand so that vis no more complicated than dv.) example.
(a) z (x2 + 2x)cosxdx: (ii) hence use integration by parts to find the exact value of cosx sinx dr. This is the currently selected item. Putting this all in 7.15:
First make a substitution, and then use integration by parts to nd the inde nite
By parts & by partial fractions integration by parts is used to integrate a product, such as the product of an algebraic and a transcendental function: Dv= sinxdx = du= dx; A mnemonic device which is helpful for selecting when using integration by parts is the liate principle of precedence for : U and dv are provided.
D uv udv vdu, and rewrite it as (7.15) udv d uv vdu in the case of 7.14, taking u x dv cosxdx, we have du dx v sinx.
R u dv = uv r v du. U = x, dv = 2x dx x ⋅ 2x ln 2 − 2x (ln 2)2 + c 4) ∫x ln x dx; Here is a set of practice problems to accompany the integration by parts section of the applications of integrals chapter of the notes for paul.