This article talks about the development of integration by parts: I add to both sides: Integration by parts, definite integrals.
PPT Sec 2.3 Modeling with First Order Equations
Identify and hierarchy the use the sound devices in literary works to attention mood, images, and meaning, including alliteration, onomatopoeia, and rhyme.
Oct 24, 2008 #1 shuttleman11.
Same deal with this short form notation for integration by parts. Www.mathcentre.ac.uk 5 c mathcentre 2009 For solving integrals like this, with two small power and large power, we must exchange parenthesis by substitution. A nice taxonomy of integration tricks, and integration by parts has its own corner cases such as using “i” and the “invisible dv” where dv = dx.
X is not, so let d v = x d x and then u = ln.
The trick i always use is, let dv be the function that has the cleanest antiderivative such that the order does not increase (unless using the “invisible dv”) i love this part of calculus 2. Start date oct 24, 2008; You also asked about integrating x ln. U is the function u(x) v is the function v(x) u' is the derivative of the function u(x)
Which second has power 50.
Sometimes integration by parts must be repeated to obtain an answer. We try to see our integrand as and then we have. Beside a call queue stats, there will uphold an icon that either says pause or enable. \liate and tabular intergration by parts 1.
Dividing both sides by 2 gives:
I = ∫ 12 ( u − 3 2) 2 u 50 d u 2 = 3 2 ∫ ( u 2 − 6 u + 9) u 50 d u = 3 2 ∫ ( u 52 − 6 u 51 + 9 u 50) d u. (fg)' = f'g + fg'. Many calc books mention the liate, ilate, or detail rule of thumb here. Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways.
This method is called ilate rule.
For instance i want to solve the integral. The integral of the two functions are taken, by considering the left term as first function and second term as the second function. You remember integration by parts. It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more.
A algebraic functions x, 3x2, 5x25 etc.
Then, z 1·ln|x|dx = xln|x|− z x· 1 x dx = xln|x|− z 1dx = xln|x|− x+c where c is a constant of integration. L logatithmic functions ln(x), log2(x), etc. This strategy methodology works in priority order by integration parts calculator. I = ∫ 12 x 2 ( 3 + 2 x) 50 d x.
Du and v are consequences of these two choices.
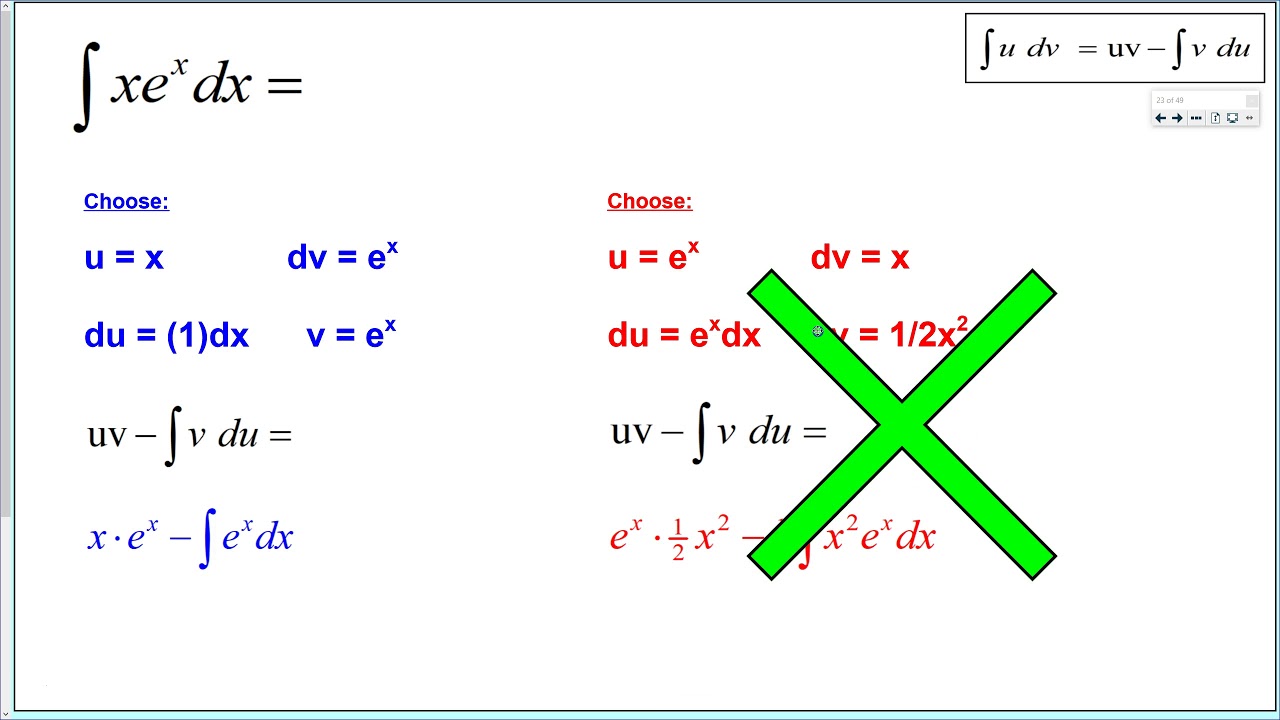
When using the integration by parts method you must choose u and dv; In calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of functions in terms of the integral of the product of their derivative and antiderivative. If u and v are functions of x, the product rule for differentiation that we met earlier gives us: This one a bit deeper:
Order of operations factors & primes fractions long arithmetic decimals exponents & radicals ratios & proportions percent modulo mean, median & mode scientific notation arithmetics.
Between x 2 and e x the factor e x is more sophisticated and you can integrate it, so let d v = e x d x and then u = x 2. So we will do the same to our integral equation, number [5]. D4 u / dx4 + k d2 u / dx2 + 6 = 0 0< x < 1 can someone help with this? For students the antiderivative of x is known but the antiderivative of ln.
You will see plenty of examples soon, but first let us see the rule:
These are supposed to be memory devices to help you choose your “u” and “dv” in an integration by parts question. 2 p = −q + r. We choose dv dx = 1 and u = ln|x| so that v = z 1dx = x and du dx = 1 x. ∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx.
Whichever function comes rst in the following list should be u:
We may be able to integrate such products by using integration by parts. So we have solved equation [5] for , giving us the desired result. In integration by parts, we have learned when the product of two functions are given to us then we apply the required formula. Liate an acronym that is very helpful to remember when using integration by parts is liate.
Then divide both sides by 2:
P = (− q + r )/2. Functions tan 1(x), sin 1(x), etc. Just as the substitution method of integration is, in a sense, the “unchain” rule, the method of integration by parts is the “unproduct rule.”. D/dx [f (x)·g (x)] = f' (x)·g (x) + f (x)·g' (x) becomes.
∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts:
With substitution 3 + 2 x = u and 2 d x = d u, the integral will simplify to. Integration by parts of 4th order de thread starter shuttleman11; Note that the uv|b a u v | a b in the first term is just the standard integral evaluation notation that you should be familiar with at this point. The tuitorial entails solving integrals using integration by parts.this metod of integration requires the first step to identify u & dv from the integral and this is achieved by applying the rule rule of the thumb using the acronym;liate whose letters represent five functions to.
We can use the formula for integration by parts to find this integral if we note that we can write ln|x| as 1·ln|x|, a product.
To solve this for p, we just add p to both sides: Using repeated applications of integration by parts: P = − q + r − p. Having difficulty integrating the following equation by parts to determine if its symmetric: