Integration by parts of uv formula. Let and be functions with continuous derivatives. Then, z 1·ln|x|dx = xln|x|− z x· 1 x dx = xln|x|− z 1dx = xln|x|− x+c where c is a constant of integration.
Integration by Parts [4, 9] (ln y)/(sqrt(y)) example 19
Even cases such as r cos(x)exdx where a derivative of zero does not occur.
The preference order of this rule will be dependent on some functions like inverse, algebraic, logarithm, trigonometric, exponent, according to the ilate rule.
The formula for integration by parts is then. We try to see our integrand as and then we have. The liate rule the di culty of integration by parts is in choosing u(x) and v0(x) correctly. Du and v are consequences of these two choices.
Let u = x then du = dx.
It is usually the last resort when we are trying to solve an integral. Let u = f (x) then du = f‘ (x) dx. Functions tan 1(x), sin 1(x), etc. Many calc books mention the liate, ilate, or detail rule of thumb here.
Integrating using linear partial fractions.
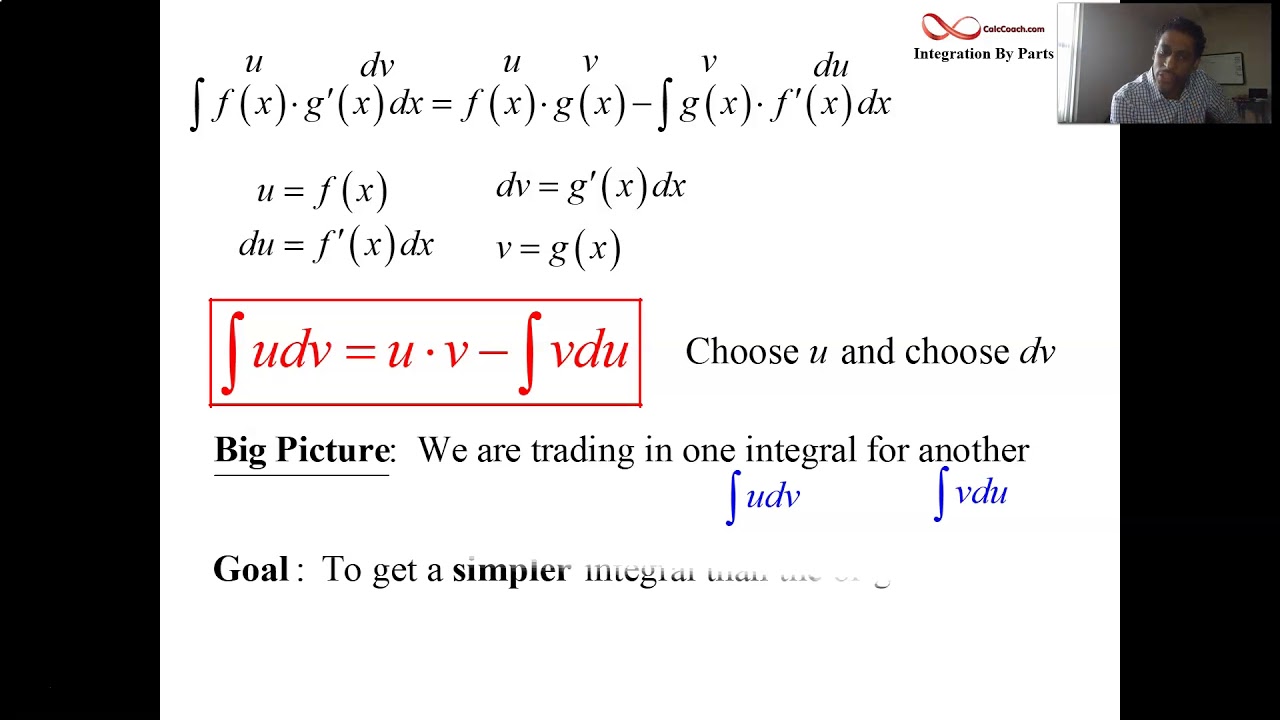
∫udv = uv − ∫vdu. You can nd many more examples on the internet and wikipeida. U = lnx (l comes before a in liate) dv = x3 dx du = x 1 dx v = ∫ = 4 4 x3dx x ∫∫x3 ln xdx = uv− vdu dx x x x x 1 4 4 (ln ) 4 4 = − ∫ x dx x = x − ∫ 3 4 4 1 4 (ln ) c x x x = − + 4 4 1 (ln ) 4 4 4 c x x x = − + 16 (ln ) 4 4 4 answer integration by parts For example, you asked about integrating x 2 e x.
We choose dv dx = 1 and u = ln|x| so that v = z 1dx = x and du dx = 1 x.
This method is used to find the integrals by reducing them into standard forms. Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. You remember integration by parts. As mentioned above, integration by parts uv formula is:
\liate and tabular intergration by parts 3 z x3 sin(x)dx = x3 cos(x) + 3x2 sin(x) + 6xcos(x) 6sin(x) + c:
Liate the word itself tells you in which order of priority you should use u(x). Applying the product rule to solve integrals. Let v = g (x) then dv = g‘ (x) dx. Choose as d v the most complicated expression in the integrand that you currently know how to integrate.
1) for how to use integration by parts and a good rule of thumb for choosing u a.
The following example illustrates its use. ∫ u d v = u v. L logatithmic functions ln(x), log2(x), etc. Integration by parts is what you use when you want to integrate the product of two functions.
You also asked about integrating x ln.
The integration by parts formula is. The product rule for derivatives is what you use when you want to differentiate the product of two functions. Integration by parts formula is used for integrating the product of two functions. Www.mathcentre.ac.uk 5 c mathcentre 2009
A algebraic functions x, 3x2, 5x25, etc.
L = logarithmic i = inverse trigonometric a = algebraic t = trigonometric Using the integration by parts formula. Liate the liate method was rst mentioned by herbert e. These are supposed to be memory devices to help you choose your “u” and “dv” in an integration by parts question.
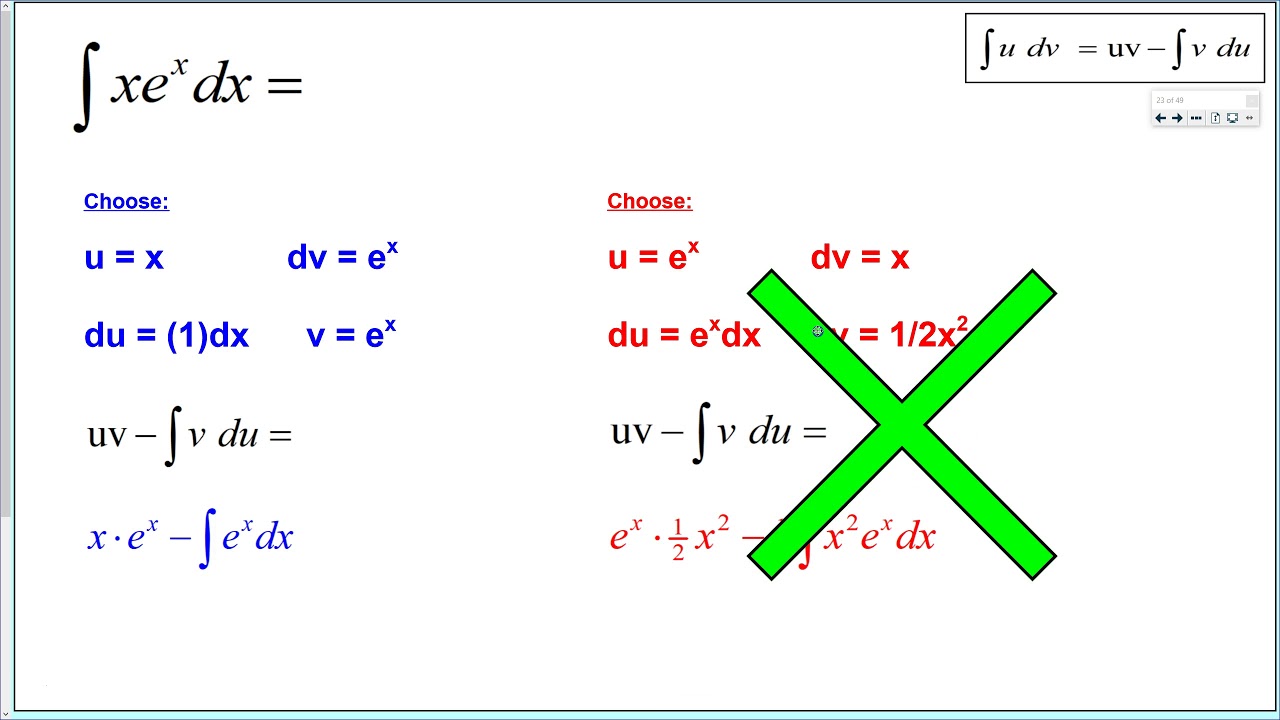
Between x 2 and e x the factor e x is more sophisticated and you can integrate it, so let d v = e x d x and then u = x 2.
Using the fact that integration reverses differentiation we'll arrive at a formula for integrals, called the integration. ∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx. You will see plenty of examples soon, but first let us see the rule: We can use the formula for integration by parts to find this integral if we note that we can write ln|x| as 1·ln|x|, a product.
The formula for integration by parts is.
With a bit of work this can be extended to almost all recursive uses of integration by parts. Mit grad shows how to integrate by parts and the liate trick. The liate rule is a rule of thumb that tells you which function you should choose as u(x): For example, if we have to find the integration of x sin x, then we need to use this formula.
When using the integration by parts method you must choose u and dv;
Just as the substitution method of integration is, in a sense, the “unchain” rule, the method of integration by parts is the “unproduct rule.”. U is the function u(x) v is the function v(x) u' is the derivative of the function u(x) The idea it is based on is very simple: It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more.
Obtain the integral through a straightforward integration of sin x.
Observation more information integration by parts essentially reverses the product rule for differentiation applied to (or ).: Since the function x is a polynomial, set u = x and d v = cos x. This is the currently selected item. \liate and tabular intergration by parts 1.
The function that appears rst in the following list should be u when using integration by parts:
This is the correct choice to make for integration by parts as d u = d x and v = sin x. So, we are going to begin by recalling the product rule. Integration by parts is a fancy technique for solving integrals.
![Integration by Parts [4, 9] (ln y)/(sqrt(y)) example 19](https://i.ytimg.com/vi/Ib1e9FTjHHE/maxresdefault.jpg)
