So for choosing which one to be first function we use ilate rule. In the integration by parts , the first two terms usually won't come together. A natural question would be how do i know which function should be \(u\) and \(dv\) in the substitution for integration by parts?
Integration by Parts [4, 9] (ln y)/(sqrt(y)) example 19
What is the rule of integration by parts?
The liate memory aid for integration by parts you now know what \(u\), \(v\), \(du\), and \(dv\) are.
It can be derived by integrating the product rule of differentiation (wiki) if u = f(x), v = g(x), and the differentials du = f ‘(x) dx and dv = g'(x) dx, then. Ilate rule is the most helpful rule used in integration by parts. Ilate rule is used in integration when we are doing integration by parts i.e when there is product of two functions and we have to integrate it. Instead of this rule, liate rule can also be applied.
Any one of the last two terms can be u, because both are differentiable and integrable.
In which the integrand is the product of two functions can be solved using integration by parts. It is used to represent those quickly oscillating integrals with sufficiently smooth integrands decay quickly. L logatithmic functions ln (x), log2 (x), etc. For those not familiar, liate is a guide to help you decide which term to differentiate and which term to integrate.
Integration by parts is often used in harmonic analysis, especially in fourier analysis.
The most common example of this is its use in showing that the decay of function’s fourier transform depends on the smoothness of that function. It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more. With a bit of work this can be extended to almost all recursive uses of integration by parts. Even cases such as r cos(x)exdx where a derivative of zero does not occur.
In calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of functions in terms of the integral of the product of their derivative and antiderivative.
The product rule for derivatives is what you use when you want to differentiate the product of two functions. ∫ u d v = u v. It’s a heuristic rule that helps transform the integral of products of functions into other integrals. The liate principle can help determine what to pick for \(u\) and \(dv\).the acronym liate stands for:
Using repeated applications of integration by parts:
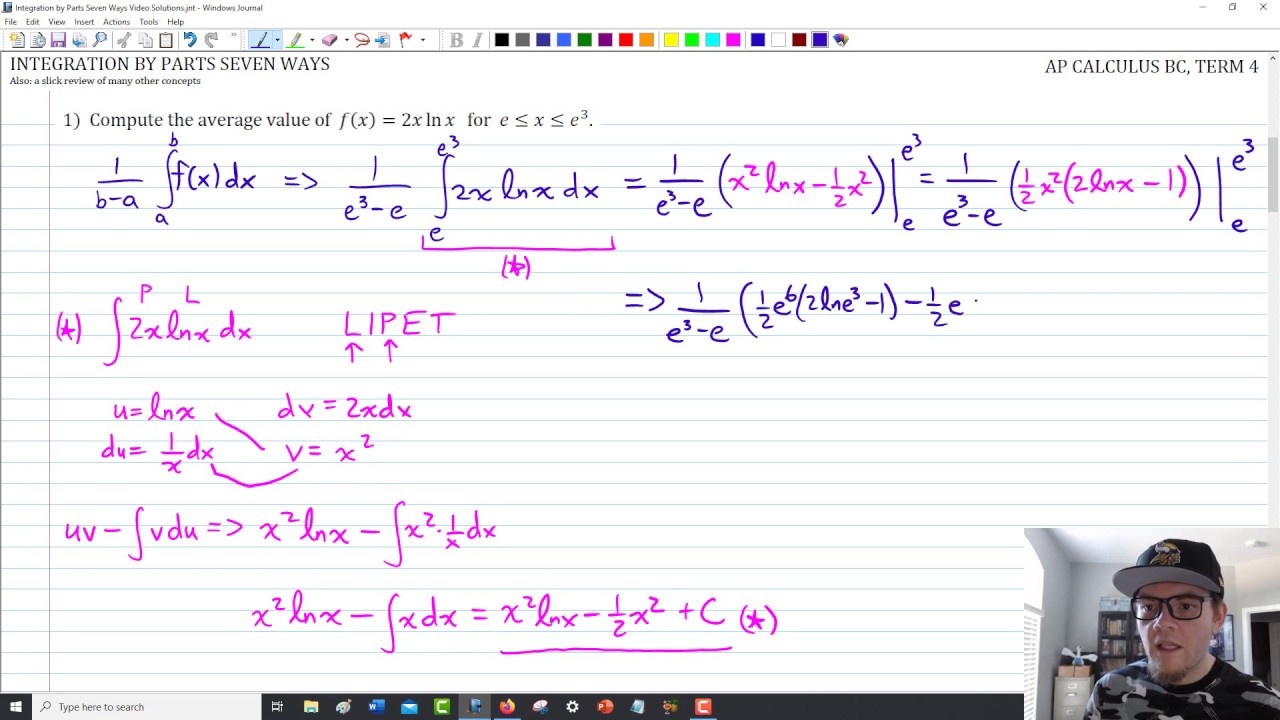
Let us learn more about ilate rule and its applications in detail along with more solved examples. ∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts: The function that appears rst in the following list should be u when using integration by parts: Liate is a term from mathematics, often called “integration by parts”.
In calculus, and more generally in mathematical analysis, integration by parts or partial integration is a process that finds the integral of a product of functions in terms of the integral of the product of their derivative and antiderivative.
The liate rule is a rule of thumb that tells you which function you should choose as u(x): This is the correct choice to make for integration by parts as d u = d x and v = sin x. Suppose that u(x) and v(x) are differentiable functions. What is liate rule in integration?
L = log, i = inverse trig, a = algebraic, t = trigonometric, e = exponential.
The integration by parts formula is. Following the liate rule, u = x and dv = sin (x)dx since x is an algebraic function and sin (x) is a trigonometric function. \liate and tabular intergration by parts 3 z x3 sin(x)dx = x3 cos(x) + 3x2 sin(x) + 6xcos(x) 6sin(x) + c: Du and v are consequences of these two choices.
Liate means logarithmic, inverse, algebraic , trigonometric and exponential.
Just as the substitution method of integration is, in a sense, the “unchain” rule, the method of integration by parts is the “unproduct rule.”. When using the integration by parts method you must choose u and dv; These are supposed to be memory devices to help you choose your “u” and “dv” in an integration by parts question. A algebraic functions x, 3x2, 5x25, etc.
You can nd many more examples on the internet and wikipeida.
This rule is used to decide which function is to be chosen as the first function when the integration is done by parts. It denotes the priorities to the functions. Many calc books mention the liate, ilate, or detail rule of thumb here. L logatithmic functions ln(x), log2(x), etc.
L = logarithmic i = inverse trigonometric a = algebraic t = trigonometric
Functions tan 1(x), sin 1(x), etc. An acronym that is very helpful to remember when using integration by parts is liate. Mit grad shows how to integrate by parts and the liate trick. Integration by parts is what you use when you want to integrate the product of two functions.
Liate the liate method was rst mentioned by herbert e.
Liate the word itself tells you in which order of priority you should use u(x). To calculate the integration by parts, take f as the first function and g as the second function, then this formula may be pronounced as: The next letter in lipet, a p, stands for polynomials. Obtain the integral through a straightforward integration of sin x.
Since the function x is a polynomial, set u = x and d v = cos x.
Either one can be taken as u in intg (u*δv). Sometimes integration by parts must be repeated to obtain an answer. The formula for integration by parts is. 1) for how to use integration by parts and a good rule of thumb for choosing u a.
The liate rule the di culty of integration by parts is in choosing u(x) and v0(x) correctly.
Whichever function comes first in the following list should be u: The term closer to e is the term usually integrated and the term closer to l is the term that is. \liate and tabular intergration by parts 1. This method is based on the product rule for differentiation.
You remember integration by parts.
![Integration by Parts from [0, 3] r^3 ln r dr example 20](https://i.ytimg.com/vi/7EbLsKaAR7I/maxresdefault.jpg)
![Integration by Parts [4, 9] (ln y)/(sqrt(y)) example 19](https://i.ytimg.com/vi/Ib1e9FTjHHE/maxresdefault.jpg)
