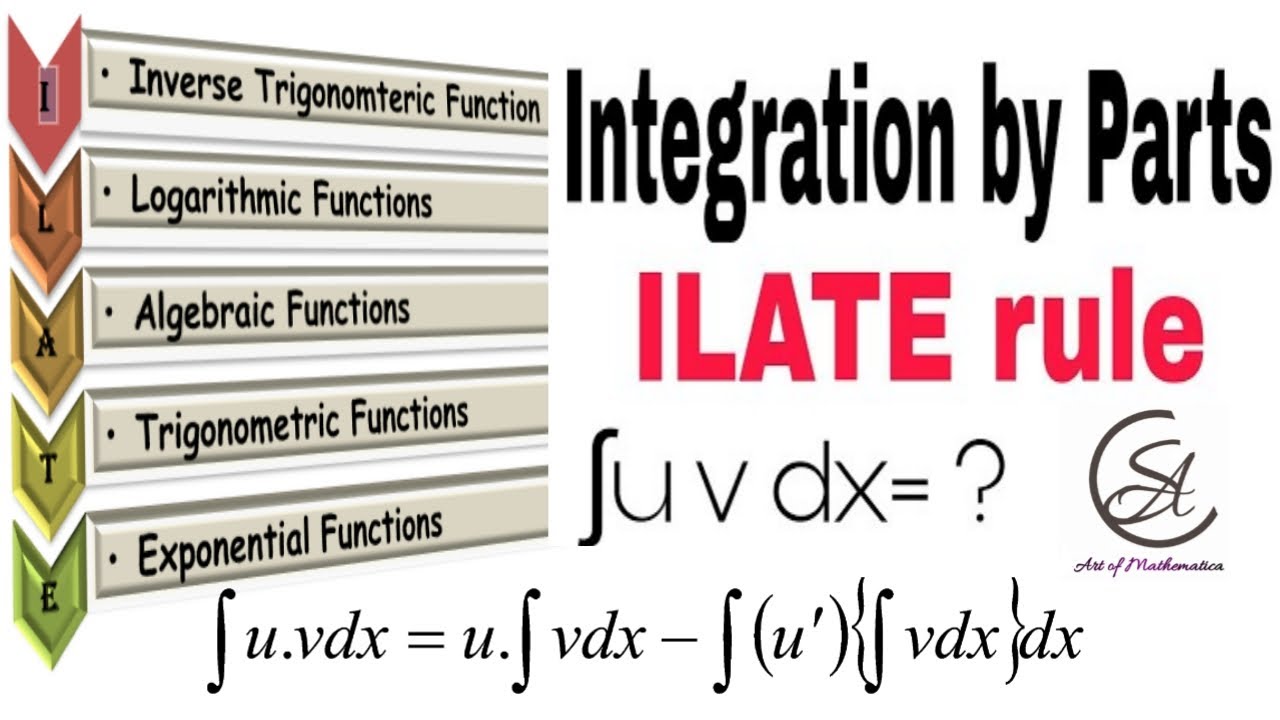
One term is called as u and another term is called as v. The acronym ilateis good for picking \(u.\) ilate stands for. Here you'll know the basic idea of ilate rule.
Integration Formulas Trig, Definite Integrals Properties
So for choosing which one to be first function we use ilate rule.
Liate the word itself tells you in which order of priority you should use u(x).
Let u = x and v = e x. The goal when using this formula is to replace one integral (on the left) with another (on the right), which can be easier to evaluate. Ilate rule is used in integration when we are doing integration by parts i.e when there is product of two functions and we have to integrate it. It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more.
For those not familiar, liate is a guide to help you decide which term to differentiate and which term to integrate.
∫ u v ′ d x = u v − ∫ u ′ v d x. In integration by parts, we get to know that the product of two functions is mentioned then we apply the required formula. ∫ u v dx = u ∫ v dx −. It is a very useful technique to find the integration of the product of two (or more) functions.
What is the rule for integration by parts?
The u and v terms are decided by liate rule. This is the integration by parts formula. So for choosing which one to be first function we use ilate rule. We try to see our integrand as and then we have.
For this, we can use the integration by parts formula ∫ u v d x = u ∫ v d x − ∫ [ d d x ( u) ∫ v d x] d x.
Ilate rule is used in integration when we are doing integration by parts i.e when there is product of two functions and we have to integrate it. Integration by parts is a procedure used to integrate and it is quite beneficial when two functions are multiplied together, but is also helpful in other ways. There are plenty of examples, but first, it is important to clear the rule: Tabular integration by parts when integration by parts is needed more than once you are actually doing integration by parts.
The integration by parts formula can be written in two ways:
Many calc books mention the liate, ilate, or detail rule of thumb here. It denotes the priorities to the functions. This method is called ilate rule. It denotes the priorities to the functions.
Sometimes, it is also referred by the name ‘partial integration’.
This method is called the ilate rule. I = ∫ x e x d x = x ∫ e x d x − ∫ [ d d x ( x) ∫ e x d x] d x. The liate method was rst mentioned by herbert e. L = log, i = inverse trig, a = algebraic, t = trigonometric, e = exponential.
Definite integration by parts is similar to integration by parts of indefinite integrals.
The ilate rule helps us make use of it in the correct way. You will see plenty of examples soon, but first let us see the rule: Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. In integration by parts, generally, we use the preference order for deciding the first function, using ilate rule (inverse trigonometric functions, logarithmic functions, algebraic functions, trigonometric functions, exponential functions).
When doing integration by parts, i know that using liate can be a useful guide most of the time.
This rule states that the inverse trigonometric function should be assumed as the first function while. Integration by parts is a powerful tool avaliable to us in calculus. Definite integration by parts is used when the function is a product of two terms of the independent variable. In mathematics, integration by part basically uses the ilate rule that helps to select the first function and second function in the integration by parts method.
U is the function u (x)
2 \liate and tabular intergration by parts and so z x3ex2dx = x2 1 2 ex2 z 1 2 ex22xdx = 1 2 x2ex2 z xex2dx = 1 2 x2ex2 1 2 ex2 + c = 1 2 ex2(x2 1) + c: L = logarithmic i = inverse trigonometric a = algebraic t = trigonometric The integral of the two functions are taken, by considering the left term as first function and second term as the second function. The formula of integration by parts was proposed by brook taylor in 1715.
These are supposed to be memory devices to help you choose your “u” and “dv” in an integration by parts question.
The liate rule is a rule of thumb that tells you which function you should choose as u(x): That’s why integration by parts is also called as ‘ the product rule of integration ’. The key thing in integration by parts is to choose \(u\) and \(dv\) correctly. From the ilate rule, we have the first function = x and the second function = e x.
The term closer to e is the term usually integrated and the term closer to l is the term that is.
The liate rule the di culty of integration by parts is in choosing u(x) and v0(x) correctly. Ilate rule is a rule that is most commonly used in the process of integration by parts and it makes the process of selecting the first function and the second function very easy. The integral of the two functions are taken, by referring to the left term as the main function and the second term as the second function. In integration by parts, we have learned when the product of two functions are given to us then we apply the required formula.