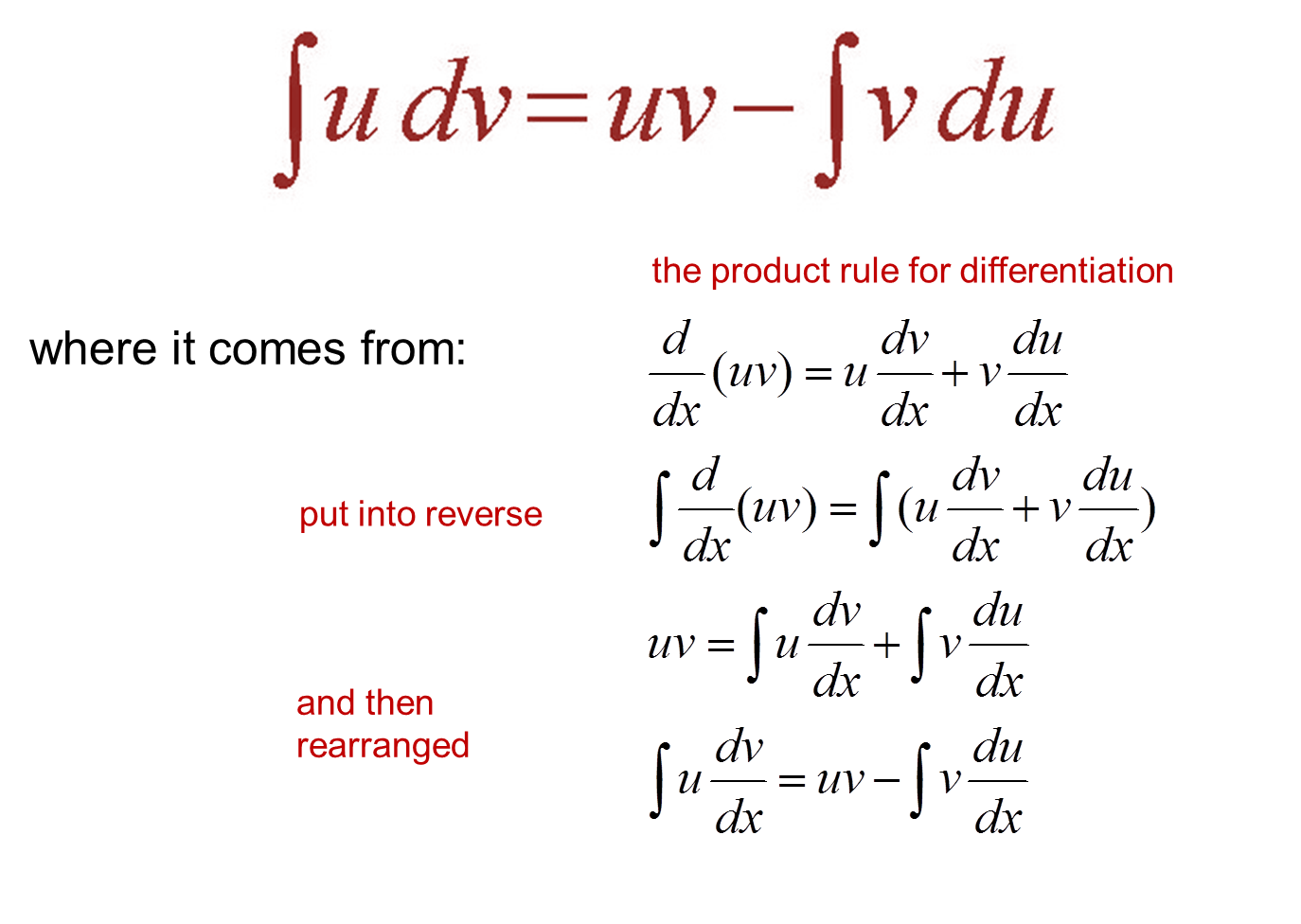U is the function u(x) The formula for integration by parts is given as: This article talks about the development of integration by parts:
PPT 6.1 Integration by parts PowerPoint Presentation
Www.mathcentre.ac.uk 5 c mathcentre 2009
This tutorial runs through an integration by parts example.this video is part of a full free calculus 2 course.
Integration by parts shortcut formula: D d x ( u v) = u d v d x + v d u d x. ∫ b a udv = uv|b a −∫ b a vdu ∫ a b u d v = u v | a b − ∫ a b v d u. Integration by parts, definite integrals.
We can integrate by parts on a definite integral, remembering to evaluate limits on each term.
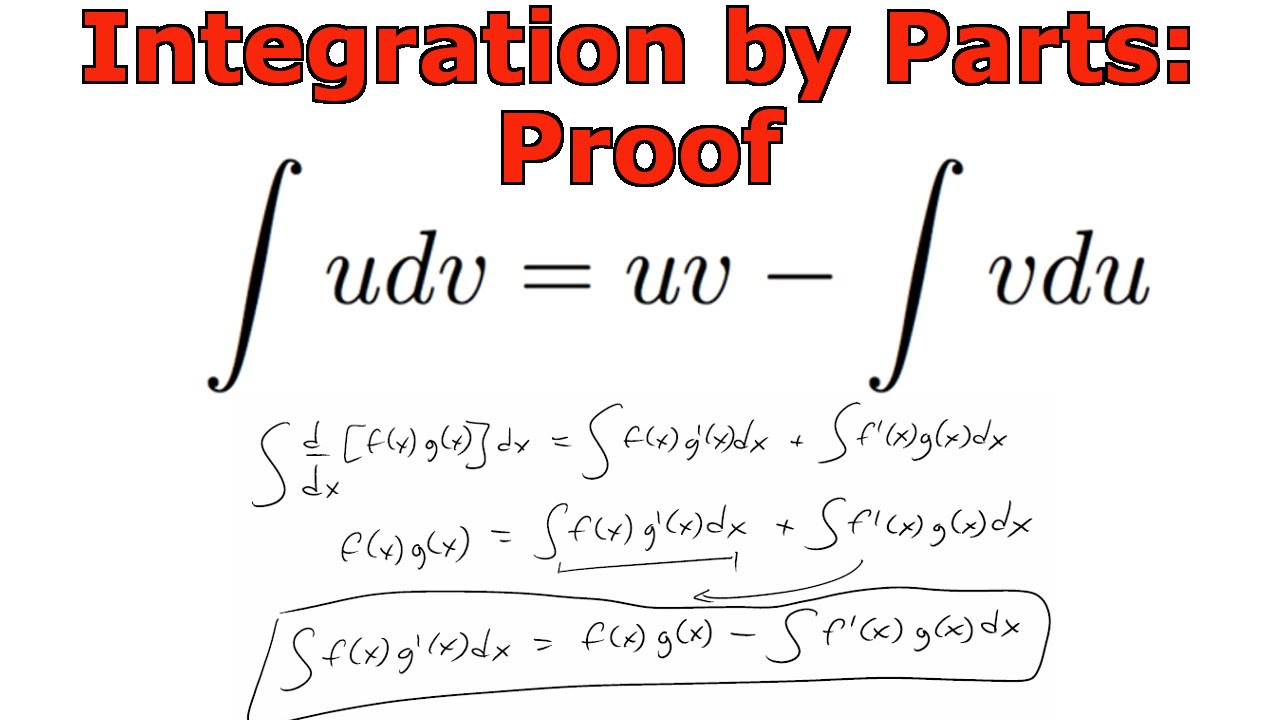
Same deal with this short form notation for integration by parts. Product rule of differentiation $f{(x)}$ and $g{(x)}$ are two functions in terms of $x$. The mathematical formula for the integration by parts can be derived in integral calculus by the concepts of differential calculus. (3) for example, consider the integral intxcosxdx and let u=x dv=cosxdx.
∫ u v d x = u d x − ∫ ( d u d x ∫ v d x) d x.
Learn more about the proof, applications of integration by parts formula. If u and v are any two differentiable functions of a single variable x (say). Integrating both sides, we get, u v = ∫ u d v d x d x + ∫. ∫uv dx = u∫v dx − ∫((du/dx)∫v dx) dx.
The formula is given by:
The method of integration using partial fractions. A single integration by parts starts with d(uv)=udv+vdu, (1) and integrates both sides, intd(uv)=uv=intudv+intvdu. Note that the uv|b a u v | a b in the first term is just the standard integral evaluation notation that you should be familiar with at this point. We can use the formula for integration by parts to find this integral if we note that we can write ln|x| as 1·ln|x|, a product.
The method of integration by substitution.
You will see plenty of examples soon, but first let us see the rule: U is the first function of x: There are two more methods that we can use to perform the integration apart from the integration by parts formula,. Then, by the product rule of differentiation, we have:
Next, work the right side of the equation out to simplify it.
Integration by parts is a procedure used to integrate and it is quite beneficial when two functions are multiplied together, but is also helpful in other ways. This one a bit deeper: D/dx [f (x)·g (x)] = f' (x)·g (x) + f (x)·g' (x) becomes. The course covers integration by table, integ.
∫u v dx = u∫v dx −∫u' (∫v dx) dx.
Then, z 1·ln|x|dx = xln|x|− z x· 1 x dx = xln|x|− z 1dx = xln|x|− x+c where c is a constant of integration. Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. So the integration by parts formula can be written as: The integration by parts formula states:
∫ u dv = uv − ∫ v du.
Integration by parts is the technique used to find the integral of the product of two types of functions. U(x) v is the second function of x: (fg)' = f'g + fg'. There are plenty of examples, but first, it is important to clear the rule:
∫ a b u ( x ) v ′ ( x ) d x = [ u ( x ) v ( x ) ] a b − ∫ a b u ′ ( x ) v ( x ) d x = u ( b ) v ( b ) − u ( a ) v ( a ) − ∫ a b u ′ ( x ) v ( x ) d x.
V(x) as the name suggests, in integration by parts, we first check which kind of two functions compose the given expression to. ∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx. Integration by parts is the inverse of the product rule for derivatives. To reverse the product rule we also have a method, called integration by parts.
As we know that, \(\frac{dy}{dx}\)= \(\frac{d}{dx}\) uv = u \(\frac{d}{dx}\) v + v \(\frac{d}{dx}\) u.
U is the function u(x) v is the function v(x) u' is the derivative of the function u(x) This is the currently selected item. What is integration by parts formula.