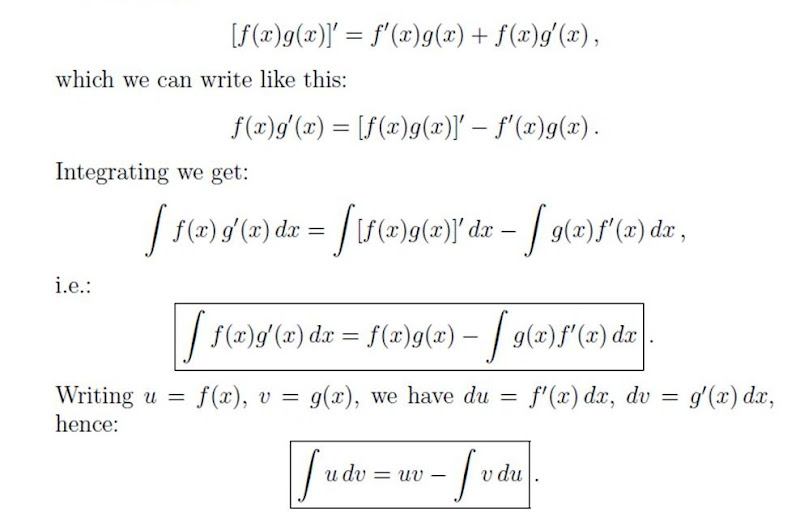Integration by parts is the inverse of the product rule for derivatives; Integration by parts, definite integrals. U is the function u(x)
Answered The integration by parts formula is … bartleby
\[\int {x\cos xdx} ,\;\;\int {{x^2}{e^x}dx} ,\;\;\int {x\ln xdx} ,\] in which the integrand is the product of two functions can be solved using integration by parts.
The course covers integration by table, integ.
Using the integration by parts formula. There is an interesting result which you should remember. Let dv = e x dx then v = e x. This method uses the fact that the differential of function is.
Note that the uv|b a u v | a b in the first term is just the standard integral evaluation notation that you should be familiar with at this point.
Find the integration of xe x by using the integration by parts formula. ∫ u v dx = u ∫ v dx − ∫ u' ( ∫ v dx) dx. Evaluate definite integral by changing limit of integration by parts using rule 2 \int _ { 0 } ^ { 1 } t \sqrt { t + 2 } dt. You will see plenty of examples soon, but first let us see the rule:
∫ 0 6 (2 +5x)e1 3xdx ∫ 6 0 ( 2 + 5 x) e 1 3 x d x solution.
2 [ ( l o g x) ⋅ x − ∫ 1 x ⋅ x d x] = 2 [ x l o g x − x] + c = 2 x ( l o g x − 1) + c. U is the function u (x) This can be proved with the help of integration by parts. For example, the following integrals.
Example 2) find the integration by parts of.
For example, if , then the differential of is. Fudv=uv fvdu use the integration by parts formula for indefinite integrals solution 1. The integration by parts formula in the following video i explain the idea that takes us to the formula, and then i solve one example that is also shown in the text below. Using the integration by parts formula.
Sometimes integration by parts must be repeated to obtain an answer.
Integration by parts is a procedure used to integrate and it is quite beneficial when two functions are multiplied together, but is also helpful in other ways. Integration by parts\(\left({ibp}\right)\) is a special method for integrating products of functions. All that’s left now is to simplify! As we know that, ∫f(x)g(x).dx = f(x)∫g(x).dx − ∫(f′(x)∫g(x).dx).dx + c ⇒ ∫xe x.dx = ∫x.e x.dx.
Let u = t and dv = \sqrt { t + 2 } dt v = \frac { 2 } { 3 } ( t + 2 ) ^ { 3/2 }
∫ l o g x 2 d x. We use integration by parts a second time to evaluate let u = x the du = dx. ∫ b a udv = uv|b a −∫ b a vdu ∫ a b u d v = u v | a b − ∫ a b v d u. We have i = ∫ e x [ f ( x) + f ′ ( x)] d x = ∫ e x f ( x) d x + ∫ e x f ′ ( x) d x.
There are plenty of examples, but first, it is important to clear the rule:
We can integrate by parts on a definite integral, remembering to evaluate limits on each term What is integration by parts formula. Integration by parts is one of the method basically used o find the integral when the integrand is a product of two different kind of function. = i 1 + ∫ e x f ′ ( x) d x, where i 1 = ∫ e x f ( x) d x.
Home→ calculus→ integration of functions→ integration by parts.
Examples of integration by parts. Example 1) find the integration by parts of. The method of integration by parts all of the following problems use the method of integration by parts. ∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of integration by parts:
In order to compute the definite integral $\displaystyle \int_1^e x \ln(x)\,dx$, it is probably easiest to compute the antiderivative $\displaystyle \int x \ln(x)\,dx$ without the limits of itegration (as we computed previously), and then use ftc ii to.
Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. ∫u v dx = u∫v dx −∫u' (∫v dx) dx. Choose u and du use the integration by parts formula for definite integrals u = dv= e did 2. To find the integration of the given expression we use the integration by parts formula:
Now that we have all the variables, let’s plug them into the integration by parts equation:
Illustrative examples using formula of integration by parts. ∫x 2 ln (x)dx=ln (x)⋅ (⅓)x 3 −∫ (⅓)x 3 ⋅ (1/x) dx. Using repeated applications of integration by parts: Further, using ilate rule, ⇒ f(x) = x and g(x) = e x
Solution 1) ∫ l o g x 2 d x = 2 ∫ ( l o g x) ⋅ 1 d x.
2 [ l o g x ⋅ ∫ 1 d x − ∫ ( d d x ( l o g x) ∫ 1 d x) d x] =. Substituting into equation 1, we get. ∫ e x [ f ( x) + f ′ ( x)] d x = e x f ( x) + c. Let dv = e x dx then v = e x.
Integration by parts jan 12 learning outcome example find fxé ' dx • recognize when to use integration by parts * recall:
Of course, we are free to use different letters for variables. The formula for the method of integration by parts is given. This tutorial runs through an integration by parts example.this video is part of a full free calculus 2 course. For example, if , then the differential of is.
( 2 − 3 x) d x solution.