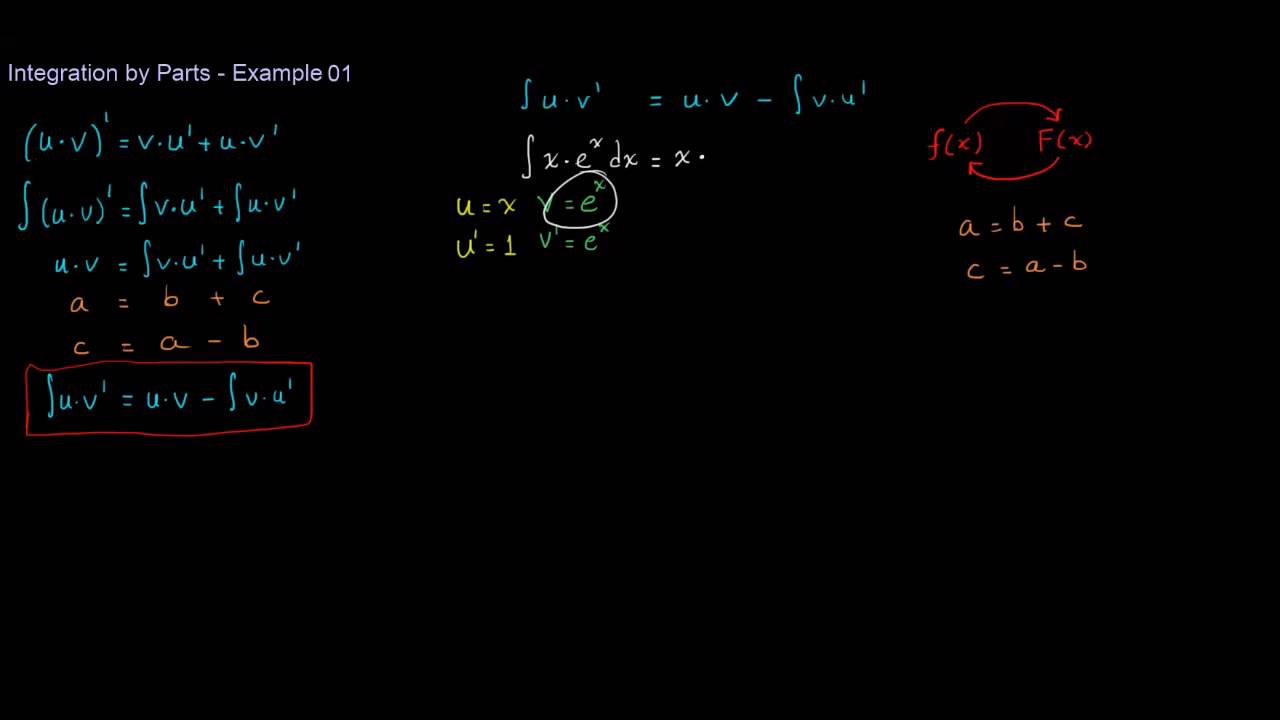We have already seen the reverse chain rule. (fg)' = f'g + fg'. Substitute this with u, and let dv / dx be the other multiple.
Deriving the Integration by Parts formula YouTube
This video aims to show you and then works through an example.
³ ³ ³x e dx fg g fdx x e n x e dxn x n x n x ' 1.
Same deal with this short form notation for integration by parts. There are two more methods that we can use to perform the integration apart from the integration by parts formula,. Many rules and formulas are used to get integration of some functions. For some questions, you need to integrate by parts more than once to get a result.
To use the integration by parts formula we let one of the terms be dv dx and the other be u.
Note that the uv|b a u v | a b in the first term is just the standard integral evaluation notation that you should be familiar with at this point. Choose u and v’, find u’ and v. So the integration by parts formula can be written as: Integration by parts is the reverse product rule.
This topic will derive and illustrate this rule which is integration by parts formula.
Applying integration by parts twice over: ∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application. Now that we have all the variables, let’s plug them into the integration by parts equation: And from that, we're going to derive the formula for integration by parts, which could really be viewed as the inverse product rule, integration by parts.
[x2 f(x) type] worked example
³³x e dx x e n x e dxn x n x n x 1. The integral above is defined for positive integer values n. We can also sometimes use integration by parts when we want to integrate a function that cannot be split into the product of two things. Most questions that require integration by parts will have x or x2 as one of the multiples.
A special rule, which is integration by parts, is available for integrating the products of two functions.
Using the formula for integration by parts example find z x cosxdx. ∫ u v dx = u ∫ v dx − ∫ u' (∫ v dx) dx. This article talks about the development of integration by parts: Putting these into the formula we get:
The trick we use in such circumstances is to multiply by 1 and take du/dx = 1.
Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. All that’s left now is to simplify! ∫ u v d x = u d x − ∫ ( d u d x ∫ v d x) d x. Notice from the formula that whichever term we let equal u we need to differentiate it in order to.
By using this website, you agree to our cookie policy.
This one a bit deeper: Sometimes integration by parts must be repeated to obtain an answer. Using repeated applications of integration by parts: D/dx [f (x)·g (x)] = f' (x)·g (x) + f (x)·g' (x) becomes.
Integration by parts, definite integrals.
This yields the formula for integration by parts: Sin sin 2 2cos 1 sin. The method of integration by substitution. So let's say that i start with some function that can be expressed as the product f of x, can be expressed as a product of two other functions, f of x times g of x.
Integration by parts has many uses, most notably integrating things of the form x^{n}f(x).
The method of integration using partial fractions. It is important that you can recognise what types of integrals require the method of integration by parts. The original integral, but reduces it to a formula that involves the same integral, but with a lower power of x. If an indefinite integral remember “ +c ”, the constant.
By using the identity sin2 =1 cos2 x,onecanexpresssinm x cosn x as a sum of constant multiples of powers of cosx if m is even.
∫ b a udv = uv|b a −∫ b a vdu ∫ a b u d v = u v | a b − ∫ a b v d u. One can use integration by parts to derive a reduction formula for integrals of powers of cosine: A)use trigonometric identities to show that. 1)view solution 2)view solution 3)view solution 4)view solutionpart (a):
U is the function u(x) v is the function v(x) u' is the derivative of the function u(x)
∫x 2 ln (x)dx=ln (x)⋅ (⅓)x 3 −∫ (⅓)x 3 ⋅ (1/x) dx. To calculate the integration by parts, take f as the first function and g as the second function, then this formula may be pronounced as: Z cos n xdx= 1 n cos n1 x sin x + n 1 n z cos n2 xdx. One can integrate all positive integer powers of cos x.
F e g x f e g nx' , , , 'x n x n.
Integration is a very important computation of calculus mathematics. Solution here, we are trying to integrate the product of the functions x and cosx. You will see plenty of examples soon, but first let us see the rule: We can therefore conclude that for any value of n: