Let x be exponentially distributed with mean 2, and let y = (x if x ≤ 5, 5 if x > 5. Integration by parts jan 12 learning outcome example find fxé ' dx • recognize when to use integration by parts * recall: Let u = x2, then du/dx = 2x or du = 2xdx.
Integration MATH100 Revision Exercises Resources
Integrating by parts, we get e(y) = z ∞ 1 (x−1) 1 2 e−x/2dx = −(x−1)e−x/2 ∞ 1 + z ∞ 1 e−x/2dx = 0−2e−x/2 ∞ 1 = 2e−1/2.
Z µ 1 2x − 2 x2 + 3 √ x ¶ dx = 1 2 z 1.
If it wasn't there, we could make it integrated. Substitute these expressions in 7.18. Z sin10(x)cos(x) dx (a)let u= sin(x) dx (b)then du= cos(x) dx (c)now substitute z sin10(x)cos(x) dx = z u10 du = 1 11 u11 +c = 1 11 sin11(x)+c 7. Here is a set of practice problems to accompany the integration by parts section of the applications of integrals chapter of the notes for paul dawkins calculus ii course at lamar university.
Note that 1dx can be considered a function.
1 simple rules so, remember that integration is the inverse operation to di erentation. In problems 1 through 7, find the indicated integral. ∫ udv= uv −∫ vdu example 1: \[\int{{x{{{{bf{e}}^{6x}}\,dx}}\] view solution so, at any level, the problem here is the \(x\) that is in front of the exponential.
For some of you who want more practice, it™s a good pool of problems.
Integration by parts 21 1.5. Then z exsinxdx= exsinx excosx z. E(y) = z 5 0 x 1 2 e−x/2dx+ z ∞ 5 5· 1 2 e−x/2dx = −xe−x/2 5 0 + z 5 0 e−x/2dx+ 5 2 z ∞ 5 e−x/2dx Example 7.5 find xe xdx.
Then use the formula z u dv dx dx = uv − z v du dx dx :
Z x cosxdx = x sinx− z (sinx)·1dx = xsinx +cosx+c where c is the constant of integration. The integrand must contain two separate functions. Z sin 1(x) dx 2. Example 6 find a reduction formula for secnx dx.
Integrate the new differential vdu.
Example find z x2e3x dx. By letting u = f (x)⇒ du = f!(x)dx dv = g(x)dx ⇒v = g(x) we get the more common formula for integration by parts: We will integrate this by parts, using the formula z f0g = fg z fg0 let g(x) = x and f0 (x) = ex then we obtain g0 and f by di⁄erentiation and integration. Note and that in making the integration of parts something that we choose for \(u.
F (x) = ex g(x) = x f0 (x) = ex g0 (x) = 1
We don't choose dv = sec x dx Write down the expressions for u dv and du v. Let u= sinx, dv= exdx. Substitute u — 3— i)16dx— 8x2 (3x —u17 + c = 17 —(3x3 l) 17 + c i 153 the definite integral in example i (b) can be evaluated more simply by carrying over the cx2.
Let u x dv exdx
This allows us to write: For example, consider again this simple problem: This is not the only way to do the algebra, and typically there are many paths to the Z ln(x) x2 dx 5.
(at this stage do not concern yourself with the constant of integration).
U =sin x (trig function) (making “same” choices for u and dv) dv =ex dx (exponential function) du =cosx dx v =∫ex dx =ex 7 practice problems concerning integration by parts 1. Up to 24% cash back integration by parts pdf problems. Z tan 1(x) dx 3.
Let u= cosx, dv= exdx.
As x varies from o to a, so u varies from() limits of integration. Then du= sinxdxand v= ex. Z f(x)g(x)dx = f(x)g(x)− z f(x) dg dx dx where df dx = f(x) of course, this is simply different notation for the same rule. Up to 24% cash back this formula for integration by parts often makes it possible to reduce a complicated integral involving a product to a simpler integral.
Helps to avoid silly mistakes.
In the next example we will see that it is sometimes necessary to apply the formula for integration by parts more than once. We substituted u = — to —ca2. Then z exsinxdx= exsinx z excosxdx now we need to use integration by parts on the second integral. Let u=lnx and dx x dv xdx du 1 = ⇒ = and 2 2 1 v = ∫ xdx = x.
For example, ∫x(cos x)dx contains the two functions of cos x and x.
Evaluate inde nite integrals using integration by parts: Choose u and du use the integration by parts formula for definite integrals u = dv= e did 2. Z u dv dx dx = uv − z du dx vdx but you may also see other forms of the formula, such as: Example 2 example 3 evaluate 8x (3x solution:
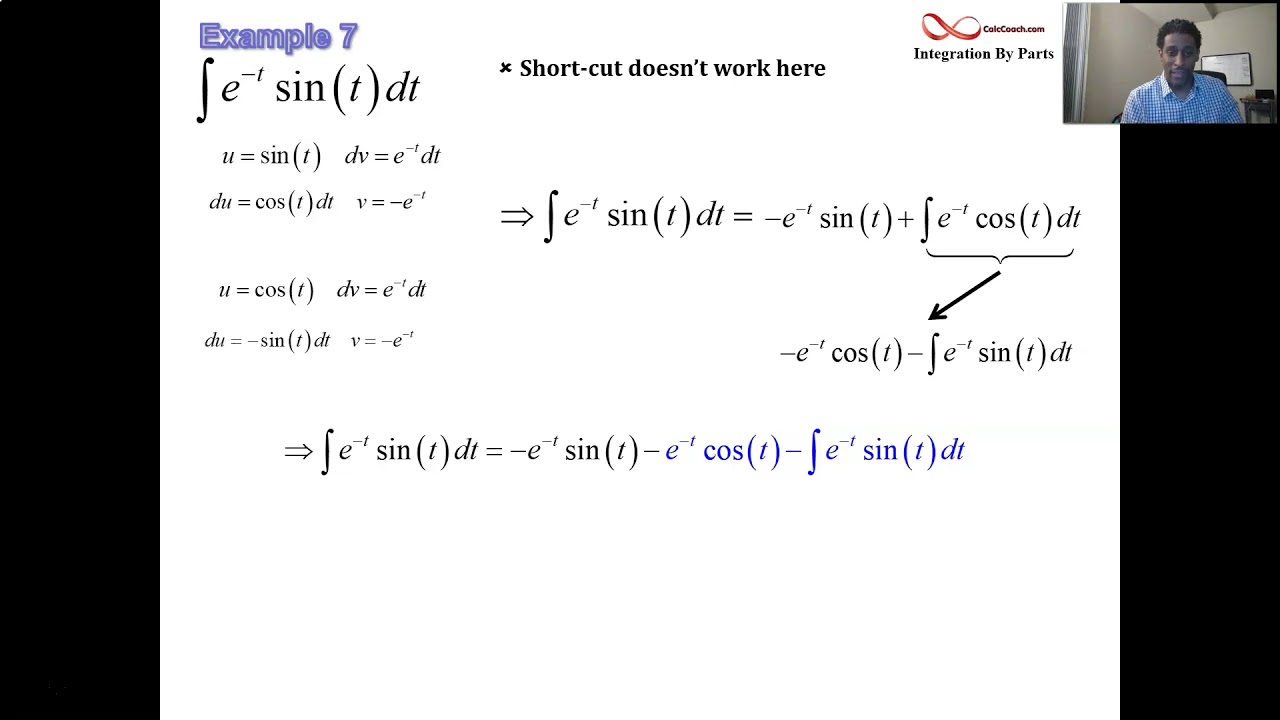
In the following example the formula of integration by parts does not yield a final answer, but an equation verified by the integral from which its value can be derived.
Solution the idea is that n is a (large) positive integer, and that we want to express the given integral in terms of a lower power of sec x. Z 3e xdx =3 exdx =3e +c. ∫ex cosx dx u =cos x (trig function) dv =ex dx (exponential function) du =−sin x dx v =∫ex dx =ex ∫ex cosx dx =uv−∫vdu =cosx ex −∫ex (−sin x) dx =cosx ex +∫ex sin x dx second application of integration by parts: Many exam problems come with a special twist.
Example 1 evaluate the following integral.
Z √ xdx = z x1 2 dx = 2 3 x3 2 +c = 2 3 x √ x+c. R ³ 1 2x −2 x2 + √3 x ´ dx solution. Apply three times, start with g(x) = x3 8. Integration by parts is a technique used to solve integrals that fit the form:
To see this, make the identifications:
First identify the parts by reading the differential to be integrated as the product of a function u easily differentiated, and a differential dv easily integrated. Z x2 sin(x) dx 6. Cosn xdx by reducing the problem to nding r cosn 2 xdx using the following reduction formula: Since we have exactly 2xdx in the original integral, we can replace it by du:
Sum/di erence r (f(x) g(x)) dx = r f(x)dx r g(x) dx scalar multiplication r cf(x.
Fudv=uv fvdu use the integration by parts formula for indefinite integrals solution 1. The following are solutions to the integration by parts practice problems posted november 9. Z 2xcos(x2)dx = z cosudu = sinu+c = sin(x2)+ c. Apply twice, start with g(x) = (ln(x))2 10.
Integration by parts the method of integration by parts is based on the product rule for.
Apply twice, start with g(x) = x2 3. Z ex cos(x) dx 5 challenge problems concerning integration by parts Thuse we get a few rules for free: The solutions are not proven
U = cosn 1 x dv = cosxdx du = (n 1)cosn 2 xsinx v = sinx z cos nx = z cos 1 cosxdx = cosn 1 xsinx (n 1) z sin2 xcosn 2 xdx = cosn 1 xsinx+ (n 1) z (1 cos2 x)cosn 2 xdx = cosn 1 xsinx+ (n 1) z
R (3x2 − √ 5x+2)dx solution. Apply twice, start with g(x) = x2 +1 6. In this tutorial, we express the rule for integration by parts using the formula: The easiest power of sec x to integrate is sec2x, so we proceed as follows.
∫u dv this method is to be used when normal integration and substitution do not work.
Evaluate the following inde nite integrals (or \ nd the following. Z cosn x = 1 n [cosn 1 xsinx+ (n 1) z cosn 2 xdx] we prove this using integration by parts: Then du= cosxdxand v= ex. Z (3x2 − √ 5x+2)dx =3 z x2dx− √ 5 z √ xdx+2 z dx = =3· 1 3 x3 − √ 5· 2 3 x √ x+2x+c = = x3 − 2 3 x √ 5x+2x+c.
Practice problems on integration by parts (with solutions) this problem set is generated by di.
Methods of integration william gunther june 15, 2011 in this we will go over some of the techniques of integration, and when to apply them. I pick the representive ones out.