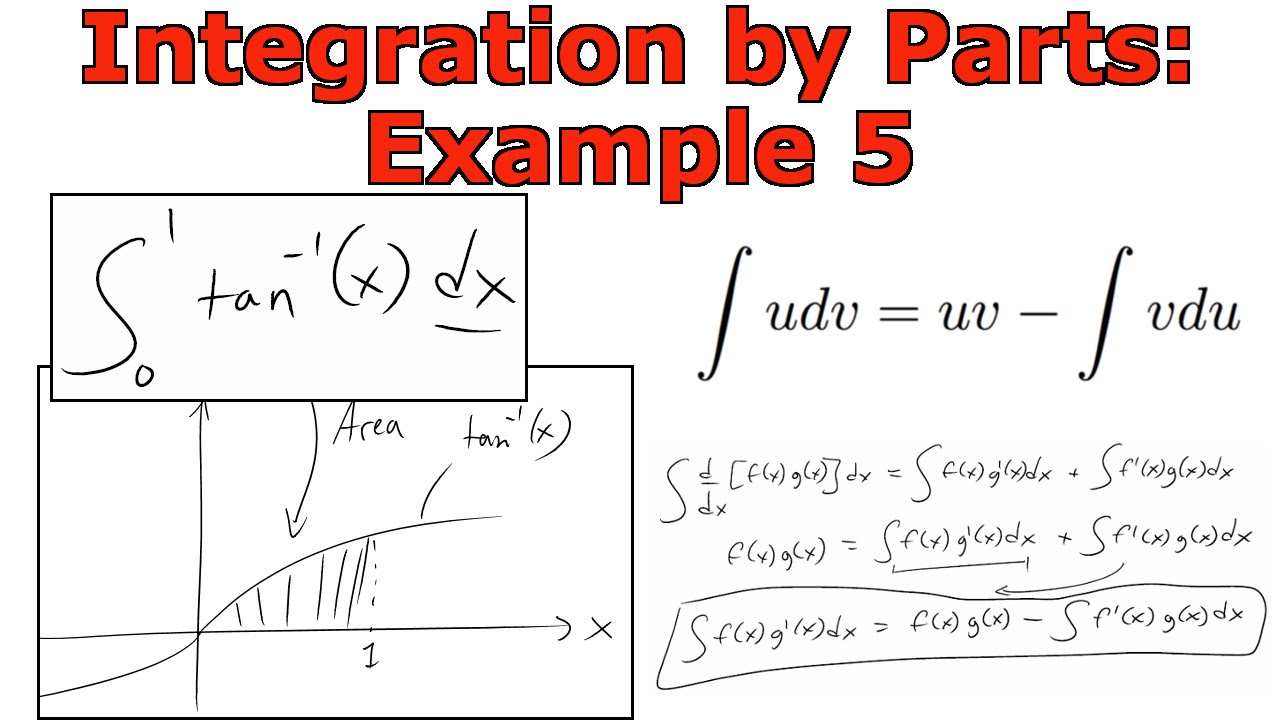
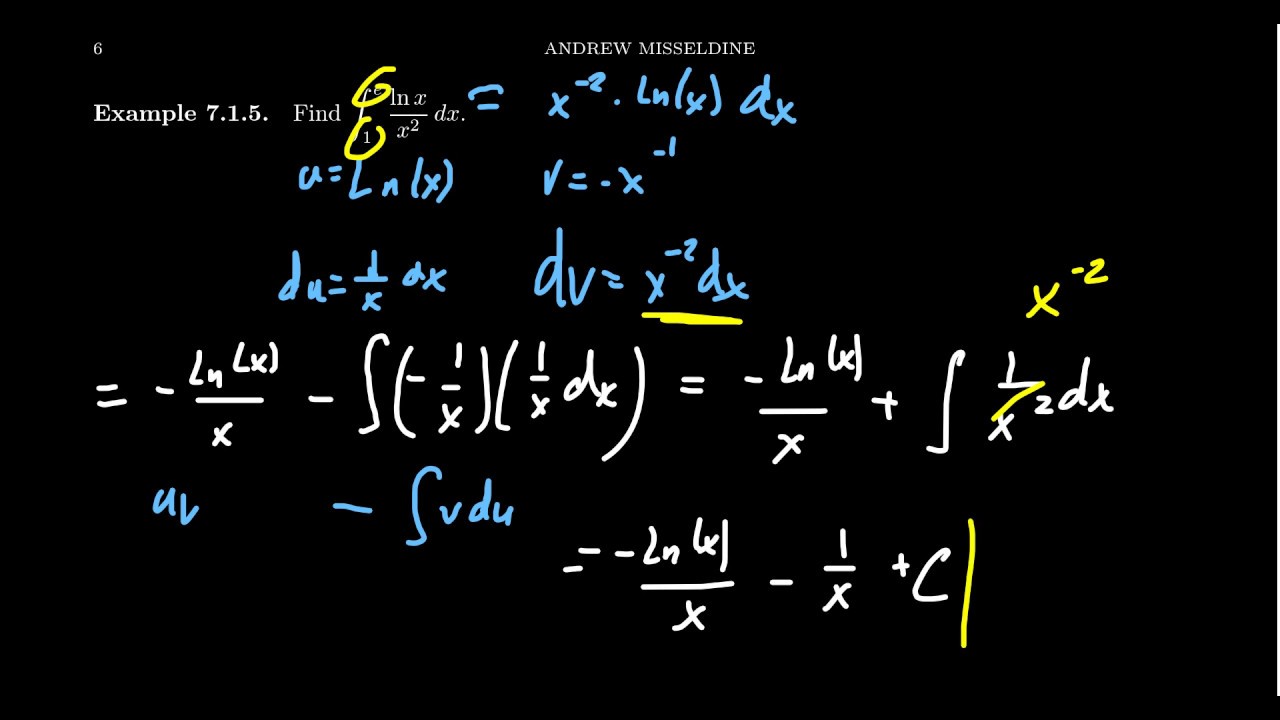First solve it, according to this: Learn how to derive the integration by parts formula in integral calculus mathematically from the concepts of differential calculus in mathematics. D/dx [f (x)·g (x)] = f' (x)·g (x) + f (x)·g' (x) becomes.
core pure 3 notes
With a definite integral, that becomes.
It's easier to see how to take the parts.
Use the method of integration by parts to evaluate the definite integral {eq}\int_0^4 xe^x\ dx {/eq}. In order to compute the definite integral ∫ 1 e x ln. Again, u = x and dv/dx = cos x. The definite integral has a unique value and is denoted by;
Definite integration by parts formula is generally used to integrate the product of two functions.
Evaluate the definite integral using integration by parts with way 2. P’ is the derivative of function p(x). ( x sin x) | a b = b sin b − a sin a. If we rewrite the integrand as.
The definite integration by parts formula is given as :
The purpose of this problem is to show that if this condition is satisfied and if f is continuous, then a definite integral of f − 1 can be expressed in terms of a definite integral of f. Definite integration by parts formula. Therefore, we have to apply the formula of integration by parts. V' = 2 xe x 2.
(a) use integration by parts to show that b a f(x) dx = bf(b) − af(a) − b a xf (x) dx (b) use the result in part (a) to show that if y = f(x), then b a f(x) dx = bf(b) − af(a) − f(b) f(a) f − 1 (y) dy (c) show that if.
( x) d x, it is probably easiest to compute the antiderivative ∫ x ln. The integration by step calculator will provide the most accurate results of integration or integrals either of definite or indefinite. Integration by parts, definite integrals. Khan academy is a 501(c)(3) nonprofit organization.
Definite integration by parts formula.
Now, since dv/dx = cos x Are the two formulas equivalent or not? Integration by parts with a definite integral. ∫ b a udv = uv|b a −∫ b a vdu ∫ a b u d v = u v | a b − ∫ a b v d u.
∫ x cos x d x = x sin x − ∫ sin x d x.
Can we write the integration by parts for definite integral the following way: For solving the above definite integral problem with integration by parts using rule 1, we have to apply limits after the end of our result. ∫ a b x cos x d x = ( x sin x) | a b − ∫ a b sin x d x. X − 1 4 x 2 + c.
We already found the value, du/dx = 1.
Here, let x is equal to u, so that after differentiation, du/dx = 1, the value we get is a constant value. Previously, we found ∫ x ln. The following example illustrates its. This article talks about the development of integration by parts:
Using the integration by parts formula, identify the functions to be used for {eq}u.
P is the function p(x), q is the function q (x), and. Same deal with this short form notation for integration by parts. ∫ b a f ( x) g ( x) d x = [ f ( x) ∫ g ( x) d x] b a − ∫ b a [ d f ( x) d x ∫ g ( x) d x] d x. Definite integration by parts formula.
As per the formula, we have to consider, dv/dx as one function and u as another function.
P represents the function p(x) Note that the uv|b a u v | a b in the first term is just the standard integral evaluation notation that you should be familiar with at this point. Explanation of definite integration by parts formula is as follows: (1 week ago) math ap®︎/college calculus bc integration and accumulation of change using integration by parts.
We need to use the factor trick on this one.
The mathematical formula for the integration by parts can be derived in integral calculus by the concepts of differential calculus. ∫ b a f ( x) g ( x) d x = f ( x) ∫ b a g ( x) d x − ∫ b a [ d f ( x) d x ∫ b a g ( x) d x] d x. Definite integration by parts formula is generally used to integrate the product of two functions. (fg)' = f'g + fg'.
\(\int_b^af\left(x\right)dx \), where b is the lower limit and a is the upper limit of the integral
( x) d x = x ln. This online tool for integration by parts will help in managing your time from the manual calculation and also increases the chances of learning the integration by part more efficiently. Thanks to all of you who support me on patreon. My book gives the following formula for definite integral integration by parts:
In the above definite integration by parts formula.
For example, the indefinite integral ∫ x cos x d x can be found with the help of integration by parts to be. This one a bit deeper: The definite integration by parts formula is given as :