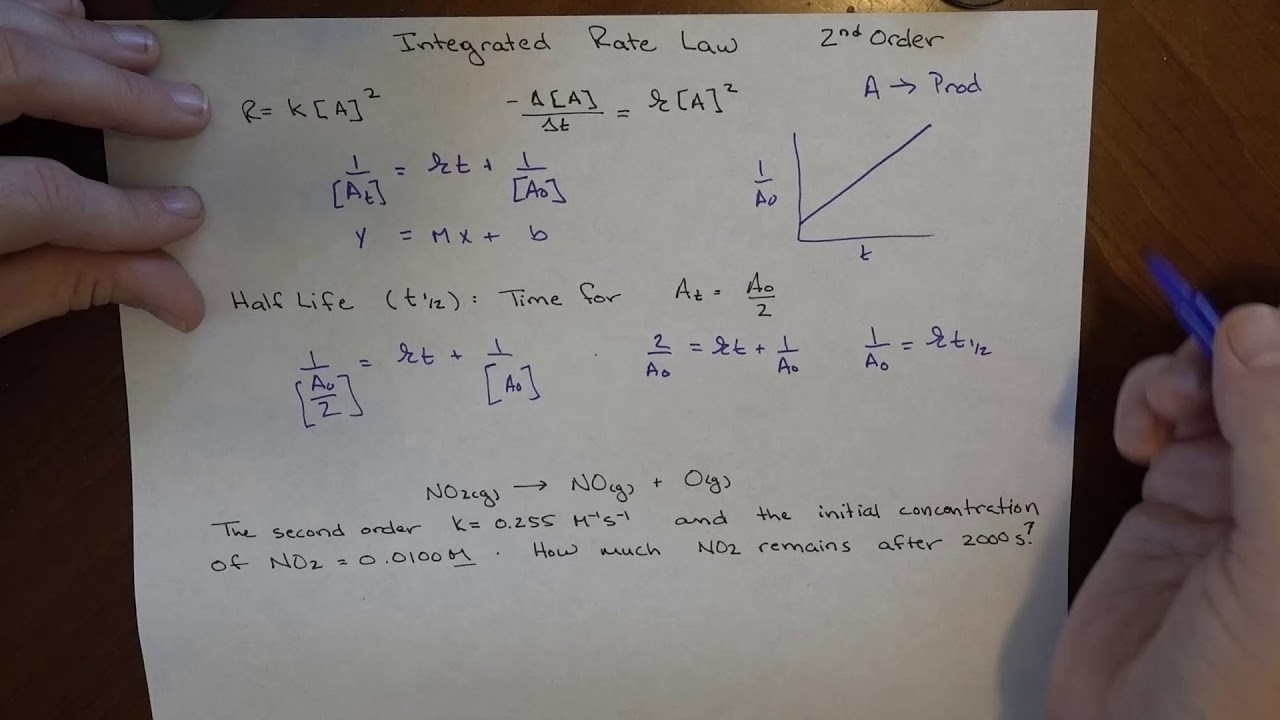
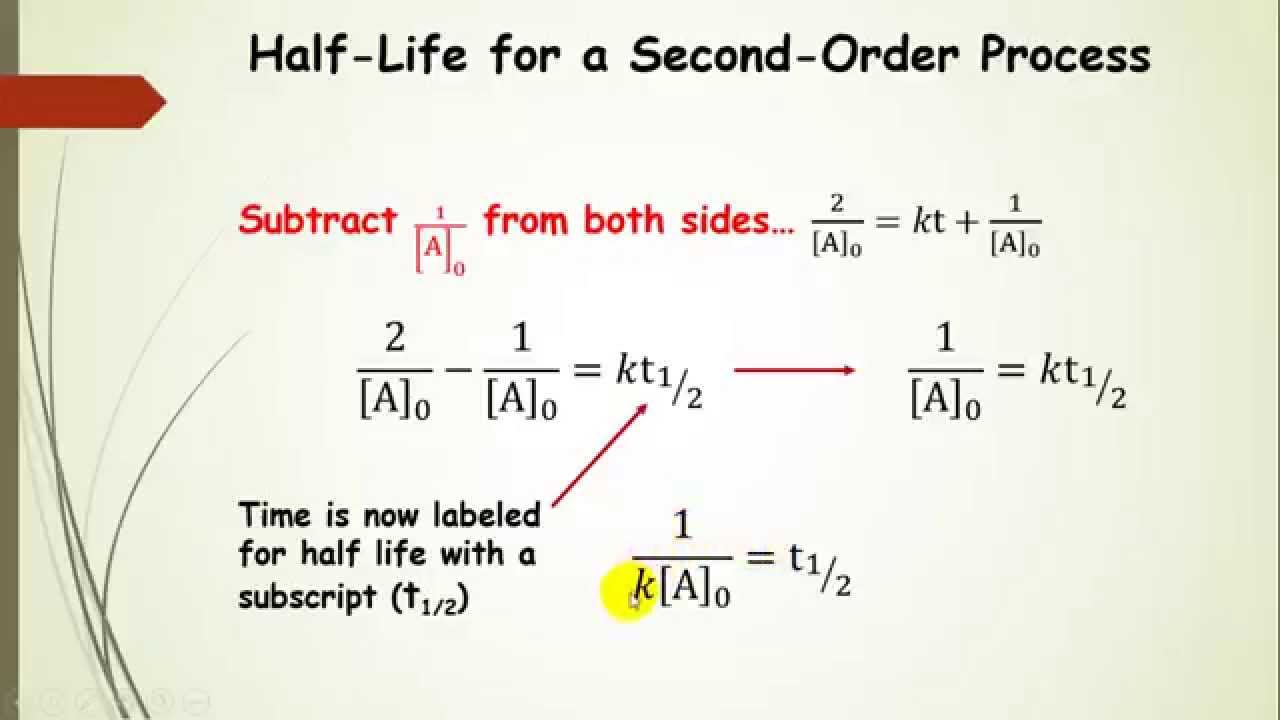
When a reaction is of second order with regard to a specific reactant, an increase in its quantity causes the rate to grow. The equation for the second order integrated rate law takes the form y = mx +b, where y = 1/a; Putting the limits in equation (1) we get the value of c, ⇒ [ a] 0 = c.
Derive the integrated rate law of the second order
−d[r] /dt = k[r] 2;
The integrated rate law equation shows a linear relationship between the reciprocal of the reactants concentration and time.
These are inherently differential equations, because the rate is always defined as a change in concentration with time; [] 2 0 1 x = 1 t k 4 note: The rate constant for the reaction can be determined from the slope of the line, which is equal to k. \[\dfrac{1}{[a]}=kt+\dfrac{1}{[a]_0} \label{int2nd}\] where the terms in the equation have their usual meanings as defined earlier.
[latex]\begin{array}{ccc}\hfill \frac{1}{\left[a\right]}& =& kt+\frac{1}{{\left[a\right]}_{0}}\hfill \\ \hfill y& =& mx+b\hfill \end{array}[/latex]
(i) where i is the constant if integration. [] [] 0 1 1 x x = + kt • straight line: If the plot is not a straight line, then the reaction is not second order. (1) where, c= constant of integration, at time, t=0, [a] = [ a] 0.
Therefore, the rate law of this reaction is, rate [r] 0.
Thus, the graph of the second order integrated rate law is a straight. The rate constant for a certain second order reaction is 8 × 10 −5m −1min −1 how long will it take at 1 m sollution to be reduced to 0.5m in reactant ? Because this equation has the form y = mx + b, a plot of the inverse of [a] as a function of time yields a straight line. The integral form of the equation was obtained from the differential form and the full integration can be found here.
1 [ a] t = k t + 1 [ a] 0 y = m x + b.
2a products or a + b products (when [a] = [b]) , rate = k[a] 2 the integrated rate law is 1/[a] = kt + 1/[a o ] Second order & pseudo first order reaction. 1 [ a] t = k t + 1 [ a] 0 y = m x + b. At t = 0, the concentration of the reactant r = [r] 0.
